Mathematics
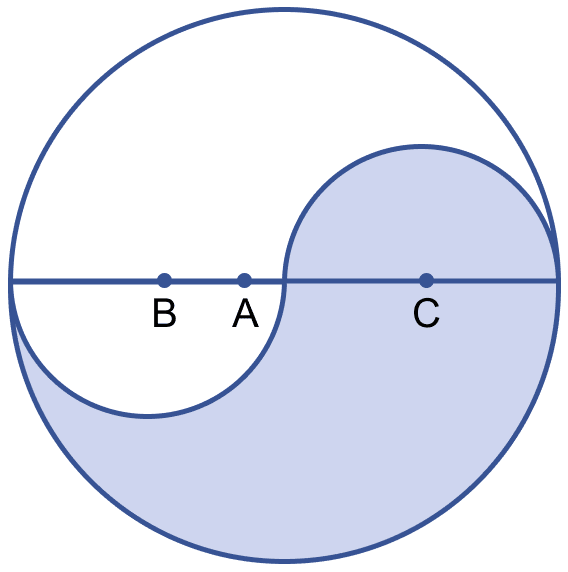
In the figure (i) given below, the points A, B and C are centres of arcs of circles of radii 5 cm, 3 cm and 2 cm respectively. Find the perimeter and the area of the shaded region. (Take π = 3.14)
Mensuration
30 Likes
Answer
Let r1 = 5 cm, r2 = 3 cm and r3 = 2 cm.
Perimeter of shaded region = Circumference of largest semi-circle + Circumference of smaller semi-circle + Circumference of smallest semi-circle
= πr1 + πr2 + πr3
= π(5 + 3 + 2)
= 10π
= 10 x 3.14
= 31.4 cm.
Area of shaded region = Area of largest semi-circle - Area of smaller semi-circle + Area of smallest semi-circle
Hence, perimeter of shaded region = 31.4 cm and area of shaded region = 31.4 cm2.
Answered By
18 Likes
Related Questions
In the figure (i) given below, ABCD is a square of side 14 cm. A, B, C and D are centres of the equal circles which touch externally in pairs. Find the area of the shaded region.

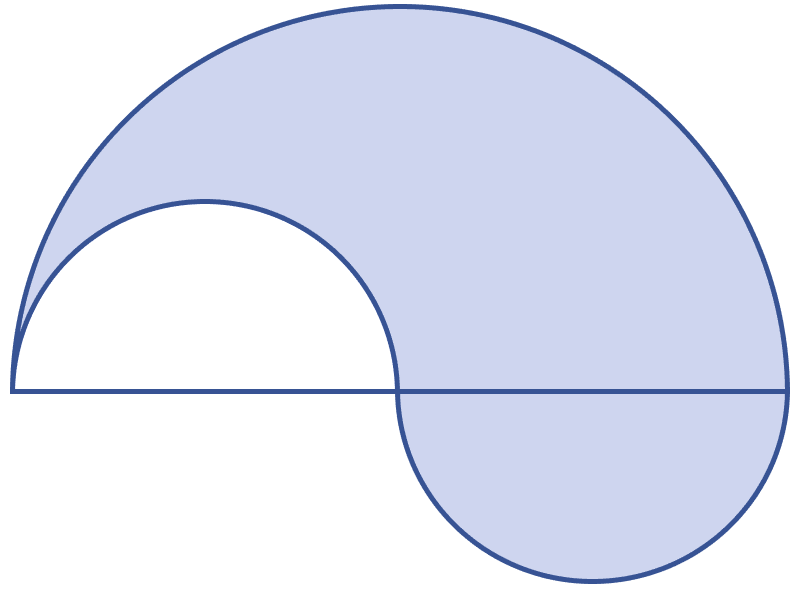
In the figure (ii) given below, the boundary of the shaded region in the given diagram consists of three semicircular arcs, the smaller being equal. If the diameter of the larger one is 10 cm, calculate.
(i) the length of the boundary.
(ii) the area of the shaded region. (Take π to be 3.14)
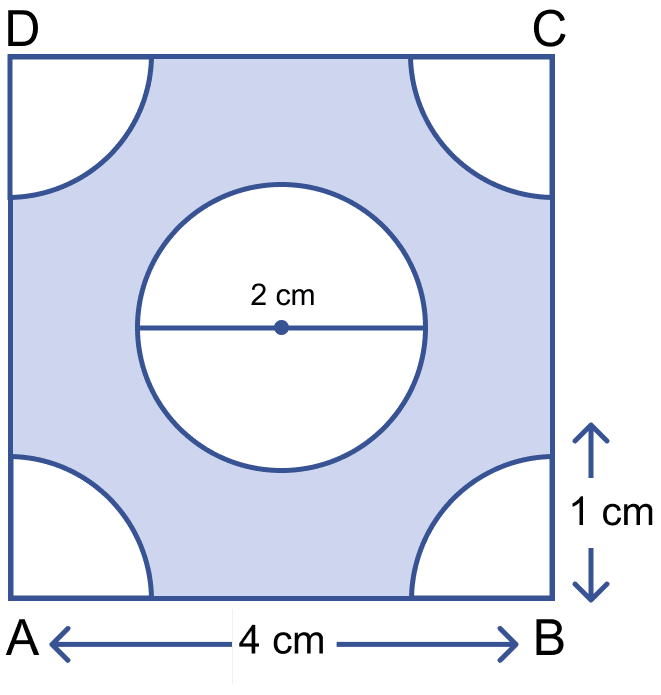
In the figure (ii) given below, ABCD is a square of side 4 cm. At each corner of the square a quarter circle of radius 1 cm, and at the centre a circle of diameter 2 cm are drawn. Find the area of the shaded region. Take π = 3.14.
In the figure (i) given below, ABCD is a rectangle. AB = 14 cm, BC = 7 cm. From the rectangle, a quarter circle BFEC and a semicircle DGE are removed. Calculate the area of the remaining piece of the rectangle.
