Mathematics
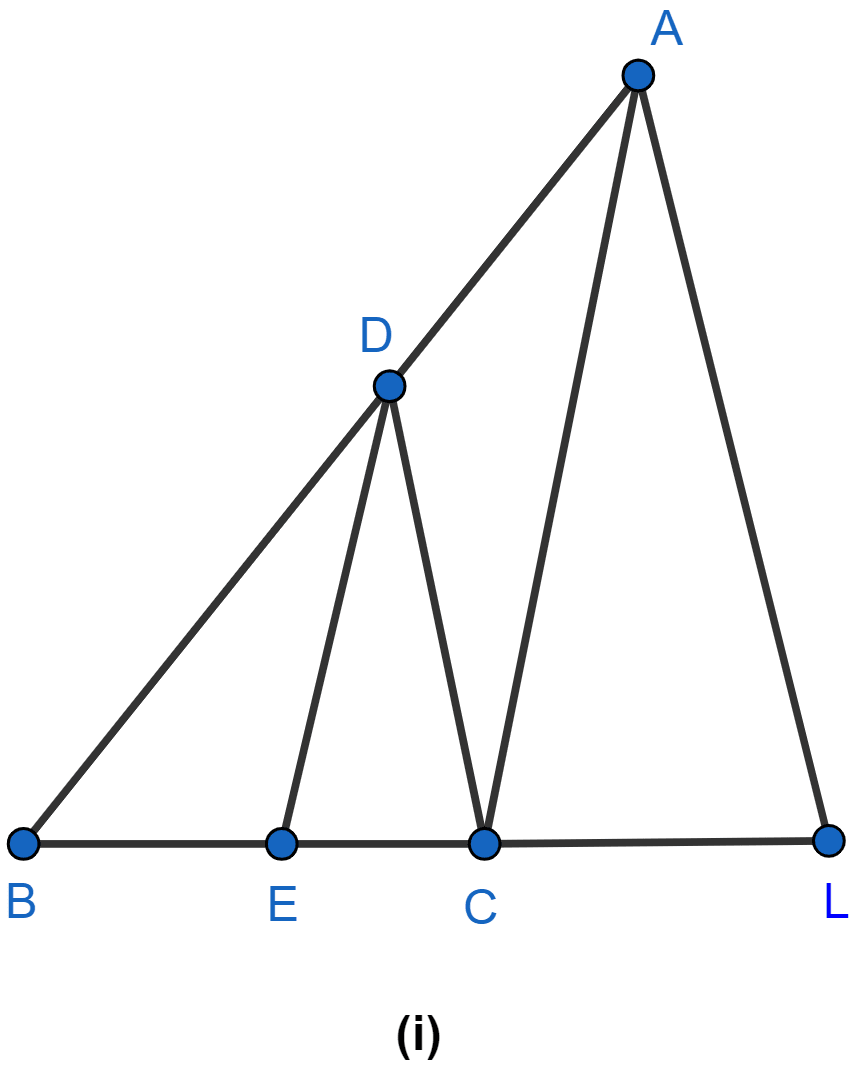
In the figure (i) given below, CD || LA and DE || AC. Find the length of CL if BE = 4 cm and EC = 2 cm.
Similarity
90 Likes
Answer
Considering △ABC and △BDE,
∠B = ∠B (Common angle)
∠DEB = ∠ACB (Corresponding angles)
So, by AA rule of similarity △ABC ~ △BDE. Since ratio of corresponding sides is same,
Considering △ABL and △BDC,
∠B = ∠B (Common angle)
∠BDC = ∠BAL (Corresponding angles)
So, by AA rule of similarity △ABL ~ △BDC. Since ratio of corresponding sides is same,
Solving Eq 1 and Eq 2 we get,
Hence, the length of CL is 3 cm.
Answered By
48 Likes
Related Questions
E and F are points on the sides PQ and PR respectively of a △PQR. For each of the following cases, state whether EF || QR.
(i) PE = 3.9 cm, EQ = 3 cm, PF = 8cm and RF = 9 cm.
(ii) PQ = 1.28 cm, PR = 2.56 cm, PE = 0.18 cm and PF = 0.36 cm.
A and B are respectively the points on the sides PQ and PR of a triangle PQR such that PQ = 12.5 cm, PA = 5cm, BR = 6 cm and PB = 4 cm. Is AB || QR ? Give reasons for your answer.
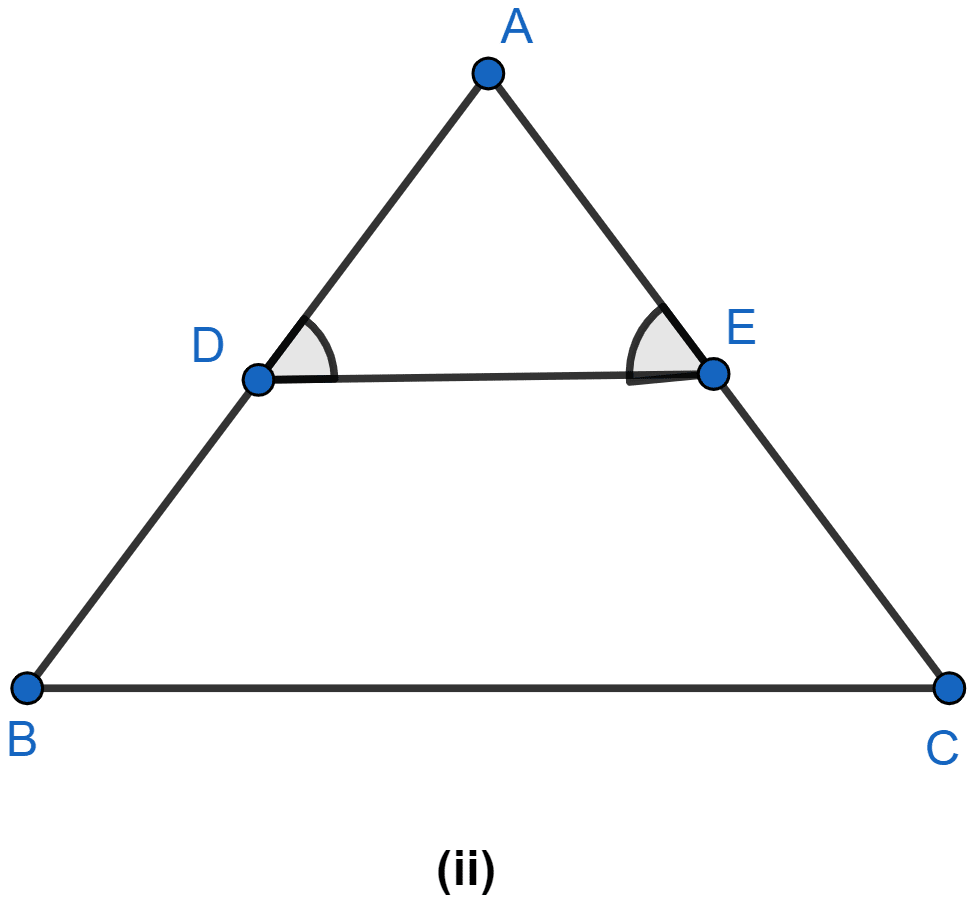
In the figure (ii) given below, ∠D = ∠E and . Prove that ABC is an isosceles triangle.
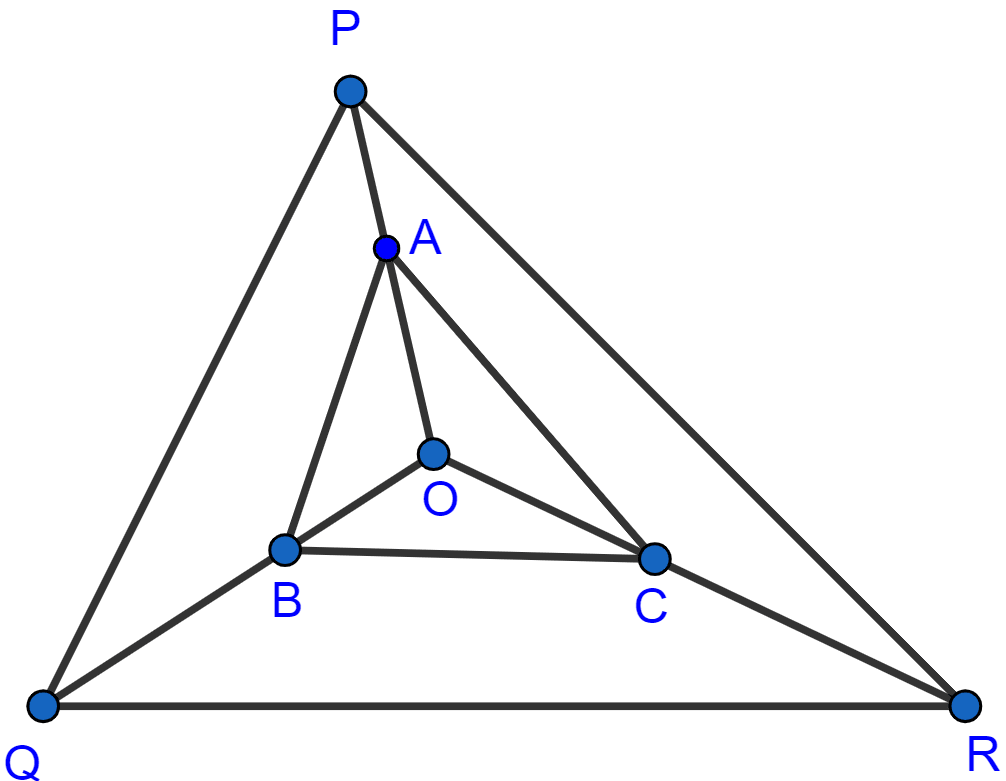
In the adjoining figure, A, B and C are points on OP, OQ and OR respectively such that AB || PQ and AC || PR. Show that BC || QR.