Mathematics
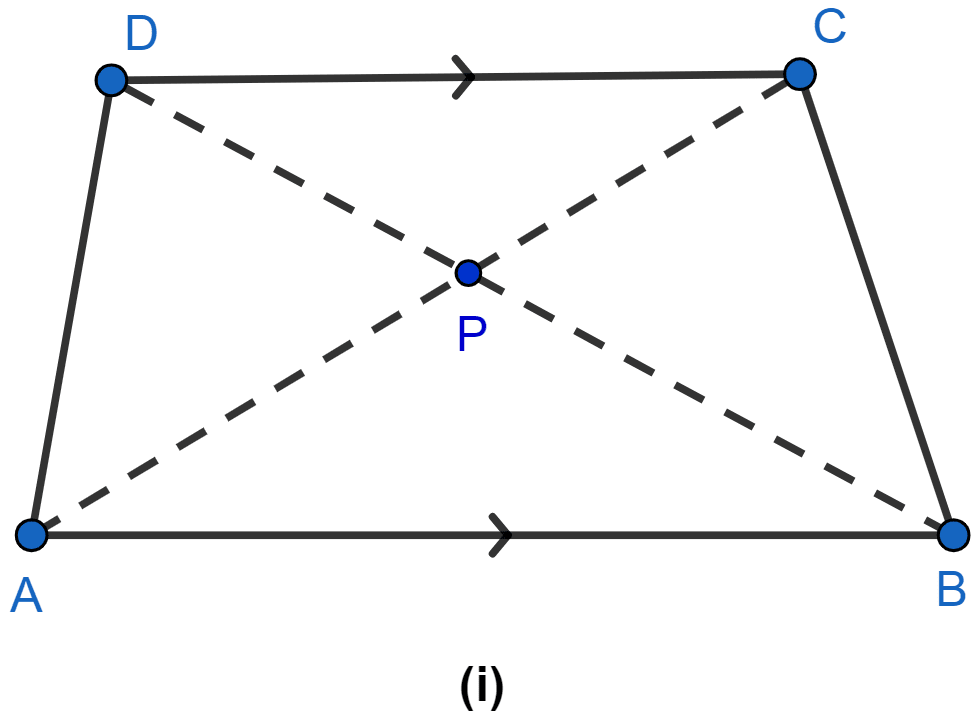
In the figure (i) given below, ABCD is a trapezium in which DC is parallel to AB. If AB = 9 cm, DC = 6 cm and BD = 12 cm, find
(i) BP
(ii) the ratio of areas of △APB and △DPC.
Similarity
32 Likes
Answer
(i) Considering △APB and △CPD.
∠APB = ∠CPD (Vertical opposite angles are equal)
∠PAB = ∠PCD (Alternate angles are equal)
Hence, by AA axiom △APB ~ △CPD.
Since triangles are similar, hence the ratio of the corresponding sides will be equal
Hence, the length of BP = 7.2 cm
(ii) We know that, the ratio of the areas of two similar triangles is equal to the ratio of the square of their corresponding sides.
Hence, area of △APB : area of △DPC = 9 : 4.
Answered By
19 Likes
Related Questions
In the figure (i) given below, DE || BC and the ratio of the areas of △ADE and trapezium DBCE is 4 : 5. Find the ratio of DE : BC.

In the figure (ii) given below, AB || DC and AB = 2DC. If AD = 3 cm, BC = 4 cm and AD, BC produced meet at E, find
(i) ED
(ii) BE
(iii) area of △EDC : area of trapezium ABCD.

In the figure (ii) given below, ∠ABC = ∠DAC and AB = 8 cm, AC = 4 cm, AD = 5 cm.
(i) Prove that △ACD is similar to △BCA.
(ii) Find BC and CD.
(iii) Find area of △ACD : area of △ABC.

In the given figure,
∠ PQR = ∠ PST = 90°, PQ = 5 cm and PS = 2 cm.(i) Prove that △PQR ~ △PST.
(ii) Find area of △PQR : area of quadrilateral SRQT.
