Mathematics
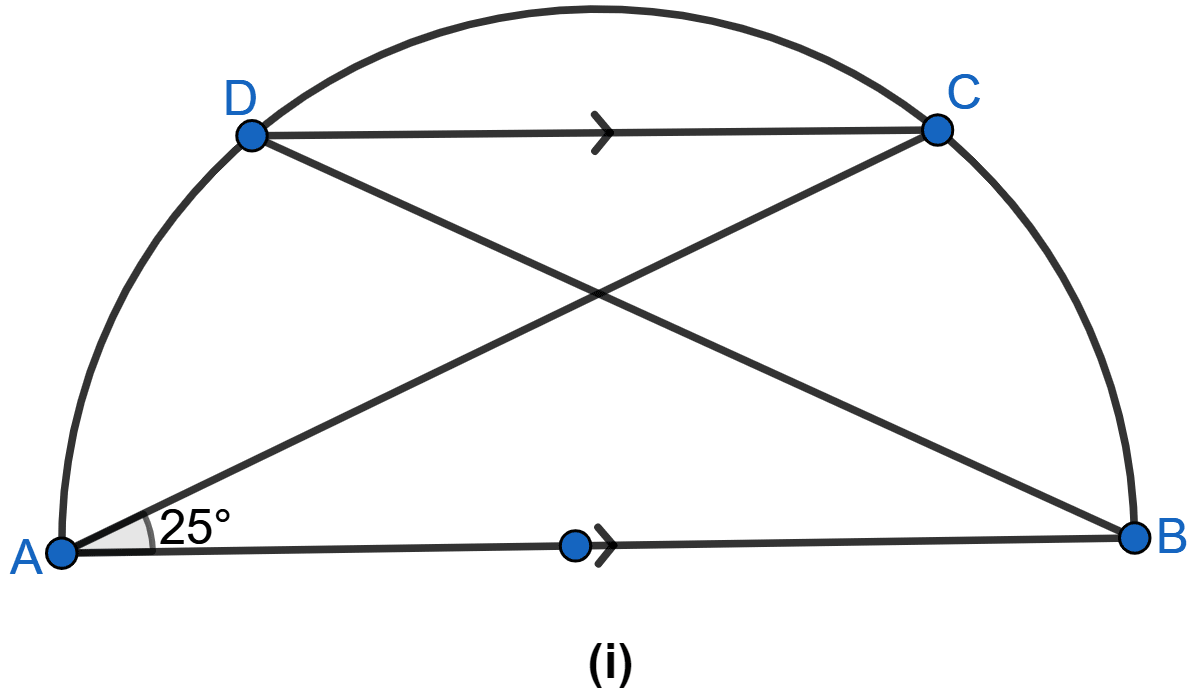
In the figure (i) given below, AB is diameter of a circle. If DC is parallel to AB and ∠CAB = 25°, find (i) ∠ADC (ii) ∠DAC.
Circles
8 Likes
Answer
(i) Join AD.
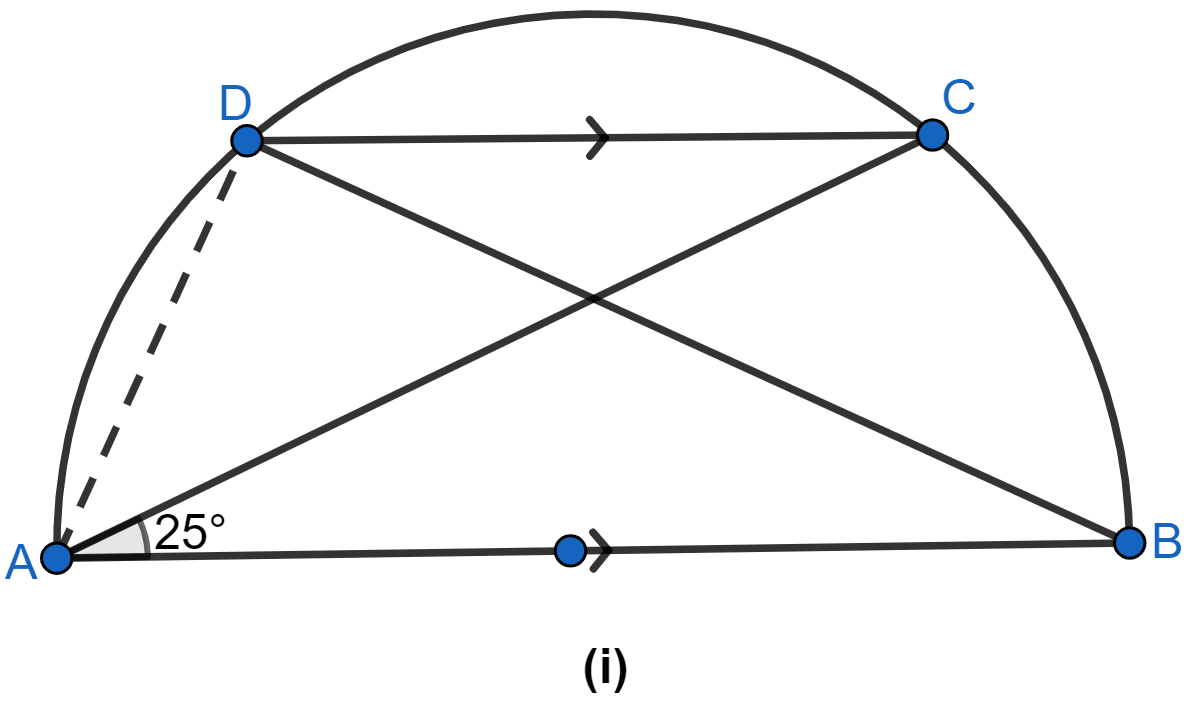
From figure,
∠BDC = ∠BAC = 25°. (∵ angles in same segment are equal.)
∠ADB = 90° (∵ angle in semicircle = 90°.)
∠ADC = ∠ADB + ∠BDC = 90° + 25° = 115°.
Hence, the value of ∠ADC = 115°.
(ii) ∠ACD = ∠CAB = 25° (∵ alternate angles are equal)
Since sum of angles in a triangle = 180°.
In △ADC,
⇒ ∠ADC + ∠DAC + ∠ACD = 180°
⇒ 115° + ∠DAC + 25° = 180°
⇒ ∠DAC + 140° = 180°
⇒ ∠DAC = 180° - 140° = 40°.
Hence, the value of ∠DAC = 40°.
Answered By
4 Likes
Related Questions
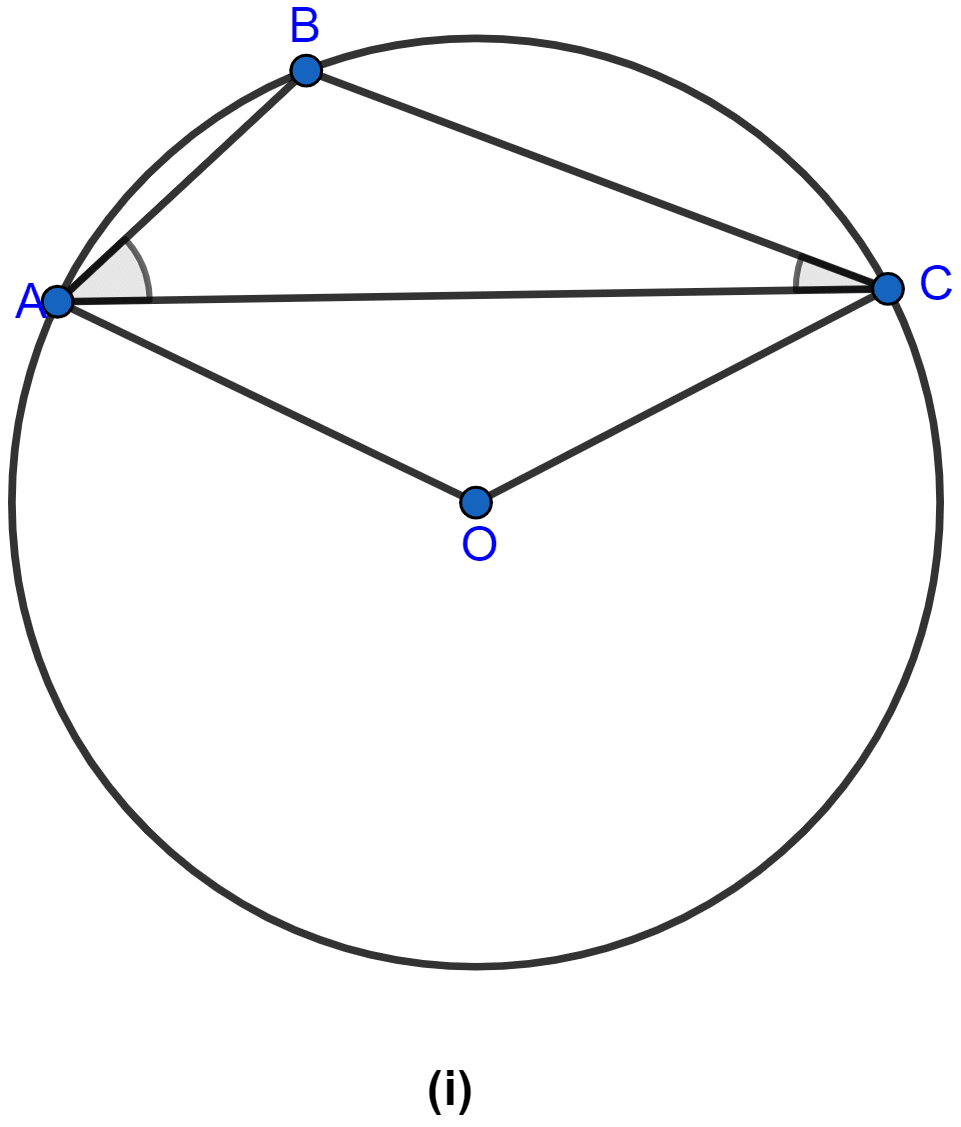
In the figure (i) given below, O is the centre of the circle. Prove that ∠AOC = 2(∠ACB + ∠BAC).
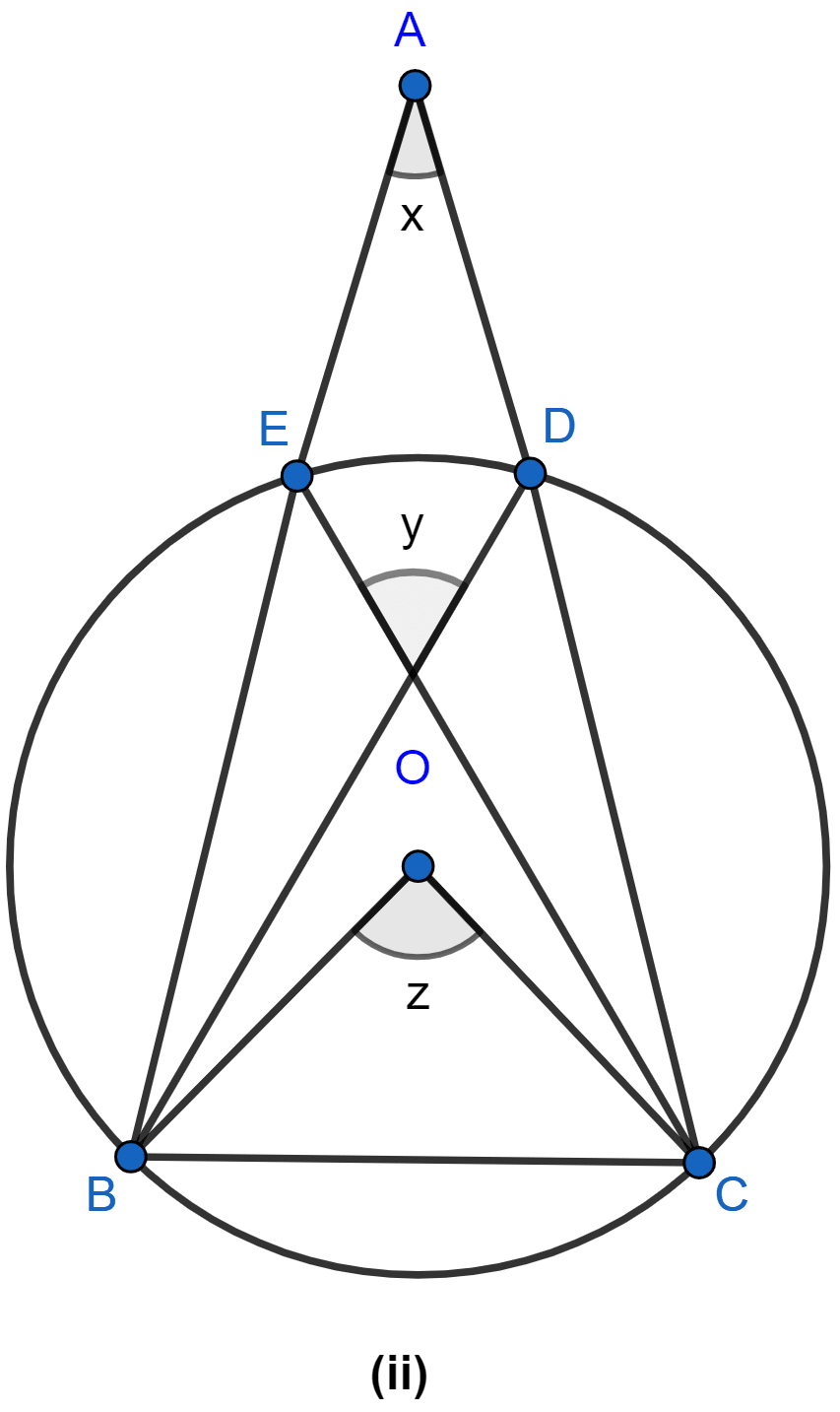
In the figure (ii) given below, O is the centre of the circle. Prove that x + y = z.
In the figure (ii) given below, sides AB and DC of a cyclic quadrilateral are produced to meet at a point P and the sides AD and BC produced to meet at a point Q. If ∠ADC = 75° and ∠BPC = 50°, find ∠BAD and ∠CQD.

In the figure (i) given below, ABDC is a cyclic quadrilateral. If AB = CD, prove that AD = BC.