Mathematics
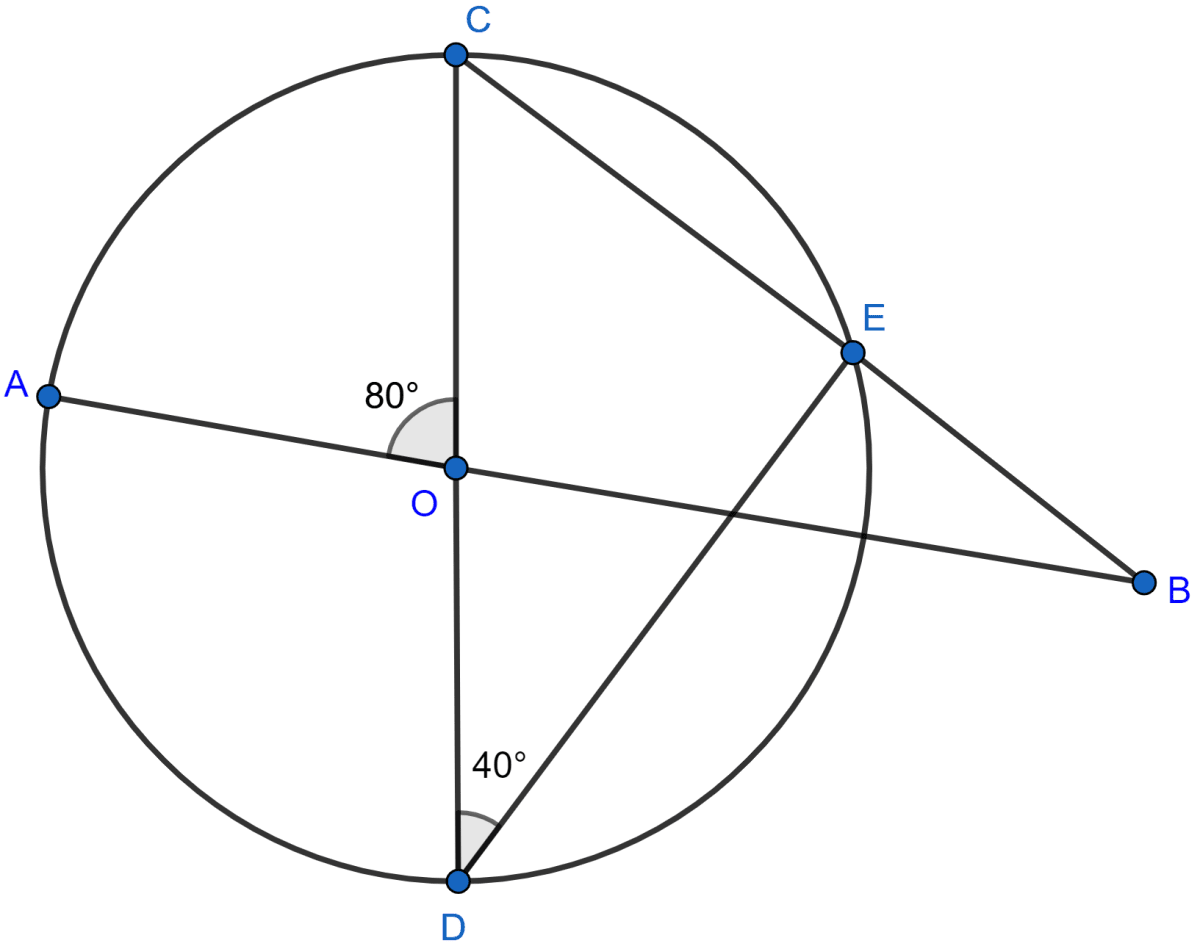
In the figure given alongside, AB and CD are straight lines through the centre O of a circle. If ∠AOC = 80° and ∠CDE = 40°, find the number of degrees in :
(i) ∠DCE,
(ii) ∠ABC.
Answer
(i) From figure,
∠CED = 90° [As angle in semi-circle = 90°]
In △CED,
⇒ ∠CED + ∠CDE + ∠DCE = 180° [By angle sum property of triangle]
⇒ 90° + 40° + ∠DCE = 180°
⇒ ∠DCE + 130° = 180°
⇒ ∠DCE = 180° - 130° = 50°.
Hence, ∠DCE = 50°.
(ii) We know that,
An exterior angle is equal to sum of two opposite interior angles.
In ∆BOC,
⇒ ∠AOC = ∠OCB + ∠OBC
⇒ ∠OBC = ∠AOC - ∠OCB
⇒ ∠OBC = ∠AOC - ∠DCE
⇒ ∠OBC = 80° - 50° = 30°.
From figure,
∠ABC = ∠OBC = 30°.
Hence, ∠ABC = 30°.
Related Questions
In the following figure, O is the centre of the circle, ∠AOB = 60° and ∠BDC = 100°. Find ∠OBC.
In the figure given alongside, AB || CD and O is the center of the circle. If ∠ADC = 25°; find the angle AEB. Give reasons in support of your answer.
In cyclic quadrilateral ABCD, ∠DAC = 27°; ∠DBA = 50° and ∠ADB = 33°. Calculate :
(i) ∠DBC,
(ii) ∠DCB,
(iii) ∠CAB.
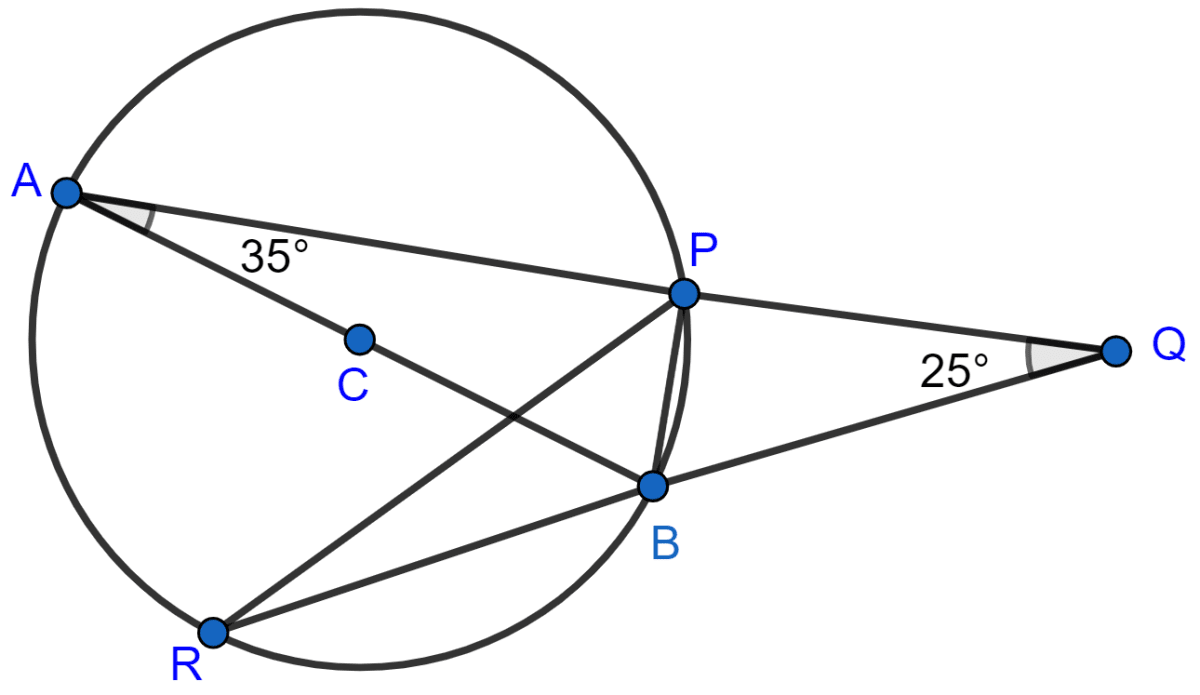
AB is a diameter of the circle APBR as shown in the figure. APQ and RBQ are straight lines. Find :
(i) ∠PRB,
(ii) ∠PBR,
(iii) ∠BPR.
