Mathematics
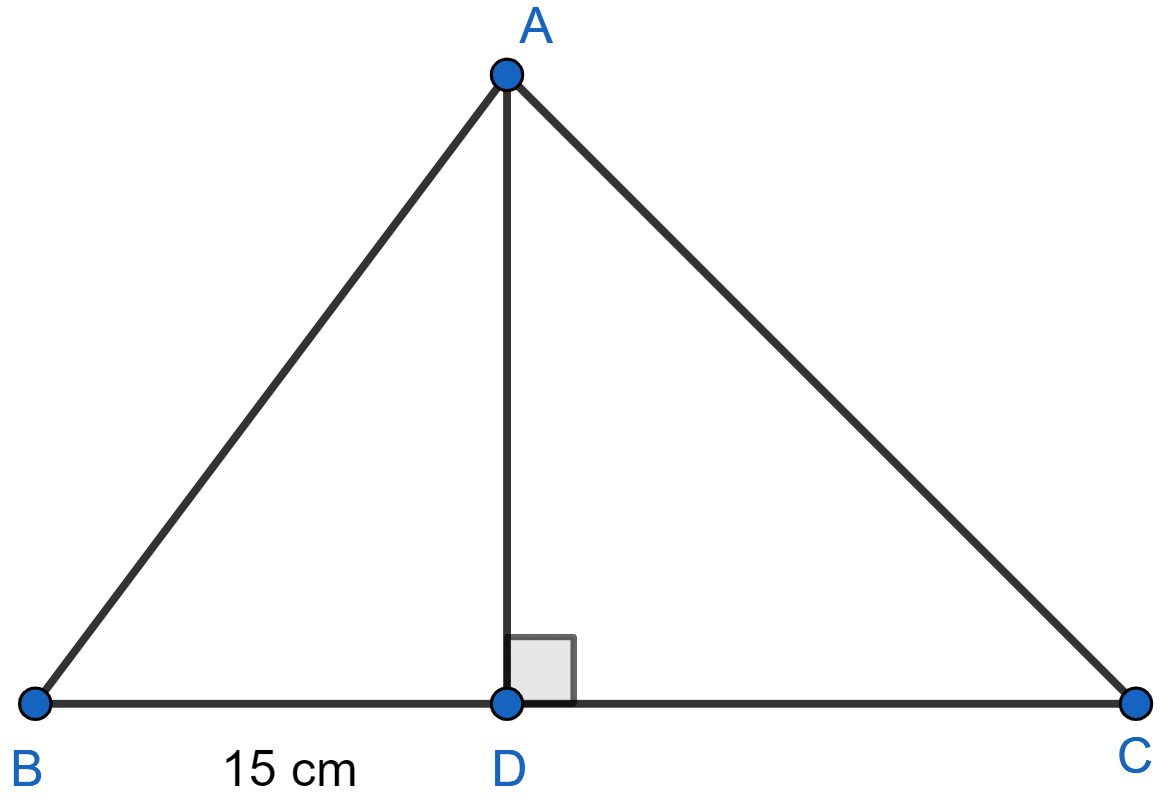
In the figure (3) given below, AD is perpendicular to BC, BD = 15 cm, sin B = and tan C = 1.
(i) Calculate the lengths of AD, AB, DC and AC.
(ii) Show that = -1.
Trigonometrical Ratios
42 Likes
Answer
(i) From figure,
tan C = =
⇒ 1 =
⇒ AD = DC.
sin B = =
⇒ =
Let AD = 4k and AB = 5k.
In right angle triangle ABD,
⇒ AB2 = AD2 + BD2
⇒ (5k)2 = (4k)2 + 152
⇒ 25k2 = 16k2 + 225
⇒ 9k2 = 225
⇒ k2 = = 25
⇒ k = = 5.
AD = 4k = 4 × 5 = 20
AB = 5k = 5 × 5 = 25.
DC = AD = 20.
In right angle triangle ADC,
⇒ AC2 = AD2 + DC2
⇒ AC2 = 202 + 202
⇒ AC2 = 400 +400
⇒ AC2 = 800
⇒ AC = .
Hence, AD = 20, AB = 25, DC = 20 and AC = .
(ii) Calculating tan B, we get :
Calculating cos B, we get :
Substituting value of tan B and cos B in L.H.S. of the equation, = -1, we get :
Hence, proved that tan2 B - = -1.
Answered By
26 Likes
Related Questions
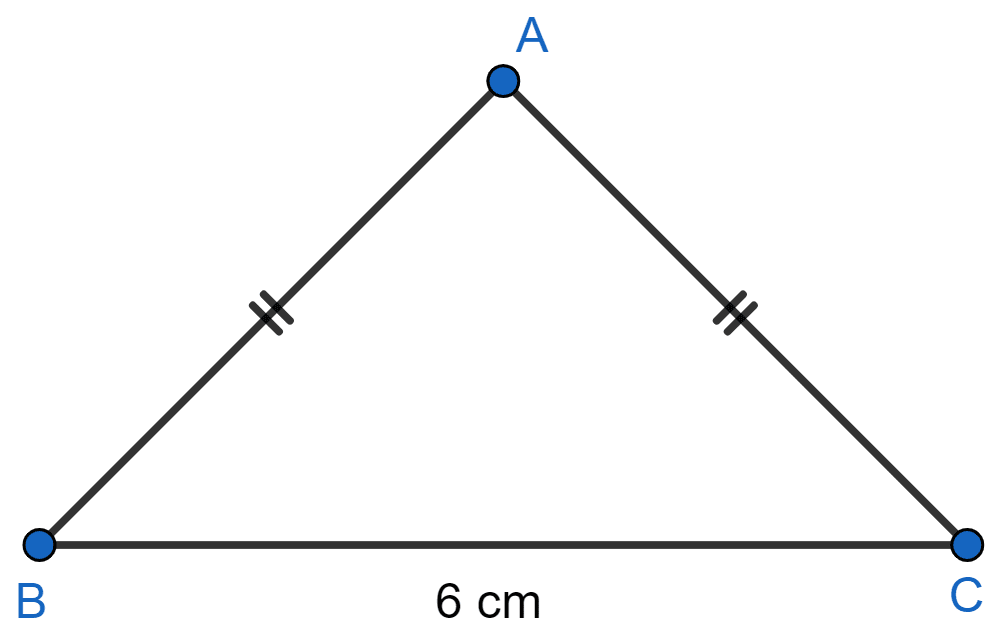
In the figure (1) given below, ∆ABC is isosceles with AB = AC = 5 cm and BC = 6 cm. Find
(i) sin C
(ii) tan B
(iii) tan C - cot B.
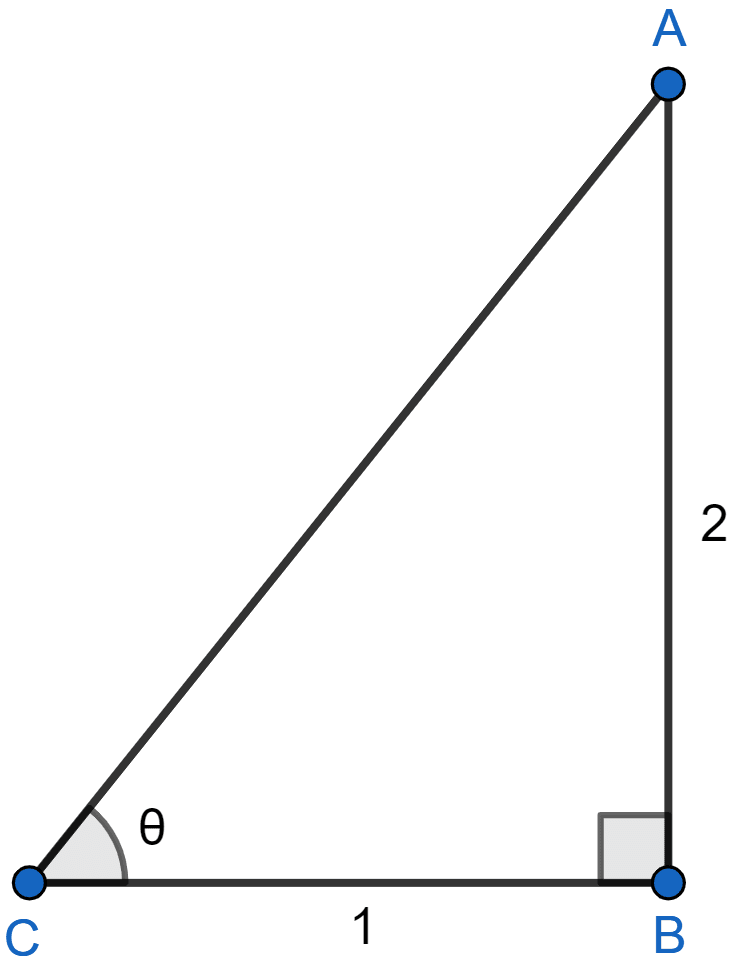
In the figure (2) given below, △ABC is right-angled at B. Given that ∠ACB = θ, side AB = 2 units and side BC = 1 unit, find the value of sin2 θ + tan2 θ.
If sin θ = and θ is acute angle, find
(i) cos θ
(ii) tan θ.
Given that tan θ = and θ is an acute angle, find sin θ and cos θ.