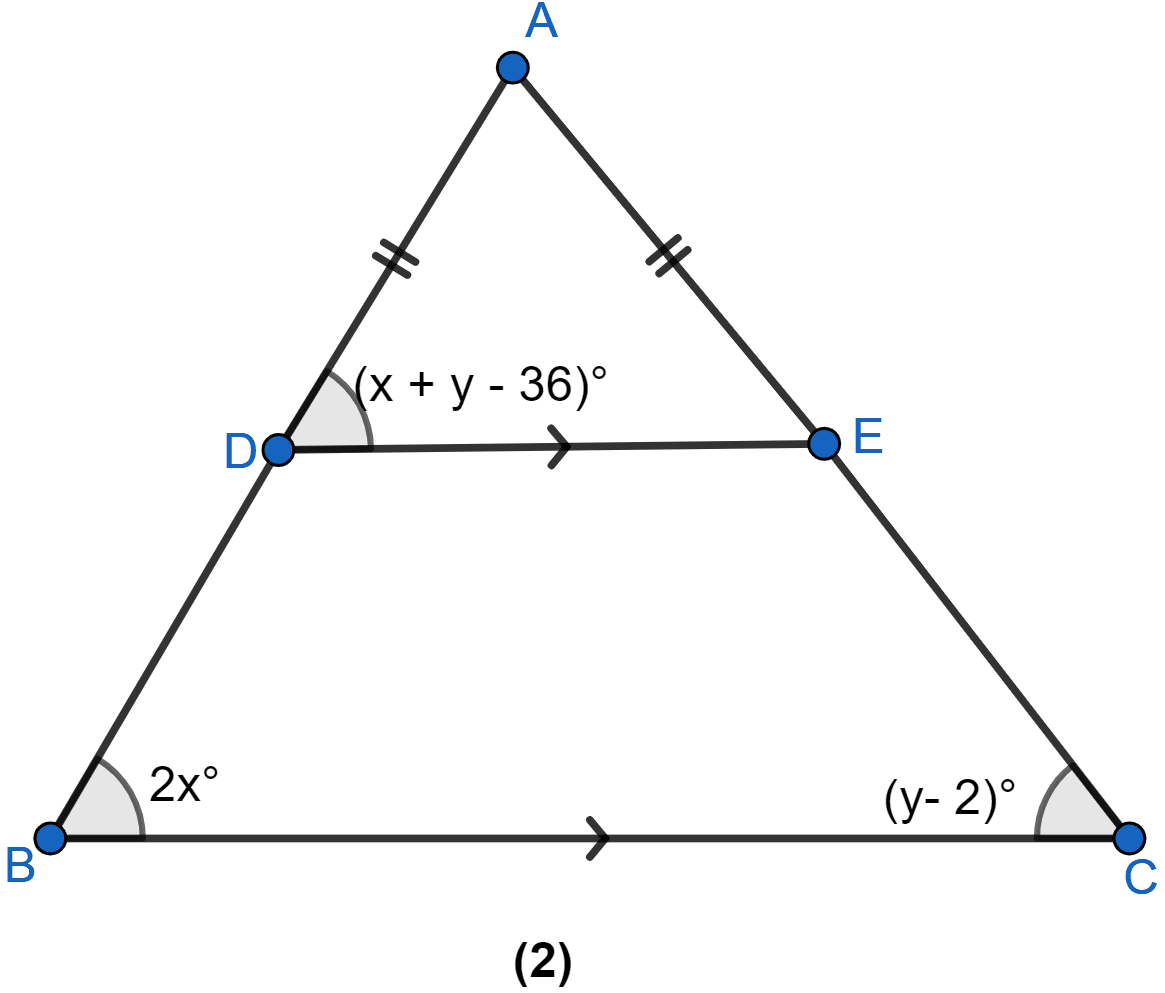
In the figure (2) given below, AB = AC and DE || BC. Calculate

(i) x

(ii) y

(iii) ∠BAC

(i) Since, AB = AC

∠ABC = ∠ACB

⇒ 2x° = (y - 2)°

⇒ y° = 2x° + 2° …….(i)

From figure,

∠ADE = ∠ABC (Corresponding angles)

⇒ x° + y° - 36° = 2x°

⇒ y° - 36° = x°

Substituting value of y from (i) in above equation,

⇒ 2x° + 2° - 36° = x°

⇒ 2x° - x° = 34°

⇒ x° = 34°

⇒ x = 34.

Hence, x = 34.

(ii) Substituting value of x in (i),

⇒ y° = 2(34)° + 2°

⇒ y° = 68° + 2° = 70°.

⇒ y = 70.

Hence, y = 70.

(iii) From figure,

∠BAC = 180° - (2x° + y° - 2°)

= 180° - (2 × 34° + 70° - 2°)

= 180° - (68° + 68°)

= 180° - 136° = 44°.

Hence, ∠BAC = 44°.