Mathematics
In the adjoining figure, O is the center of a circular arc and AOB is a line segment. Find the perimeter and the area of the shaded region correct to one decimal place. (Take π = 3.142)
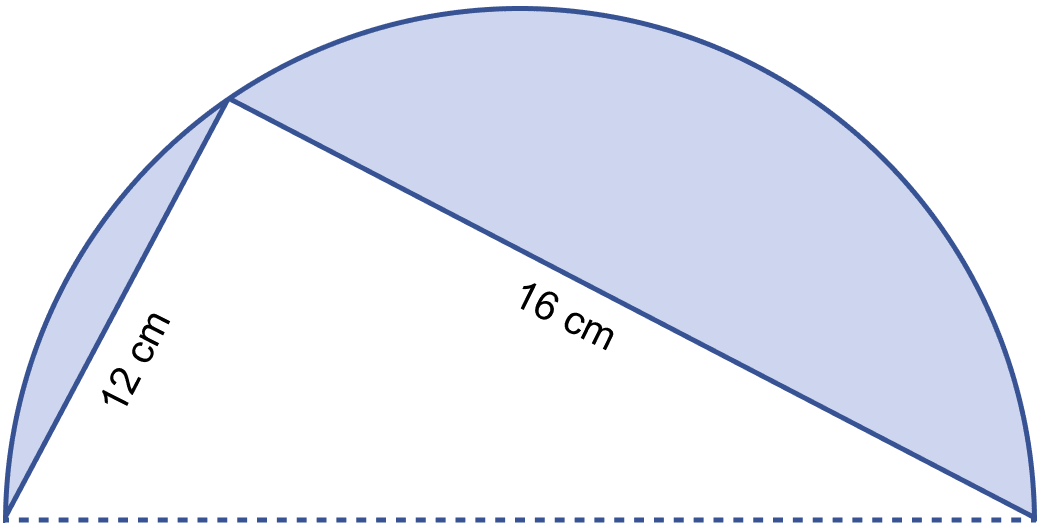
Mensuration
7 Likes
Answer
Angle in semi-circle = 90°
∴ ∠ACB = 90°
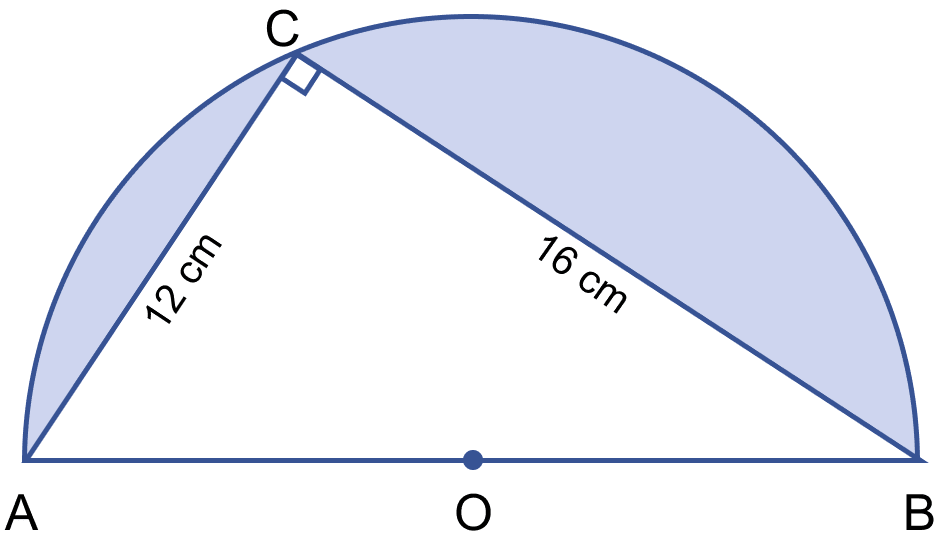
Using pythagoras theorem,
⇒ AB2 = AC2 + BC2
⇒ AB2 = 122 + 162
⇒ AB2 = 144 + 256
⇒ AB2 = 400
⇒ AB = = 20 cm.
AB is the diameter of circle,
∴ Radius = = = 10 cm.
Area of shaded region = Area of semi-circle - Area of triangle
Perimeter of shaded region = Circumference of semi-circle + AC + CB
= πr + 12 + 16
= 3.142 × 10 + 28
= 31.42 + 28
= 59.42 cm.
Hence, perimeter of shaded region = 59.42 cm and area of semi-circle = 61.1 cm2.
Answered By
3 Likes
Related Questions
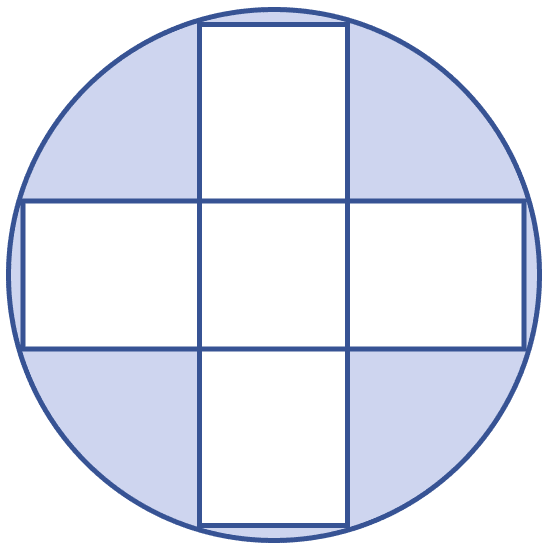
In the figure (ii) given below, there are five squares each of side 2 cm.
(i) Find the radius of the circle.
(ii) Find the area of the shaded region. (Take π = 3.14).
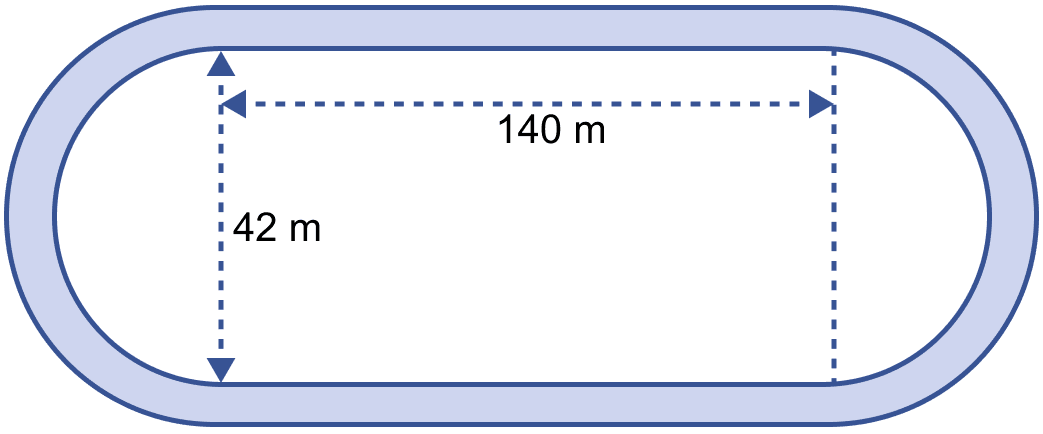
The adjoining sketch shows a running track 3.5 m wide all around which consists of two straight paths and two semicircular rings. Find the area of the track.
A road 3.5 m wide surrounds a circular park whose circumference is 88 m. Find the cost of paving the road at the rate of ₹60 per square metre.
In the figure (i) given below, the radius is 3.5 cm. Find the perimeter of the quarter of the circle.
