Mathematics
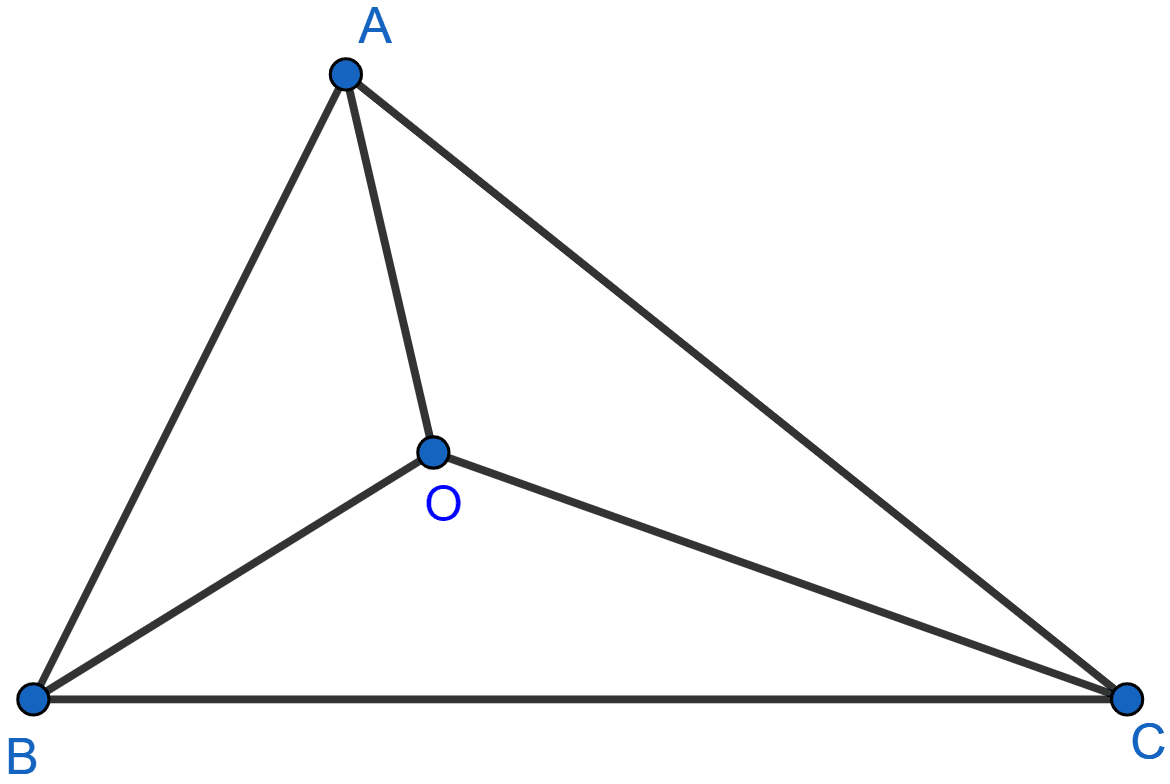
In the adjoining figure, AP ⊥ l and PR > PQ. Show that AR > AQ.
Triangles
14 Likes
Answer
Take a point S on PR such that PS = PQ.
Join A and S. Mark angles as shown.
PQ = PS
AP = AP (Common)
∠APQ = ∠APS (Both are equal to 90°)
△APQ ≅ △APS (By SAS axiom)
We know that corresponding parts of congruent triangles are equal.
∠1 = ∠2.
In △ARS,
∠2 > ∠3 (As exterior angle is greater than each interior opposite angle.)
∴ ∠1 > ∠3
⇒ AR > AQ (As side opposite to greater angle is greater.)
Hence, proved that AR > AQ.
Answered By
6 Likes
Related Questions
In the figure (1) given below, AD = BD = DC and ∠ACD = 35°. Show that
(i) AC > DC
(ii) AB > AD.

In the figure (2) given below, prove that
(i) x + y = 90°
(ii) z = 90°
(iii) AB = BC.
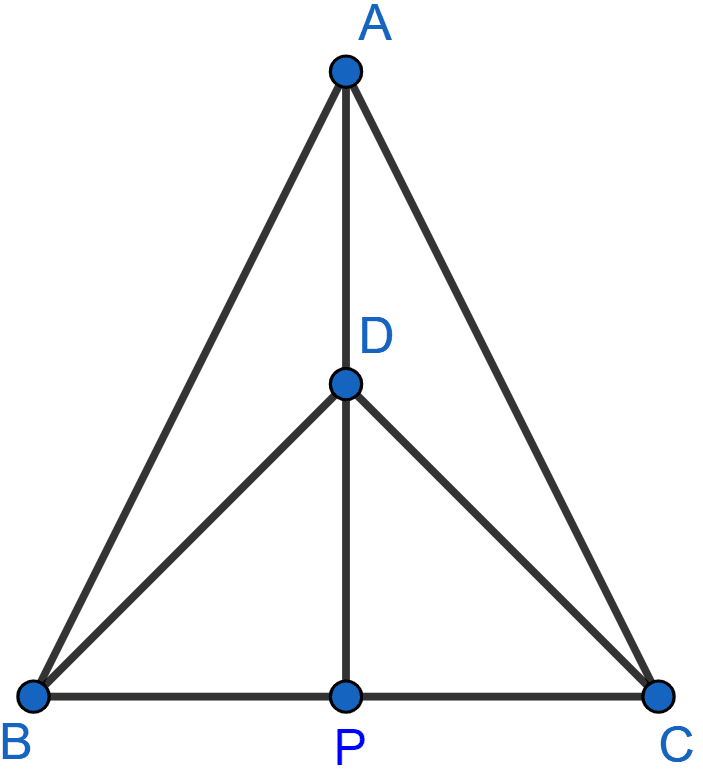
In the adjoining figure, ABC and DBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC. If AD is extended to intersect BC at P, show that
(i) △ABD ≅ △ACD
(ii) △ABP ≅ △ACP
(iii) AP bisects ∠A as well as ∠D
(iv) AP is the perpendicular bisector of BC.
If O is any point in the interior of a triangle ABC, show that OA + OB + OC > (AB + BC + CA).