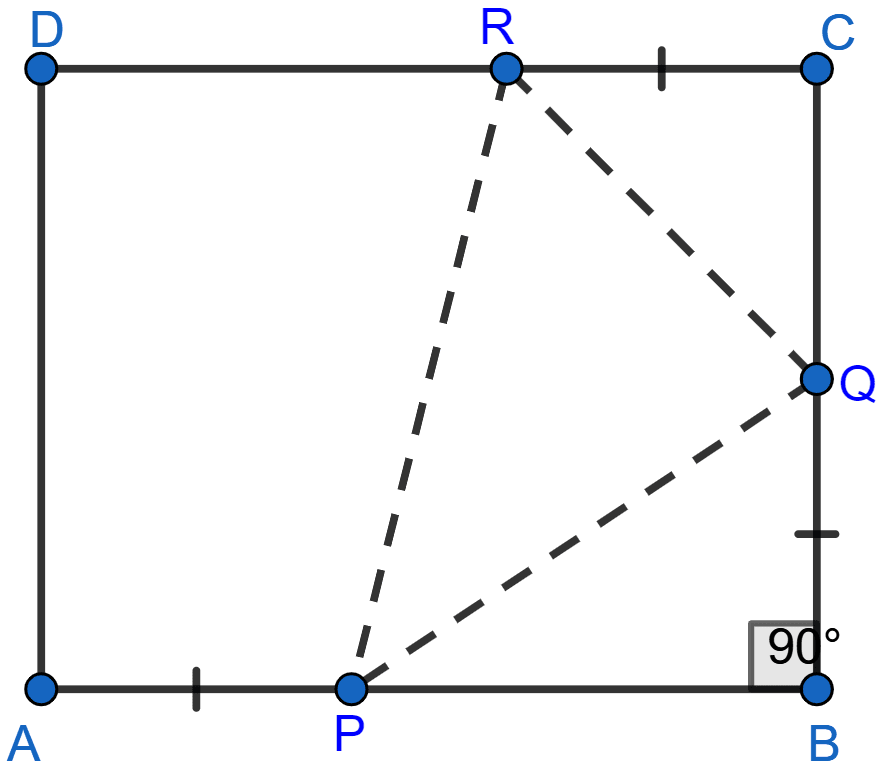
In the adjoining figure, ABCD is a square. P, Q and R are points on the sides AB, BC and CD respectively such that AP = BQ = CR and ∠PQR = 90°. Prove that

(a) △PBQ ≅ △QCR

(b) PQ = QR

(c) ∠PRQ = 45°

(a) Given, ABCD is a square.

∴ AB = BC.

Given, AP = BQ.

∴ AB - AP = BC - BQ …….(i)

In △PBQ and △QCR,

BQ = CR (Given)

From (i) we get,

PB = QC

∠PBQ = ∠QCR (Both are equal to 90°, as each angle in square = 90°.)

Hence, proved △PBQ ≅ △QCR by SAS axiom.

(b) We know that corresponding parts of congruent triangles are equal.

∴ PQ = QR.

Hence, proved that PQ = QR.

(c) Considering △PQR.

We know PQ = QR and ∠Q = 90°.

Hence, △PQR is an isosceles triangle with ∠P = ∠R = x.

Sum of angles of triangle = 180°.

⇒ ∠P + ∠Q + ∠R = 180°

⇒ x + 90° + x = 180°

⇒ 2x = 90°

⇒ x = 45°.

Hence, proved that ∠PRQ = 45°.