Mathematics
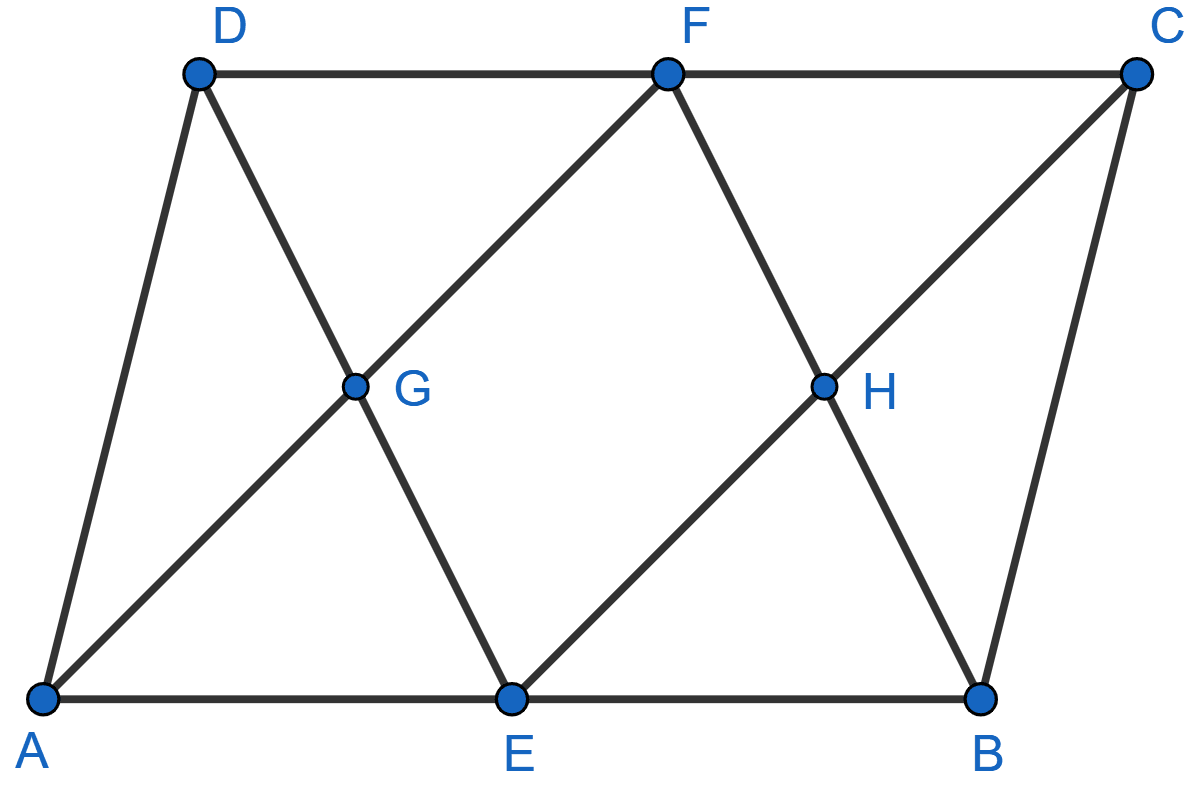
In the adjoining figure, ABCD is a parallelogram. E and F are mid-points of the sides AB and CD respectively. The straight lines AF and BF meet the straight lines ED and EC in points G and H respectively. Prove that
(i) △HEB ≅ △HCF
(ii) GEHF is a parallelogram.
Mid-point Theorem
67 Likes
Answer
(i) We know that,
ABCD is a parallelogram,
∴ FC || BE
∠CEB = ∠FCE (Alternate angles)
⇒ ∠HEB = ∠FCH …….(1)
∠EBF = ∠CFB (Alternate angles)
⇒ ∠EBH = ∠CFH …….(2)
Here E and F are mid-points of AB and CD
BE = AB ……..(3)
CF = CD ……..(4)
We know that ABCD is a parallelogram,
AB = CD
Now dividing both sides by
AB = CD
Using equations 3 and 4 we get,
BE = CF …….(5)
In △HEB and △HCF,
∠HEB = ∠FCH (Using eqn. i)
∠EBH = ∠CFH (Using eqn. ii)
BE = CF (Using eqn. v)
∴ △HEB ≅ △HCF (By ASA axiom of congruency)
Hence, proved that △HEB ≅ △HCF.
(ii) AB = CD (As ABCD is a parallelogram)
Hence, AE = CF (As E and F are mid-points of the sides AB and CD respectively)
As, AB || CF we can say that,
AE || CF
Since, AE = CF and AE || CF
∴ AECF is a parallelogram.
∴ AF || EC
From figure we get,
GF || EH ……..(1)
AB = CD (As ABCD is a parallelogram)
Hence, DF = EB (As E and F are mid-points of the sides AB and CD respectively) and DF || EB.
Since, DF = EB and DF || EB
∴ DEBF is a parallelogram.
∴ DE || FB
From figure we get,
GE || FH ……..(2)
From 1 and 2 we get,
GF || EH and GE || FH.
∴ GEHF is a parallelogram.
Hence, proved that GEHF is a parallelogram.
Answered By
41 Likes
Related Questions
Show that the quadrilateral formed by joining the mid-points of the adjacent sides of a square, is also a square.
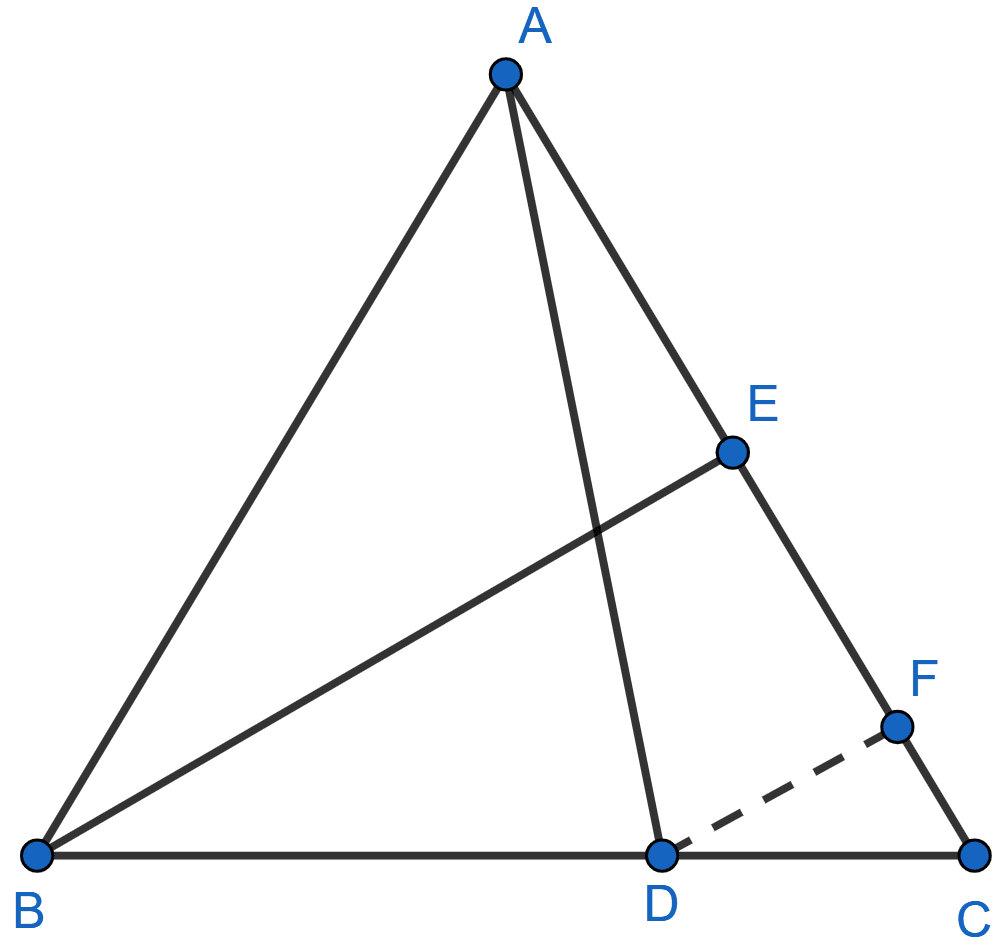
In the adjoining figure, AD and BE are medians of △ABC. If DF || BE, prove that CF =
ABC is an isosceles triangle with AB = AC. D, E and F are mid-points of the sides BC, AB and AC respectively. Prove that line segment AD is perpendicular to EF and is bisected by it.
In the quadrilateral given below, AB || DC, E and F are mid-points of AD and BD respectively. Prove that
(i) G is the mid-point of BC
(ii) EG = (AB + DC).