Mathematics
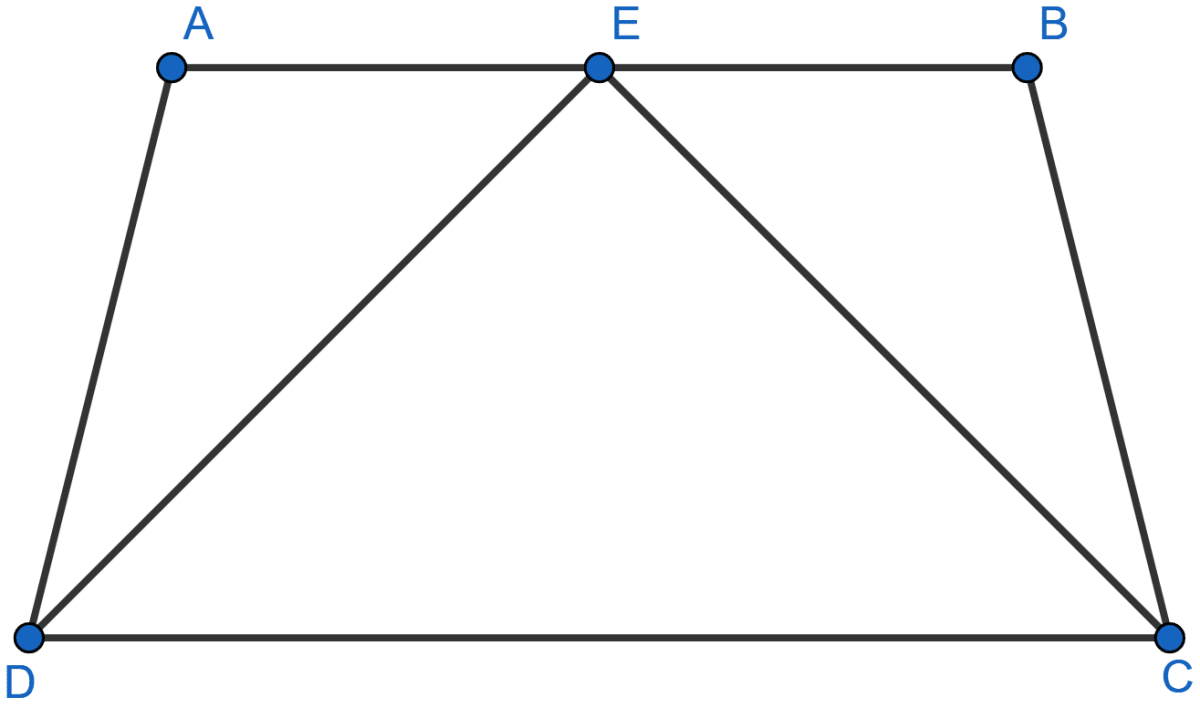
In the adjoining figure, AB || DC. CE and DE bisects ∠BCD and ∠ADC respectively. Prove that AB = AD + BC.
Triangles
47 Likes
Answer
Given,
DE bisects ∠D.
∴ ∠EDA = ∠EDC = .
AB || CD and DE cuts these parallel lines.
∠DEA = ∠EDC = .
In △ADE,
∠ADE = ∠DEA = .
Hence, AD = AE.
Given,
CE bisects ∠C.
∴ ∠ECB = ∠ECD = .
AB || CD and CE cuts these parallel lines.
∠CEB = ∠ECD = .
In △BCE,
∠BEC = ∠BCE =
Hence, BE = BC.
⇒ AB = AE + BE = AD + BC
⇒ AB = AD + BC.
Hence, proved that AB = AD + BC.
Answered By
24 Likes
Related Questions
In the adjoining figure, PQ || BA and RS || CA. If BP = RC, prove that:
(i) △BSR ≅ △PQC
(ii) BS = PQ
(iii) RS = CQ.

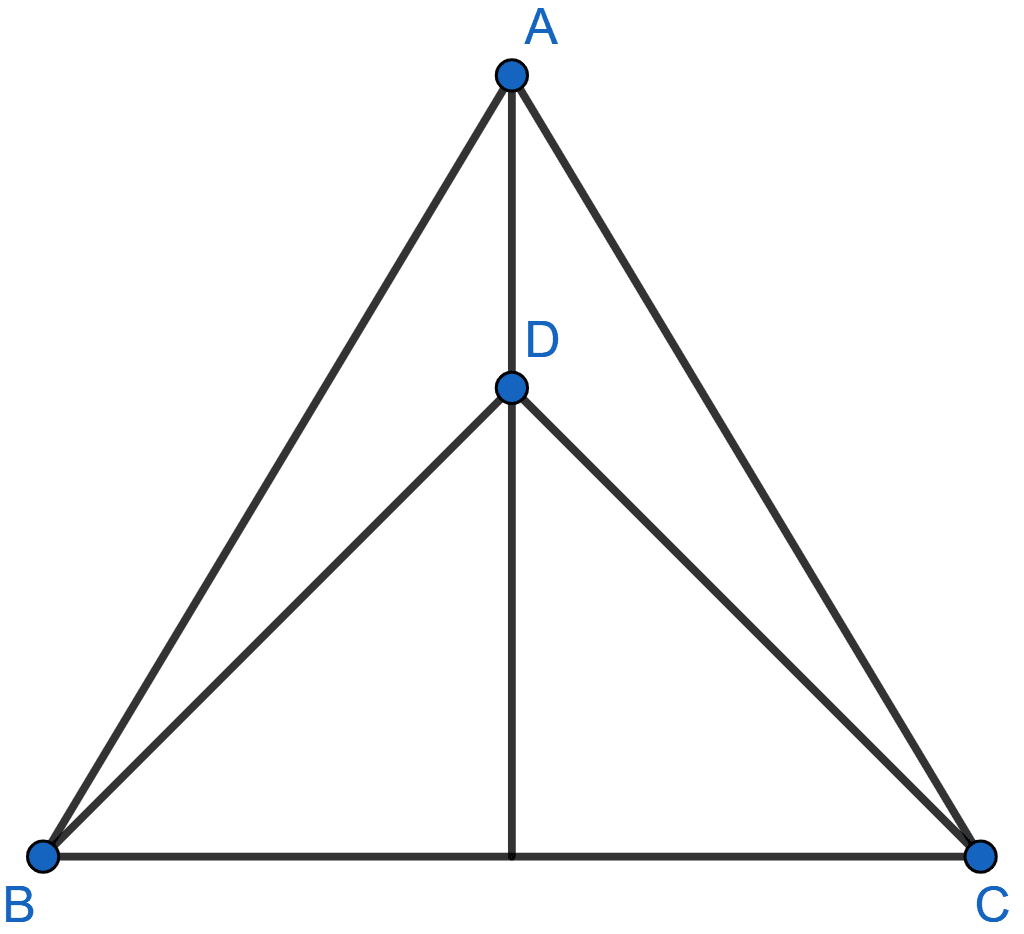
In the adjoining figure, AB = AC, D is a point in the interior of △ABC such that ∠DBC = ∠DCB. Prove that AD bisects ∠BAC of △ABC.
In △ABC, D is a point on BC such that AD is the bisector of ∠BAC. CE is drawn parallel to DA to meet BD produced at E. Prove that △CAE is isosceles.
In the adjoining figure, ABC is a right angled triangle at B. ADEC and BCFG are squares. Prove that AF = BE.
