Mathematics
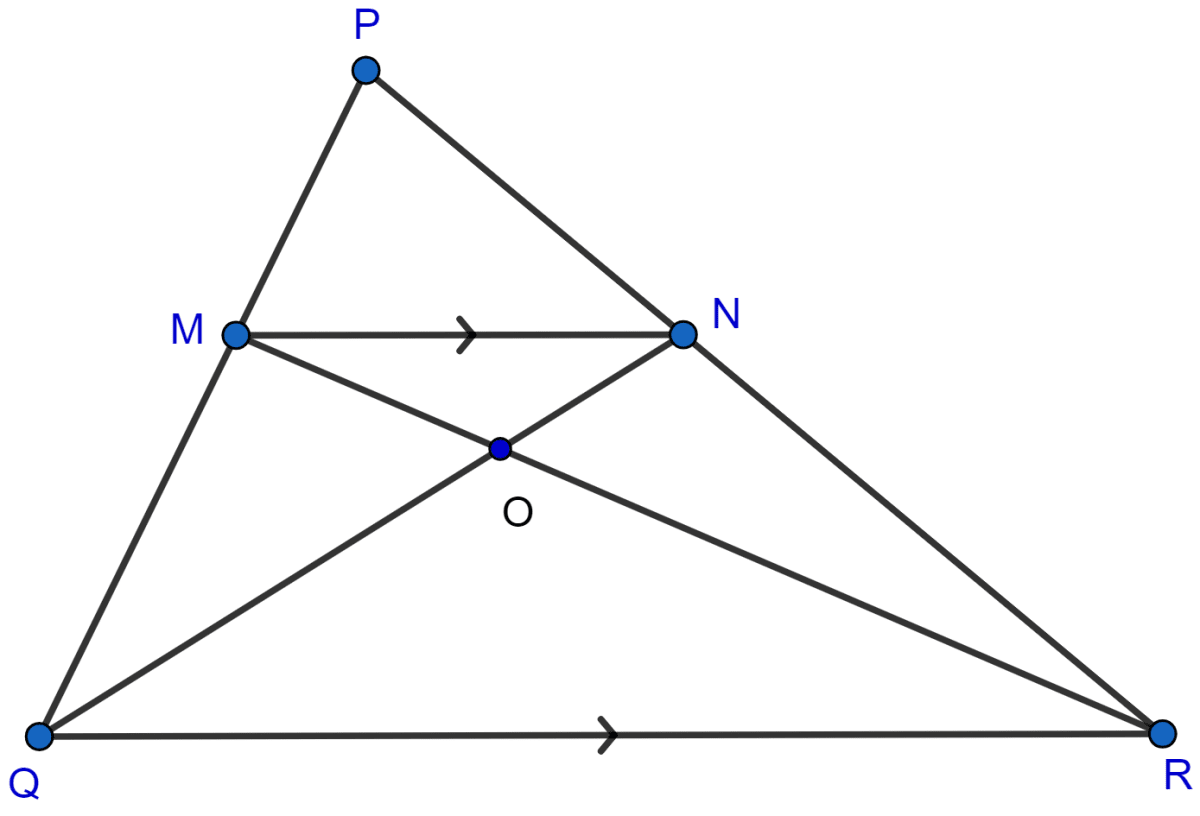
In △PQR, MN is parallel to QR and
(i) Find .
(ii) Prove that △OMN and △ORQ are similar.
(iii) Find area of △OMN : area of △ORQ.
Similarity
44 Likes
Answer
(i) Considering △PMN and △PQR,
∠ P = ∠ P (Common angles)
∠ PMN = ∠ PQR (Corresponding angles are equal)
Hence, by AA axiom △PMN ~ △PQR.
Given,
Since triangles are similar hence the ratio of the corresponding sides will be equal,
Hence,
(ii) Considering △OMN and △ORQ,
∠ MON = ∠ QOR (Vertically opposite angles are equal)
∠ OMN = ∠ ORQ (Alternate angles are equal)
Hence, by AA axiom △OMN ~ △ORQ.
(iii) We know that, the ratio of the areas of two similar triangles is equal to the ratio of the square of their corresponding sides.
Hence, the ratio of the Area of △OMN : Area of △ORQ = 4 : 25.
Answered By
30 Likes
Related Questions
In the given figure, AB and DE are perpendiculars to BC.
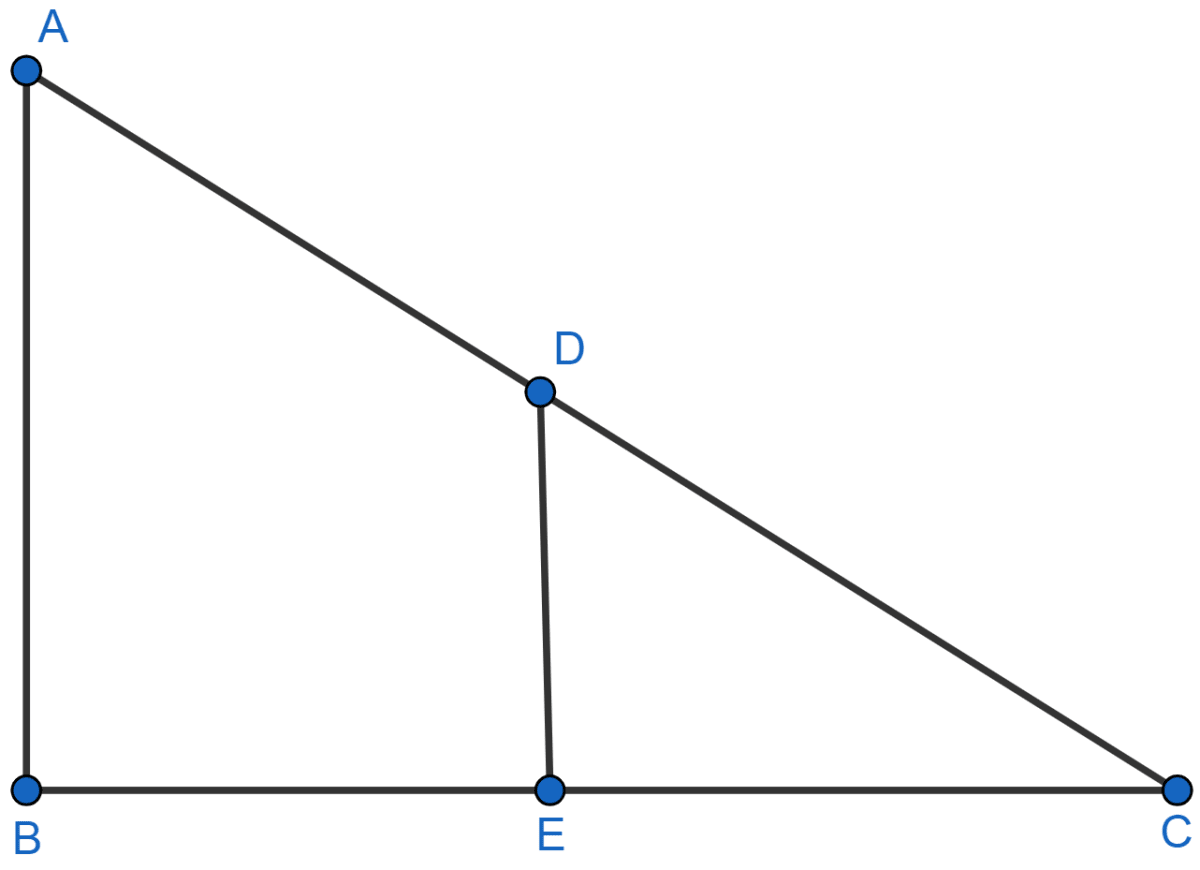
(i) Prove that △ABC ~ △DEC.
(ii) If AB = 6 cm, DE = 4 cm and AC = 15 cm, calculate CD.
(iii) Find the ratio of the area of △ABC : area of △DEC.
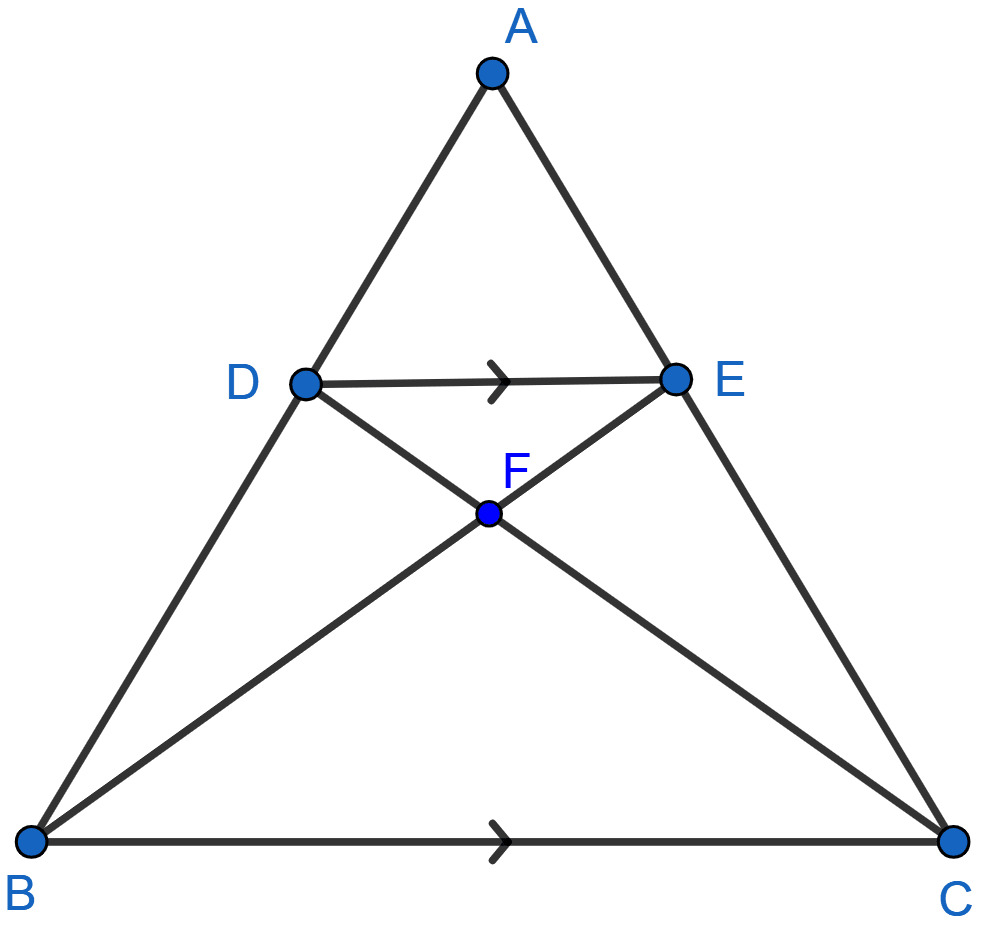
In the adjoining figure, ABC is a triangle. DE is parallel to BC and
(i) Determine the ratio
(ii) Prove that △DEF is similar to △CBF. Hence, find
(iii) What is the ratio of the areas of △DEF and △CBF ?
In △ABC, AP : PB = 2 : 3. PO is parallel to BC and is extended to Q so that CQ is parallel to BA. Find :
(i) area △APO : area △ABC
(ii) area △APO : area △CQO.

In the figure (i) given below, ABCD is a trapezium in which AB || DC and AB = 2 CD. Determine the ratio of the areas of △AOB and △COD.
