Mathematics
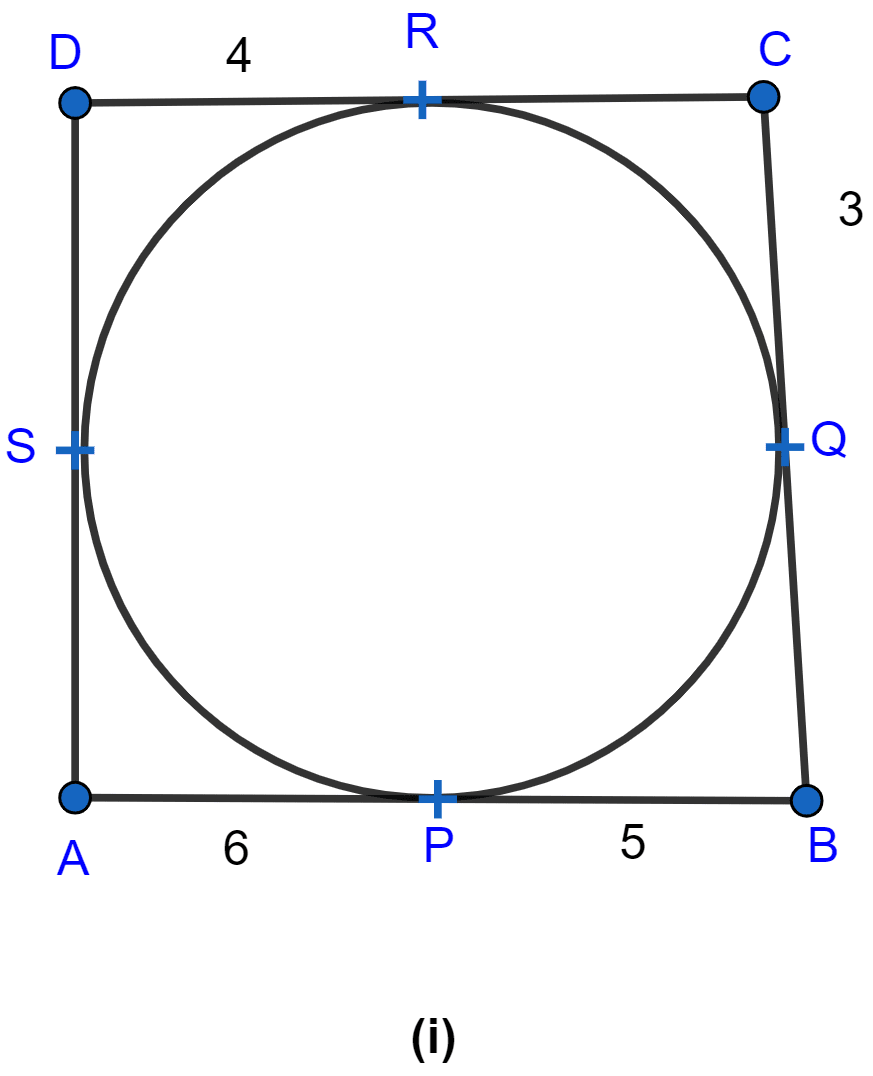
In figure (i) given below, quadrilateral ABCD is circumscribed ; find the perimeter of quadrilateral ABCD.
Circles
20 Likes
Answer
From A, AP and AS are the tangents to the circle.
∴ AS = AP = 6 cm. (∵ if two tangents are drawn to a circle from an external point then the tangents have equal lengths.)
From B, BP and BQ are the tangents to the circle.
∴ BQ = BP = 5 cm. (∵ if two tangents are drawn to a circle from an external point then the tangents have equal lengths.)
From C, CQ and CR are the tangents to the circle.
∴ CR = CQ = 3 cm. (∵ if two tangents are drawn to a circle from an external point then the tangents have equal lengths.)
From D, DS and DR are the tangents to the circle.
∴ DS = DR = 4 cm. (∵ if two tangents are drawn to a circle from an external point then the tangents have equal lengths.)
Therefore, perimeter of the quadrilateral ABCD
Hence, the perimeter of ABCD = 36 cm.
Answered By
12 Likes
Related Questions
In figure (i) given below, triangle ABC is circumscribed, find x.

In figure (ii) given below, quadrilateral ABCD is circumscribed, find x.

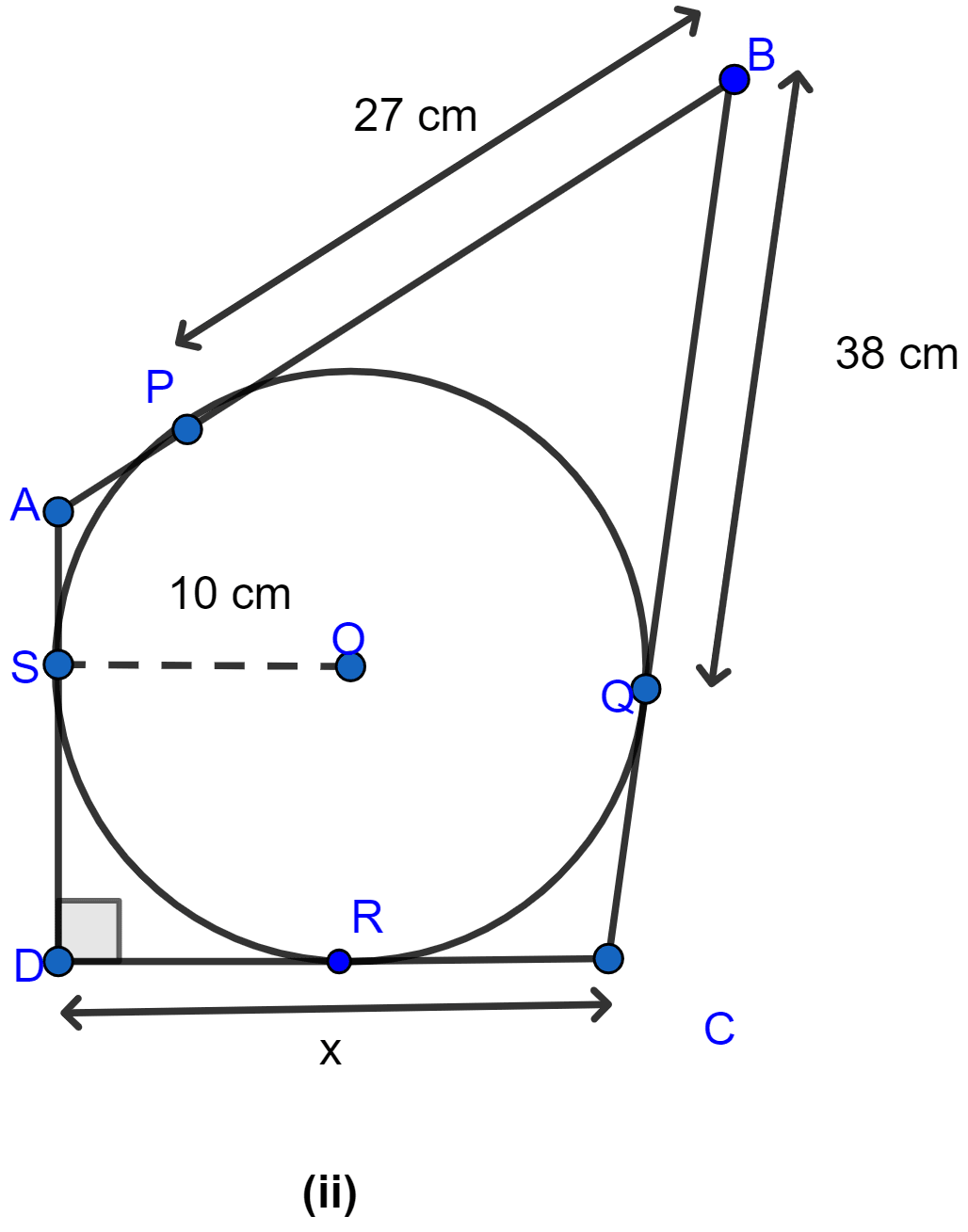
In figure (ii) given below, quadrilateral ABCD is circumscribed and AD ⊥ DC; find x if radius of incircle is 10 cm.
In figure (i) given below, O is the centre of the circle and AB is a tangent at B. If AB = 15 cm and AC = 7.5 cm, find the radius of the circle.
