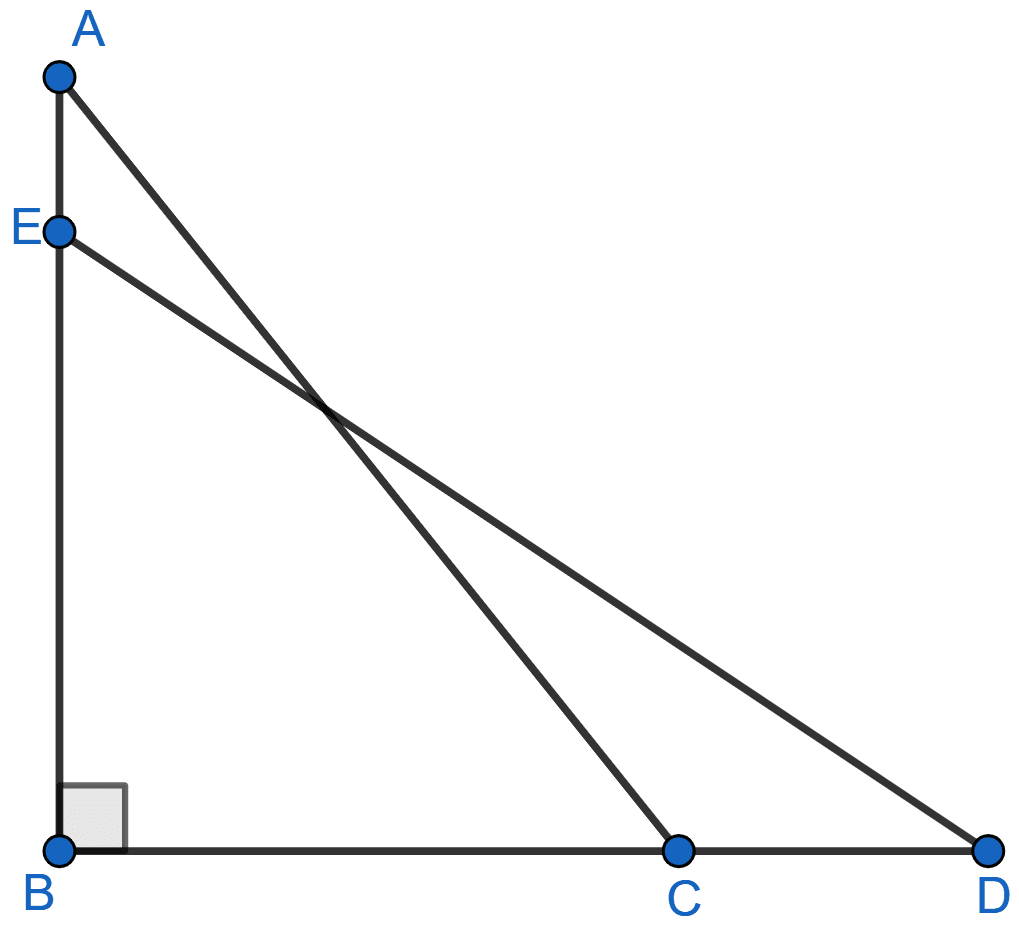
In figure given below, BC = 5 cm, ∠B = 90°, AB = 5AE, CD = 2AE and AC = ED. Calculate the lengths of EA, CD, AB and AC.

In right angle △ABC,

By pythagoras theorem,

⇒ AC² = AB² + BC²

⇒ AC² = (5AE)² + 5²

⇒ AC² = 25AE² + 25

⇒ AC² = 25(AE² + 1) …….(i)

From figure,

EB = AB - AE = 5AE - AE = 4AE.

In right angle △BED,

⇒ ED² = EB² + BD²

⇒ ED² = (4AE)² + (5 + 2AE)²

⇒ ED² = 16AE² + 25 + 4AE² + 20AE

⇒ ED² = 20AE² + 20AE + 25 …….(ii)

Given, AC = ED.

∴ From (i) and (ii) we get,

⇒ 25(AE² + 1) = 20AE² + 20AE + 25

⇒ 25AE² + 25 = 20AE² + 20AE + 25

⇒ 25AE² - 20AE² - 20AE + 25 - 25 = 0

⇒ 5AE² - 20AE = 0

⇒ 5AE(AE - 4) = 0

⇒ 5AE = 0 or AE - 4 = 0

⇒ AE = 0 or AE = 4 cm.

Since, side cannot be 0 so AE ≠ 0.

AE = 4 cm,

CD = 2AE = 8 cm,

AB = 5AE = 20 cm,

Substituting value of AE in (i) we get,

⇒ AC² = 25(AE² + 1)

⇒ AC² = 25(4² + 1)

⇒ AC² = 25(16 + 1) = 25 × 17 = 425

⇒ AC = $\sqrt{425} = 5\sqrt{17}$ cm.

Hence, EA = 4 cm, CD = 8 cm, AB = 20 cm and AC = $5\sqrt{17}$ cm.