Mathematics
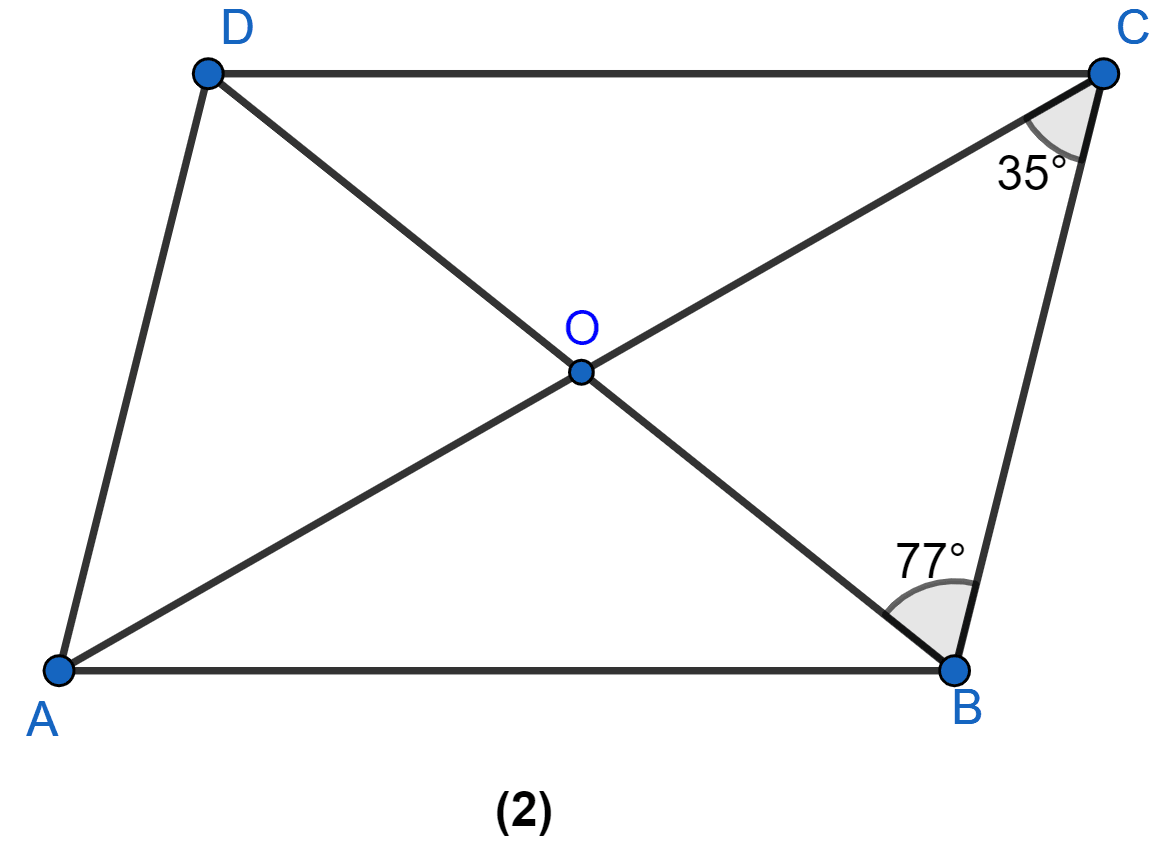
In figure (2) given below, ABCD is a parallelogram. Find the angles of △AOD.
Rectilinear Figures
46 Likes
Answer
Sum of angles of a triangle = 180°
In △BOC,
⇒ ∠BOC + ∠OCB + ∠CBO = 180°
⇒ ∠BOC + 35° + 77° = 180°
⇒ ∠BOC = 180° - 35° - 77° = 68°.
From figure,
∠AOD = ∠BOC = 68° (Vertically opposite angles are equal)
∠OAD = ∠OCB = 35° (Alternate angles are equal)
∠ADO = ∠CBO = 77° (Alternate angles are equal)
Hence, ∠AOD = 68°, ∠OAD = 35°, ∠ADO = 77°.
Answered By
31 Likes
Related Questions
If an angle of a parallelogram is two-thirds of its adjacent angle, find the angles of the parallelogram.
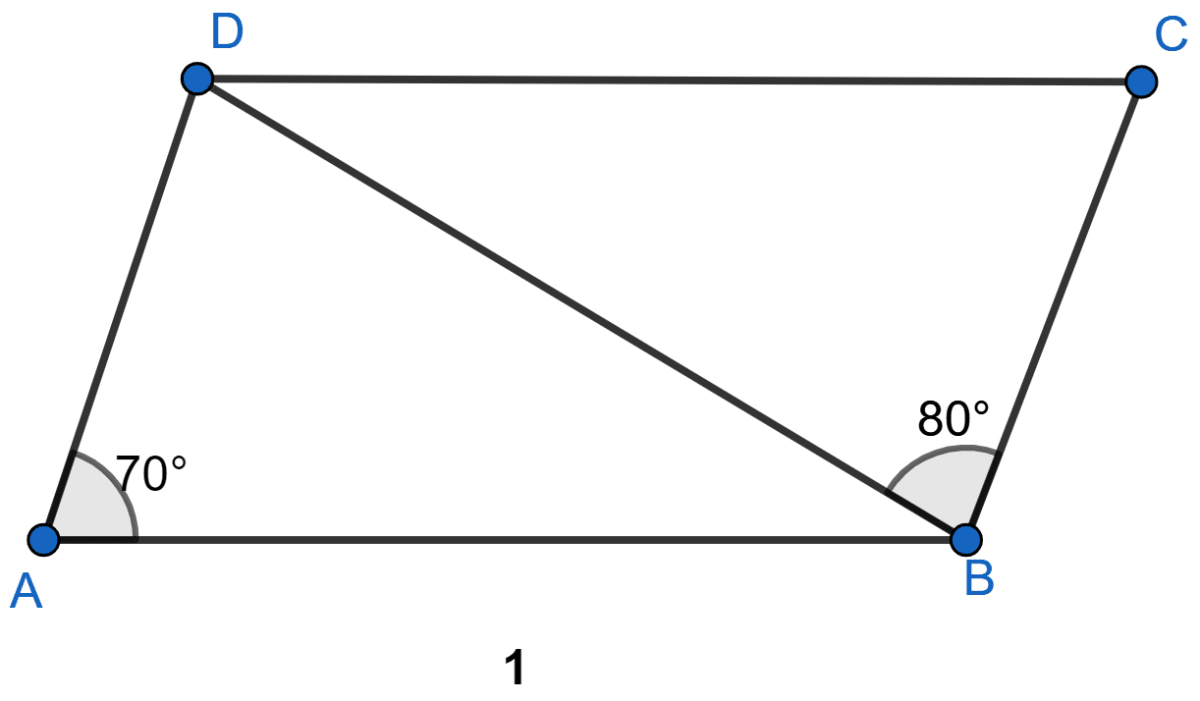
In the figure (1) given below, ABCD is a parallelogram in which ∠DAB = 70°, ∠DBC = 80°. Calculate angles CDB and ADB.
In figure (3) given below, ABCD is a rhombus. Find the value of x.

In figure (1) given below, ABCD is a parallelogram with perimeter 40. Find the values of x and y.
