Mathematics
In cyclic quadrilateral ABCD; AD = BC, ∠BAC = 30° and ∠CBD = 70°; find :
(i) ∠BCD
(ii) ∠BCA
(iii) ∠ABC
(iv) ∠ADC
Circles
5 Likes
Answer
(i) From figure,
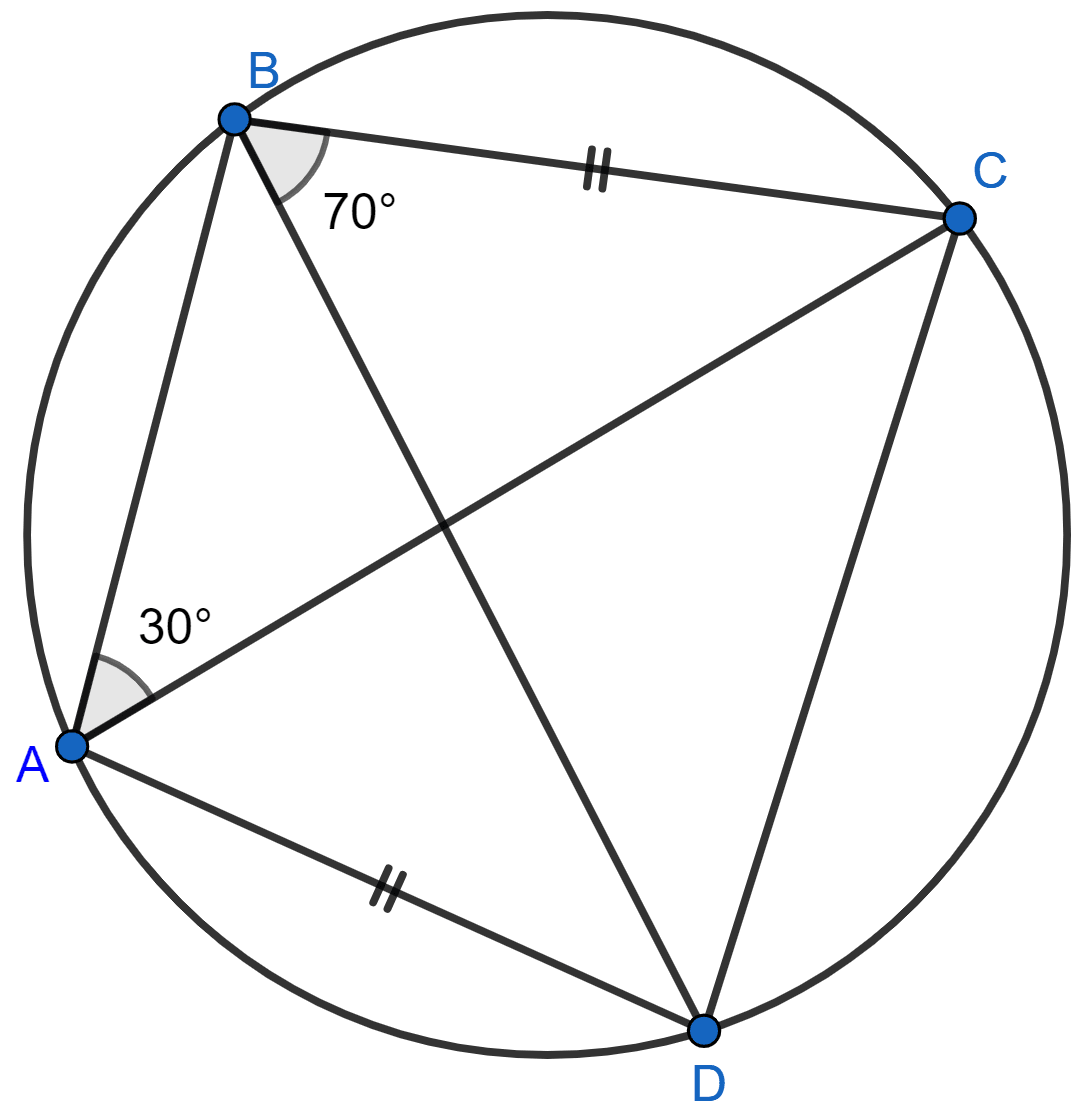
∠DAC = ∠CBD = 70° [Angles in same segment are equal]
∠BAD = ∠BAC + ∠DAC = 30° + 70° = 100°.
Since, sum of opposite angles of cyclic quadrilateral = 180°.
⇒ ∠BAD + ∠BCD = 180°
⇒ 100° + ∠BCD = 180°
⇒ ∠BCD = 180° - 100° = 80°.
Hence, ∠BCD = 80°.
(ii) Since, AD = BC
∠ACD = ∠BDC …………(1) [Equal chords subtends equal angles]
As,
Angles in same segment are equal.
∠ACB = ∠ADB ………..(2)
Adding (1) and (2) we get,
⇒ ∠ACD + ∠ACB = ∠BDC + ∠ADB
⇒ ∠BCD = ∠ADC = 80°.
In △BCD,
⇒ ∠CBD + ∠BCD + ∠BDC = 180°
⇒ 70° + 80° + ∠BDC = 180°
⇒ 150° + ∠BDC = 180°
⇒ ∠BDC = 180° - 150° = 30°.
∠ACD = ∠BDC = 30° [As equal chords subtends equal angles.]
From figure,
⇒ ∠BCA = ∠BCD - ∠ACD = 80° - 30° = 50°.
Hence, ∠BCA = 50°.
(iii) As sum of opposite angles in a cyclic quadrilateral = 180°.
⇒ ∠ADC + ∠ABC = 180°
⇒ 80° + ∠ABC = 180°
⇒ ∠ABC = 180° - 80° = 100°.
Hence, ∠ABC = 100°.
(iv) From part (ii) we get,
Hence, ∠ADC = 80°.
Answered By
5 Likes
Related Questions
ABCD is a cyclic quadrilateral. Sides AB and DC produced meet at point E; whereas sides BC and AD produced meet at point F. If ∠DCF : ∠F : ∠E = 3 : 5 : 4, find the angles of the cyclic quadrilateral ABCD.
In the given figure, AB is the diameter of a circle with center O. If chord AC = chord AD, prove that :
(i) arc BC = arc DB
(ii) AB is the bisector of ∠CAD.
Further, if the length of arc AC is twice the length of arc BC, find :
(a) ∠BAC
(b) ∠ABC

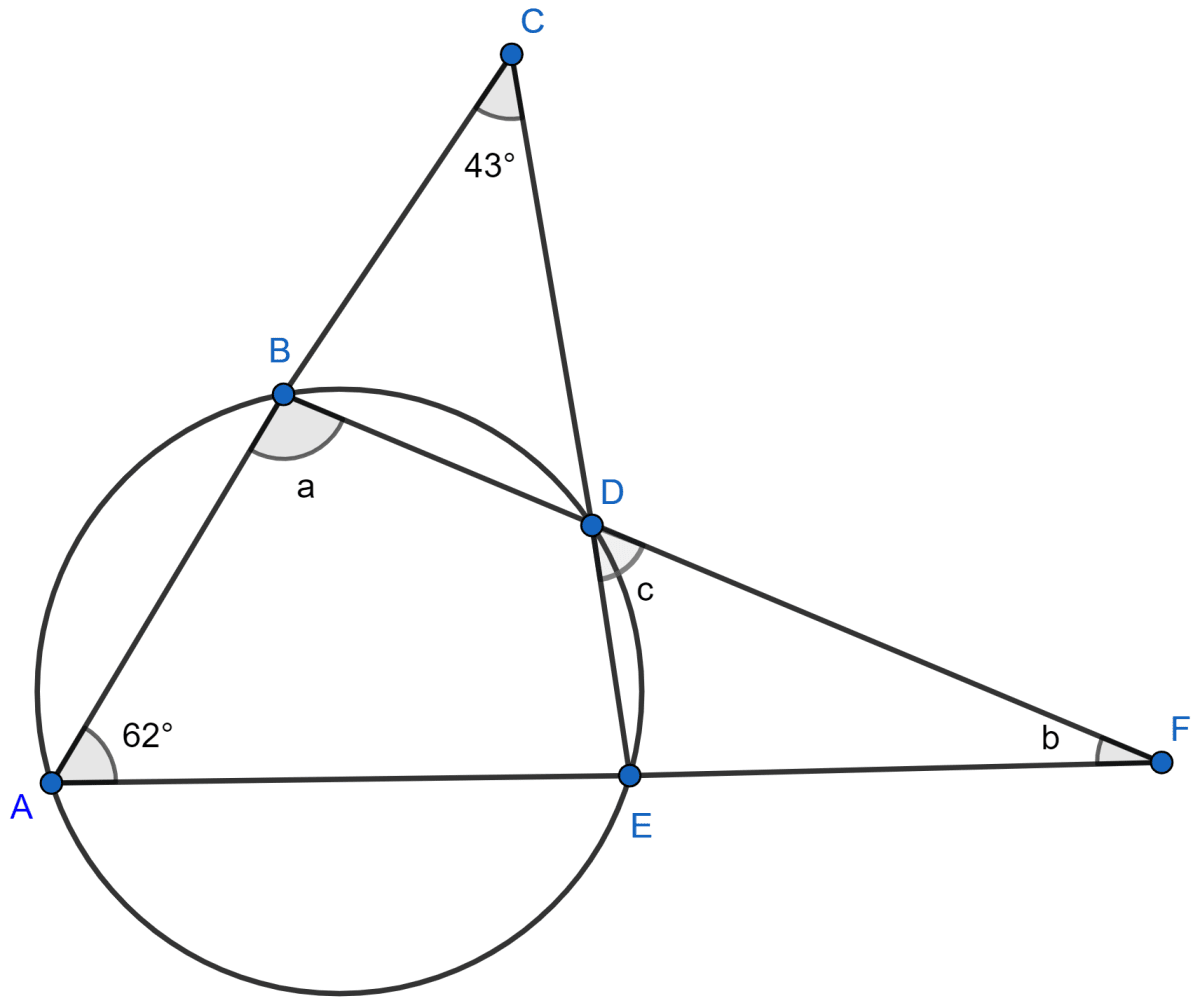
In the given figure, ∠ACE = 43° and ∠CAF = 62°; find the values of a, b and c.
In the given figure, AB is parallel to DC, ∠BCE = 80° and ∠BAC = 25°.
Find :
(i) ∠CAD
(ii) ∠CBD
(iii) ∠ADC
