Mathematics
In a △ABC, ∠A = 90°, CA = AB and D is a point on AB produced. Prove that
DC2 - BD2 = 2AB × AD.
Answer
In right angle triangle ACD,
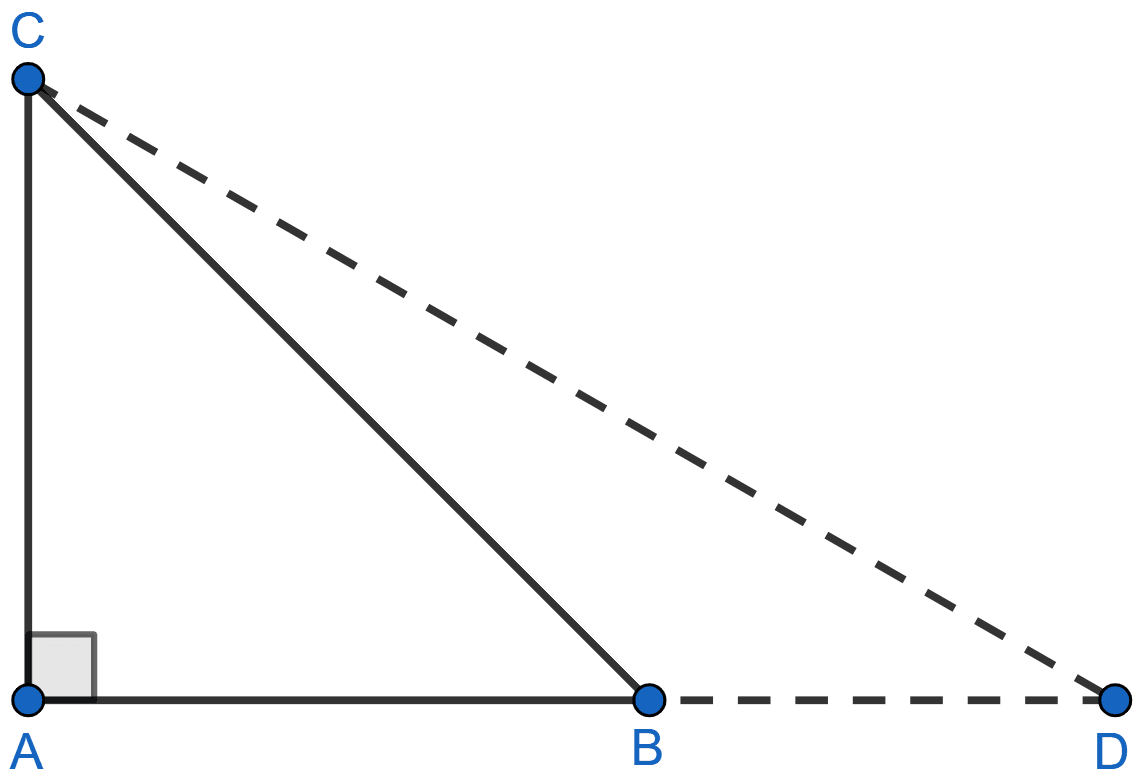
DC2 = CA2 + AD2 (Pythagoras theorem)
DC2 = CA2 + (AB + BD)2
DC2 = CA2 + AB2 + BD2 + 2AB.BD
DC2 - BD2 = AB2 + AB2 + 2AB.BD [∵ CA = AB]
DC2 - BD2 = 2AB2 + 2AB.BD
DC2 - BD2 = 2AB(AB + BD)
From figure, AB + BD = AD
DC2 - BD2 = 2AB.AD
Hence, proved that DC2 - BD2 = 2AB.AD.
Related Questions
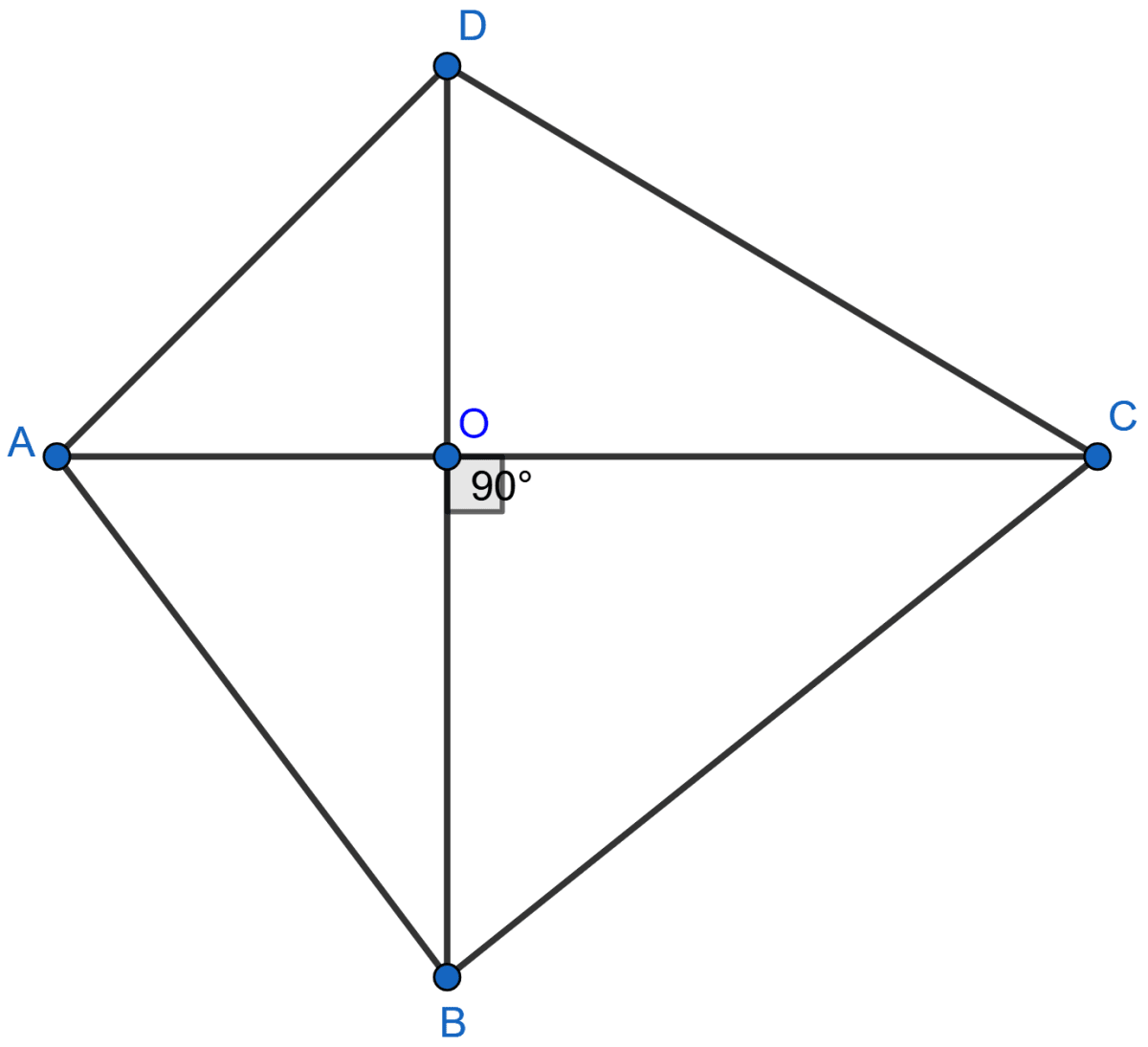
In the adjoining figure, the diagonals AC and BD of a quadrilateral ABCD intersect at O, at right angles. Prove that AB2 + CD2 = AD2 + BC2.
In an isosceles triangle ABC, AB = AC and D is a point on BC produced. Prove that AD2 = AC2 + BD × CD.
In a quadrilateral ABCD, ∠B = 90° = ∠D. Prove that
2AC2 - BC2 = AB2 + AD2 + DC2.
If AD, BE and CF are medians of △ABC, prove that
3(AB2 + BC2 + CA2) = 4(AD2 + BE2 + CF2).