KnowledgeBoat Logo

Mathematics

If the length of the shadow of a tower is 3\sqrt{3} times that of its height, then the angle of elevation of the sun is

  1. 15°

  2. 30°

  3. 45°

  4. 60°

Heights & Distances

4 Likes

Answer

Let the angle of elevation be θ and height of tower be h meters.

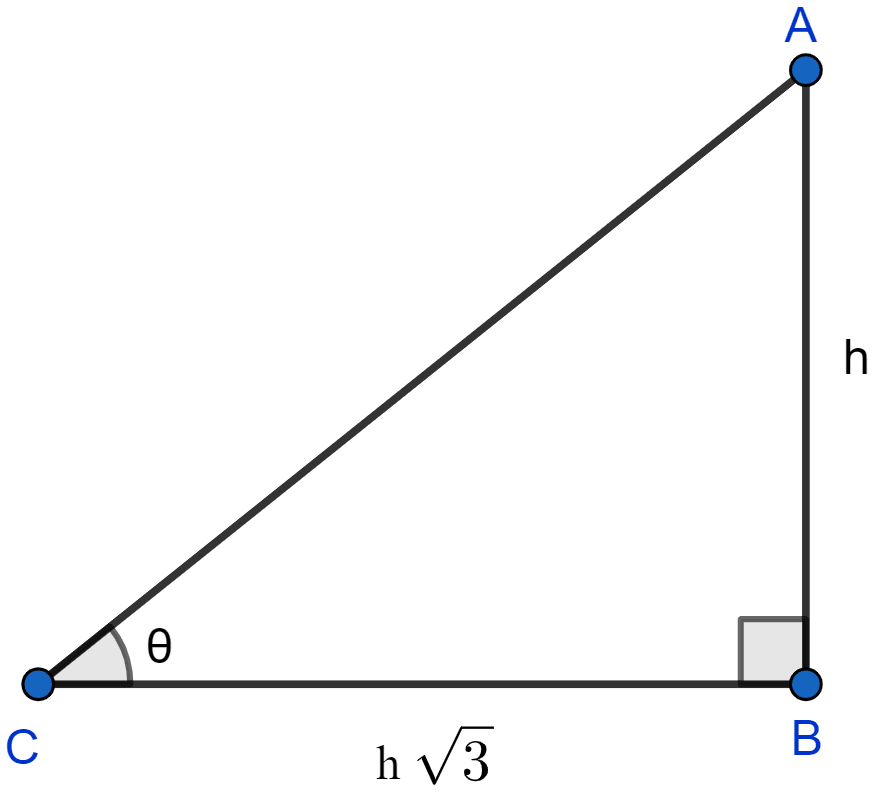
If the length of the shadow of a tower is √3 times that of its height, then the angle of elevation of the sun is. Heights and Distances, ML Aggarwal Understanding Mathematics Solutions ICSE Class 10.

So, shadow of tower = h3\sqrt{3} meters

Considering right angled △ABC we get,

⇒tan θ=ABBC⇒tan θ=hh3⇒tan θ=13⇒tan θ=tan 30°⇒θ=30°.\Rightarrow \text{tan θ} = \dfrac{AB}{BC} \\[1em] \Rightarrow \text{tan θ} = \dfrac{h}{h\sqrt{3}} \\[1em] \Rightarrow \text{tan θ} = \dfrac{1}{\sqrt{3}} \\[1em] \Rightarrow \text{tan θ} = \text{tan 30°} \\[1em] \Rightarrow θ = 30°.

Hence, Option 2 is the correct option.

Answered By

2 Likes

Related Questions