If the diagonals of a rhombus are equal, prove that it is a square.

From figure,

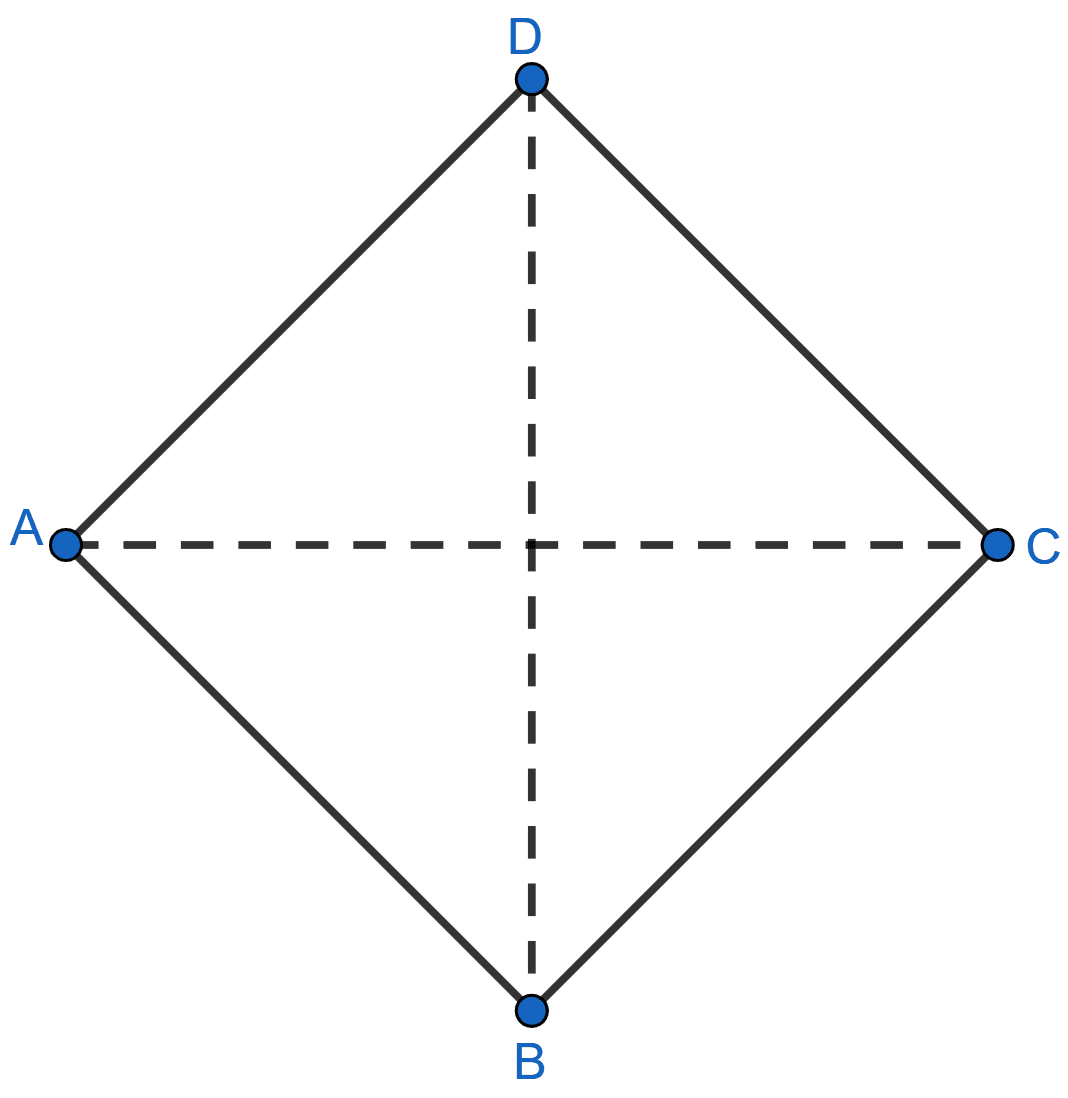
In △ABC and △BCD,

AB = DC (All sides of rhombus are equal)

BC = BC (Common sides)

AC = BD (Given diagonals are equal)

∴ △ABC ≅ △BCD (By SSS rule of congruency)

∠ABC = ∠BCD = x (let) (By C.P.C.T.)

∠ABC + ∠DCB = 180° [∵ AB || DC, sum of co-int ∠s = 180°]

⇒ x + x = 180°

⇒ 2x = 180°

x = $\dfrac{180°}{2}$

⇒ x = 90°.

∴ ∠ABC = ∠DCB = 90°.

Hence, proved that ABCD is a square.