Mathematics
If the areas of two similar triangles are 360 cm2 and 250 cm2 and if one side of the first triangle is 8 cm, find the length of the corresponding side of the second triangle.
Similarity
10 Likes
Answer
Let the corresponding side of the second triangle be x cm.
We know that, the ratio of the areas of two similar triangles is equal to the ratio of the square of their corresponding sides.
1}{\text{Area of } △2} = \dfrac{(\text{Side of } △1)^2}{(\text{Side of } △2)^2} \\[1em] \Rightarrow \dfrac{360}{250} = \dfrac{8^2}{x^2} \\[1em] \Rightarrow x^2 = \dfrac{64 \times 250}{360} \\[1em] \Rightarrow x^2 = \dfrac{16000}{360} \\[1em] \Rightarrow x^2 = \dfrac{1600}{36} \\[1em] \Rightarrow x = \sqrt{\dfrac{1600}{36}} \\[1em] \Rightarrow x = \dfrac{40}{6} \\[1em] \Rightarrow x = \dfrac{20}{3} = 6\dfrac{2}{3}.
Hence, the length of corresponding side of second triangle is cm.
Answered By
6 Likes
Related Questions
In a △ABC, D and E are points on the sides AB and AC respectively such that DE || BC. If AD = 2.4 cm, AE = 3.2 cm, DE = 2 cm and BC = 5 cm, find BD and CE.
In a △ABC, D and E are points on the sides AB and AC respectively such that AD = 5.7 cm, BD = 9.5 cm, AE = 3.3 cm and AC = 8.8 cm. Is DE || BC? Justify your answer.
In the adjoining figure, D is a point on BC such that ∠ABD = ∠CAD. If AB = 5 cm, AC = 3 cm and AD = 4 cm, find
(i) BC
(ii) DC
(iii) area of △ACD : area of △BCA

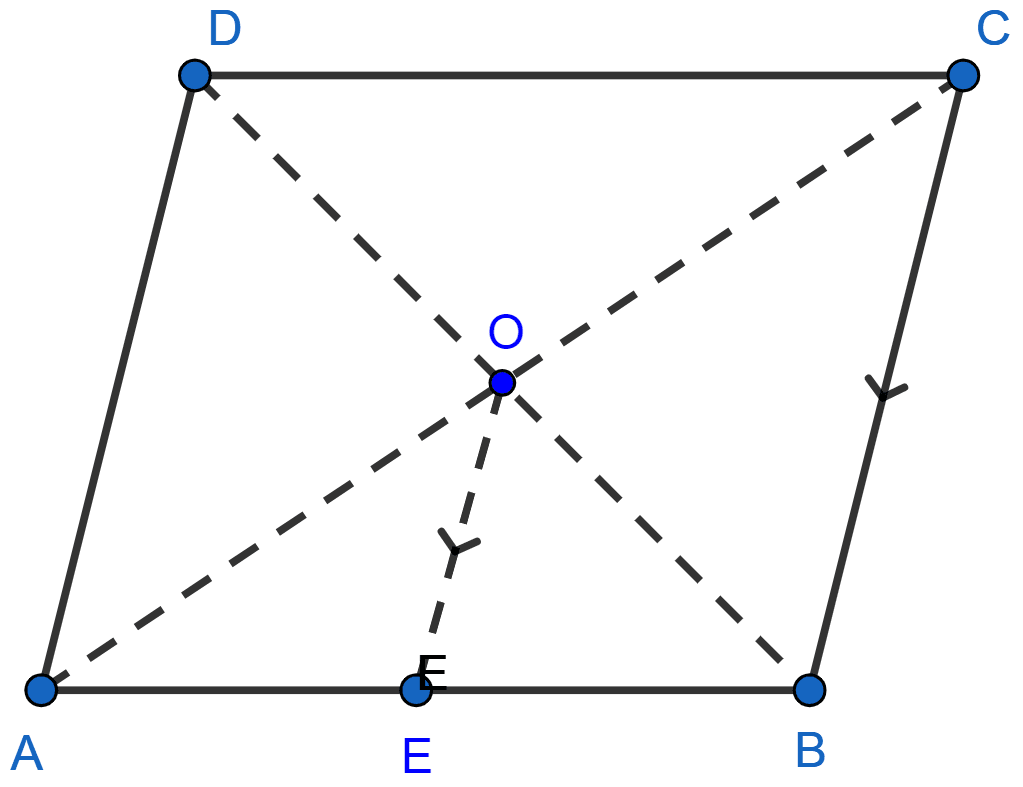
In the adjoining figure, the diagonals of a parallelogram intersect at O. OE is drawn parallel to CB to meet AB at E, find area of △AOE : area of ||gm ABCD.