Mathematics
If the area of an isosceles triangle is 60 cm2 and the length of each of its equal sides is 13 cm, find its base.
Mensuration
50 Likes
Answer
Let the length of equal sides be a cm and length of base be b cm.
Area of isosceles △ABC = , where a is length of equal sides and b is the length of other side.
Substituting values in above equation we get,
Squaring both sides we get,
Hence, the length of base = 10 cm or 24 cm.
Answered By
30 Likes
Related Questions
If the perimeter of a right angled triangle is 60 cm and its hypotenuse is 25 cm, find its area.
The perimeter of an isosceles triangle is 40 cm. The base is two third of the sum of equal sides. Find the length of each side.
The base of a triangular field is 3 times its height. If the cost of cultivating the field at the rate of ₹25 per 100 m2 is ₹60000, find its base and height.
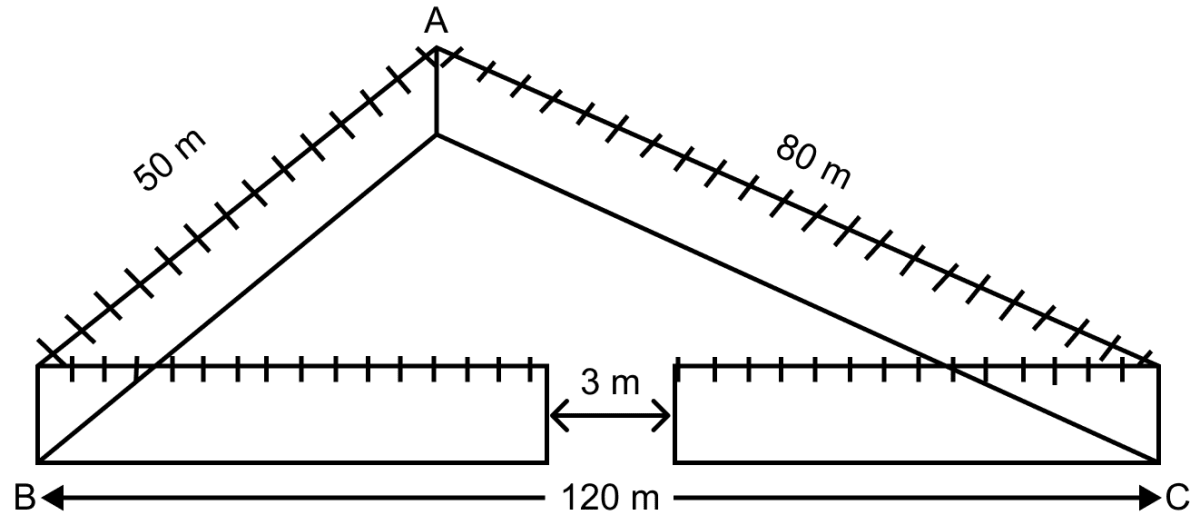
A triangular park ABC has sides 120 m, 80 m and 50 m (as shown in the adjoining figure). A gardner Dhania has to put a fence around it and also plant grass inside. How much area does she need to plant? Find the cost of fencing it with barbed wire at the rate of ₹ 20 per metre leaving a space 3 m wide for a gate on one side.