Mathematics
If a, b, c are the sides of a right angled triangle where c is the hypotenuse, prove that the radius r of the circle which touches the sides of the triangle is given by r = .
Circles
58 Likes
Answer
Let the circle touch the sides BC, CA and AB of the right triangle ABC at points D, E and F respectively,
where BC = a, CA = b and AB = c (as shown in the given figure).
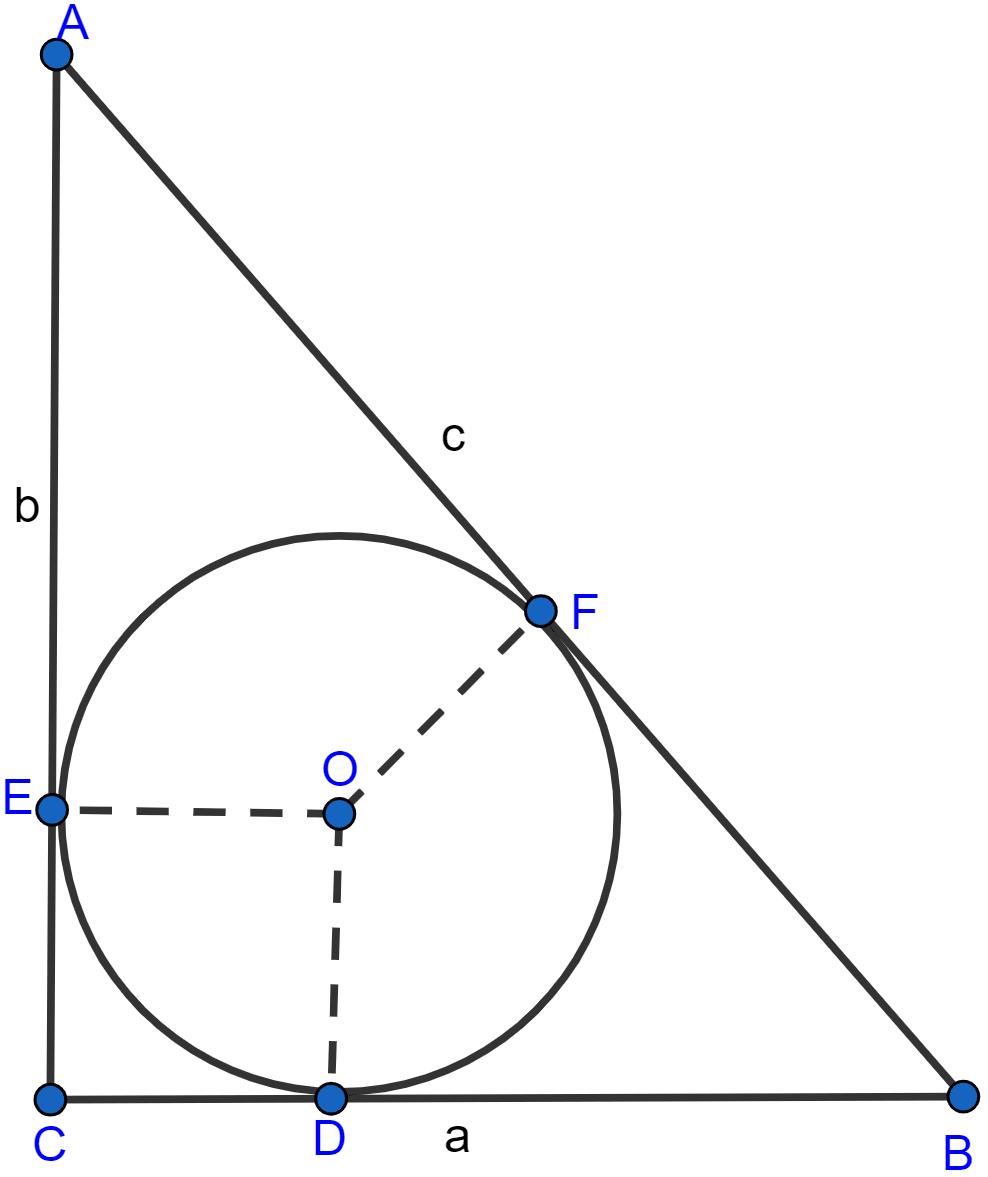
As the lengths of tangents drawn from an external point to a circle are equal
AE = AF, BD = BF and CD = CE
OD ⊥ BC and OE ⊥ CA (∵ tangents is ⊥ to radius)
ODCE is a square of side r
DC = CE = r
AF = AE = AC - EC = b - r and,
BF = BD = BC - DC = a - r
Now,
AB = AF + BF
⇒ c = (b - r) + (a - r)
⇒ c = b + a - 2r
⇒ 2r = a + b - c
⇒ r = .
Hence, proved that r =
Answered By
36 Likes
Related Questions
In figure (i) given below, O is the centre of the circle and AB is a tangent at B. If AB = 15 cm and AC = 7.5 cm, find the radius of the circle.

In the figure (ii) given below, from an external point P, tangents PA and PB are drawn to a circle. CE is a tangent to the circle at D. If AP = 15 cm, find the perimeter of the triangle PEC.

In the given figure, PB is a tangent to a circle with centre O at B. AB is a chord of length 24 cm at a distance of 5 cm from the centre. If the length of the tangent is 20 cm, find the length of OP.

Three circles of radii 2 cm, 3 cm and 4 cm touch each other externally. Find the perimeter of the triangle obtained on joining the centres of these circles.