Given O (0, 0), P(1, 2), S(-3, 0). P divides OQ in the ratio 2 : 3 and OPRS is a parallelogram.

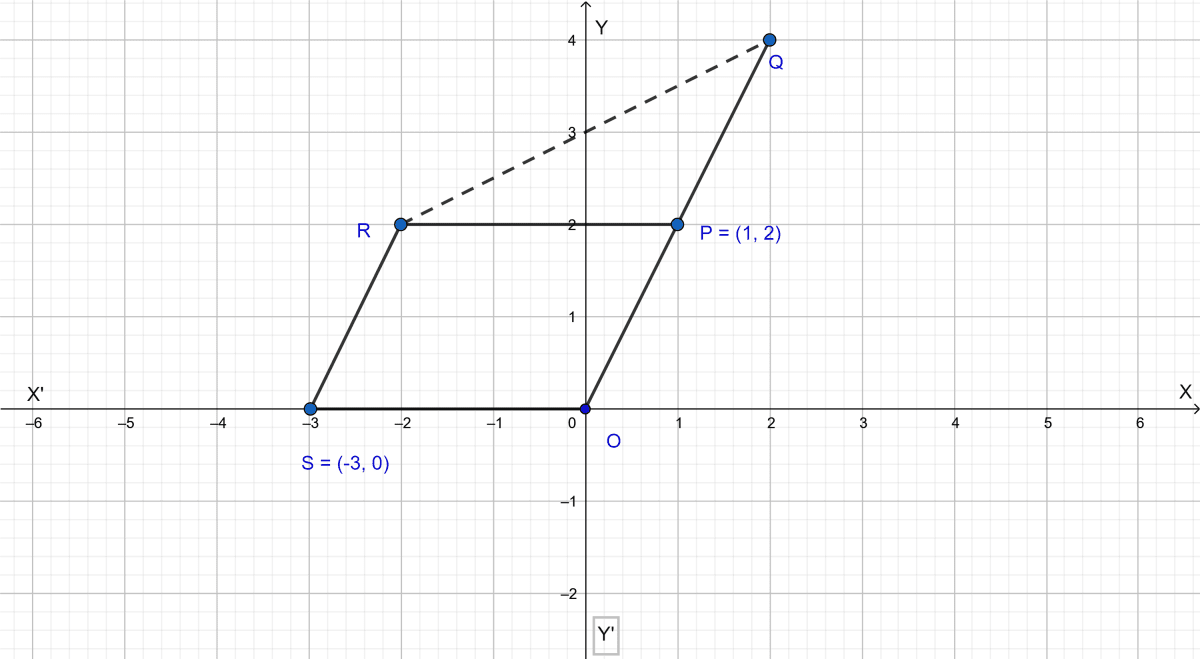
Find :

(i) the coordinates of Q.

(ii) the coordinates of R.

(iii) the ratio in which RQ is divided by the x-axis.

(i) Let coordinates of Q be (a, b).

Given, point P(1, 2) divides OQ in the ratio of 2 : 3. Here, O(0, 0) is the origin.

By section formula we get x,

x-coordinate = $\dfrac{m$1x2 + m2x1}{m1 + m2}

$\Rightarrow 1 = \dfrac{2 \times a + 3 \times 0}{2 + 3} \\[1em] \Rightarrow 1 = \dfrac{2a}{5} \\[1em] \Rightarrow a = \dfrac{5}{2}.$

By section formula we get y,

y-coordinate = $\dfrac{m$1y2 + m2y1}{m1 + m2}

$\Rightarrow 2 = \dfrac{2 \times b + 3 \times 0}{2 + 3} \\[1em] \Rightarrow 2 = \dfrac{2b}{5} \\[1em] \Rightarrow b = 5.$

Hence, coordinates of Q are $(\dfrac{5}{2}, 5)$.

(ii) In OPRS, OR and PS are diagonals. Let them bisect each other at point M which is the mid-point of both the diagonals. Let coordinates of R be (c, d).

Since, M is the midpoint of PS, by mid-point formula, coordinates of M

$= \Big(\dfrac{1 + (-3)}{2}, \dfrac{2 + 0}{2}\Big) \\[1em] = \Big(\dfrac{-2}{2}, \dfrac{2}{2}\Big) \\[1em] = (-1, 1).$

Since, M is the mid-point of OR also so,

$\Rightarrow -1 = \dfrac{0 + c}{2} \text{ and } 1 = \dfrac{0 + d}{2} \\[1em] \Rightarrow c = -2 \text{ and } d = 2.$

Hence, coordinates of R are (-2, 2).

(iii) Let the point on y-axis that divides RQ is N and it divides in ratio m₁ : m₂.

Since, N lies on y-axis so abscissa(x) = 0.

By section formula we get,

$\text{x-coordinate } = \dfrac{m$1x2 + m2x1}{m1 + m2} \\[1em] \Rightarrow 0 = \dfrac{m1 \times \dfrac{5}{2} + m2 \times (-2)}{m1 + m2} \\[1em] \Rightarrow \dfrac{5m1}{2} - 2m2 = 0 \\[1em] \Rightarrow \dfrac{5}{2}m1 = 2m2 \\[1em] \Rightarrow m1 : m2 = \dfrac{2 \times 2}{5} \\[1em] \Rightarrow m1 : m2 = 4 : 5.

Hence, RQ is divided in the ratio 4 : 5 by x-axis.