Mathematics
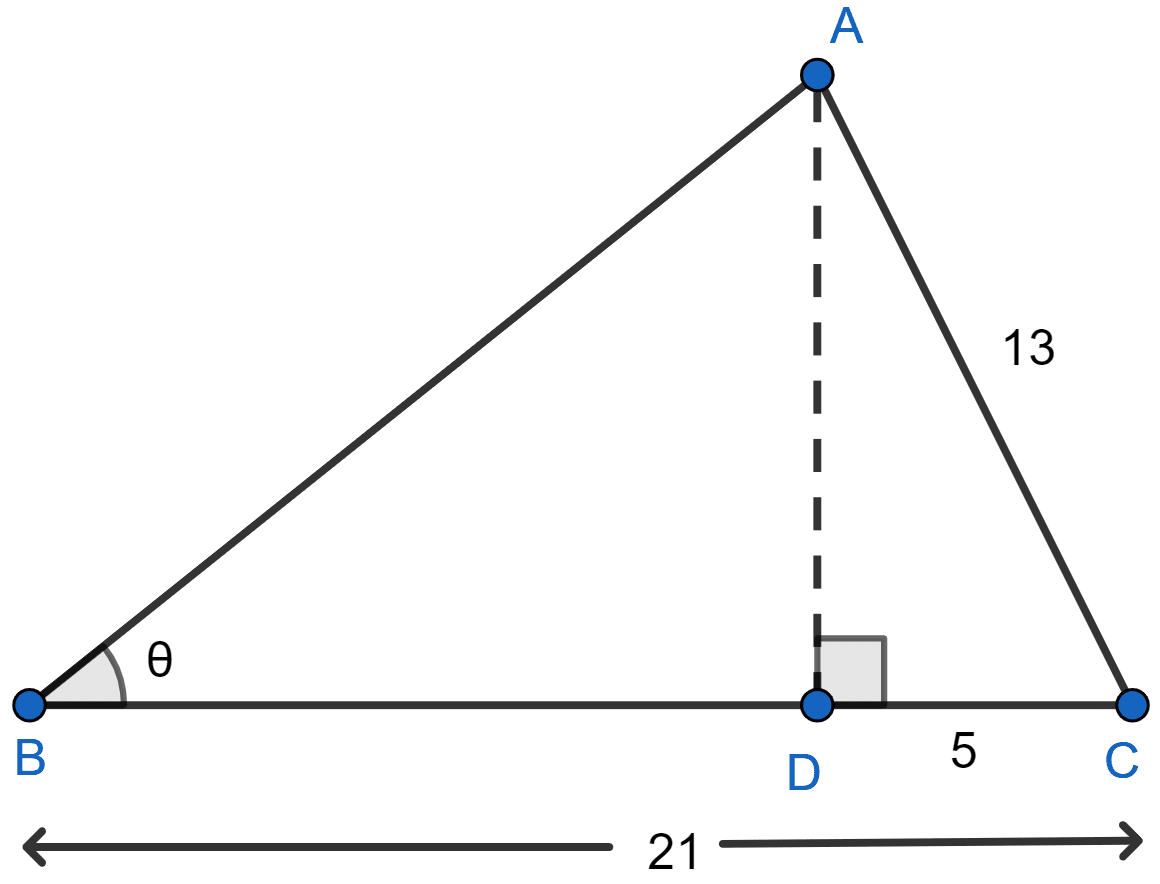
From the figure (1) given below, find the value of sec θ.
Trigonometrical Ratios
30 Likes
Answer
From figure,
⇒ BD = BC - CD = 21 - 5 = 16.
In △ADC,
⇒ AC2 = AD2 + DC2 [By pythagoras theorem]
⇒ AD2 = AC2 - DC2
⇒ AD2 = 132 - 52
⇒ AD2 = 169 - 25
⇒ AD2 = 144
⇒ AD =
⇒ AD = 12.
In △ABD,
⇒ AB2 = AD2 + BD2 [By pythagoras theorem]
⇒ AB2 = 122 + 162
⇒ AB2 = 144 + 256
⇒ AB2 = 400
⇒ AB =
⇒ AB = 20.
By formula,
sec θ =
= .
Hence, sec θ = .
Answered By
16 Likes
Related Questions
From the figure (1) given below, find the values of:
(i) sin B
(ii) cos C
(iii) sin B + sin C
(iv) sin B cos C + sin C cos B.

From the figure (2) given below, find the values of :
(i) tan x
(ii) cos y
(iii) cosec2 y - cot2 y
(iv) .
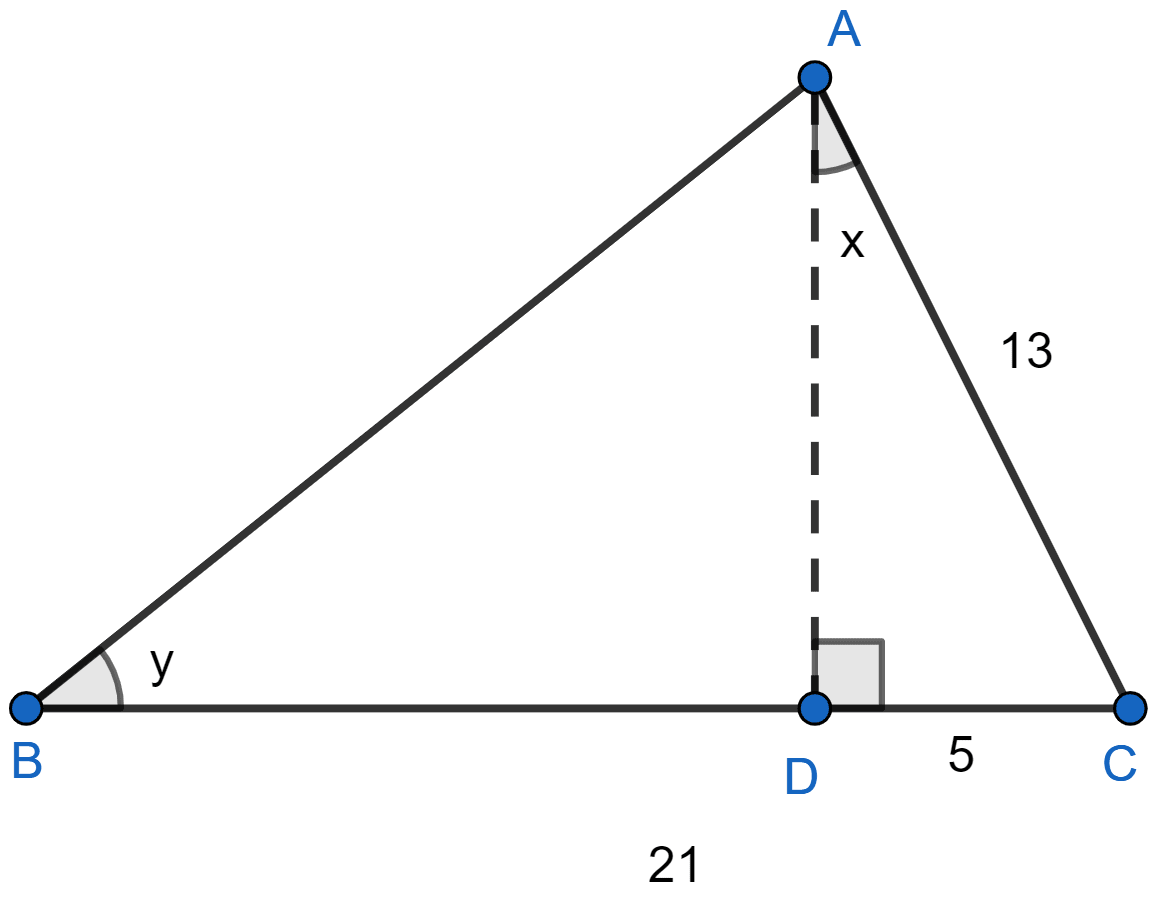
From the figure (2) given below, find the value of :
(i) sin x
(ii) cot x
(iii) cot2 x - cosec2 x
(iv) sec y
(v) tan2 y -
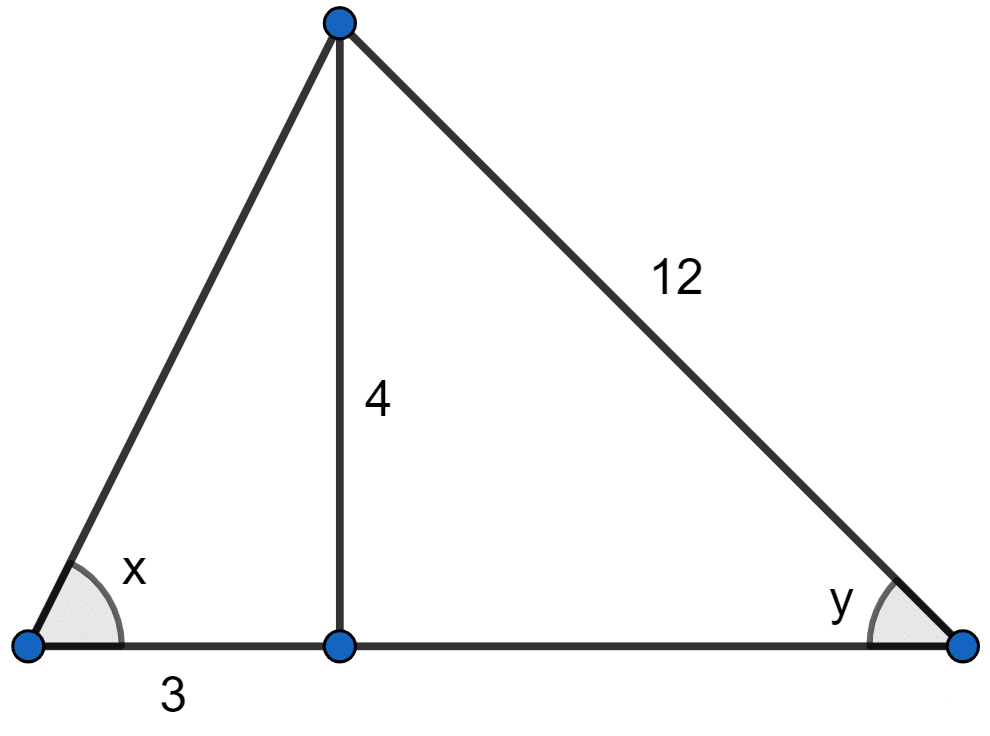
From the figure (1) given below, find the values of :
(i) 2 sin y - cos y
(ii) 2 sin x - cos x
(iii) 1 - sin x + cos y
(iv) 2 cos x - 3 sin y + 4 tan x
