Mathematics
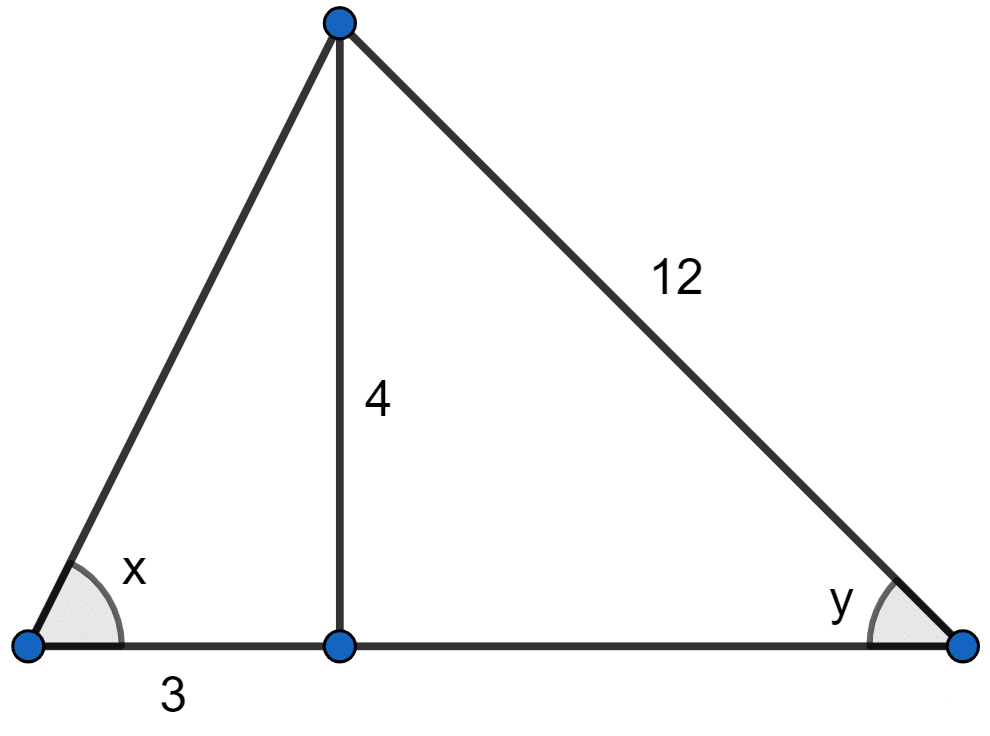
From the figure (2) given below, find the value of :
(i) sin x
(ii) cot x
(iii) cot2 x - cosec2 x
(iv) sec y
(v) tan2 y -
Trigonometrical Ratios
51 Likes
Answer
In right angled ∆ABD,
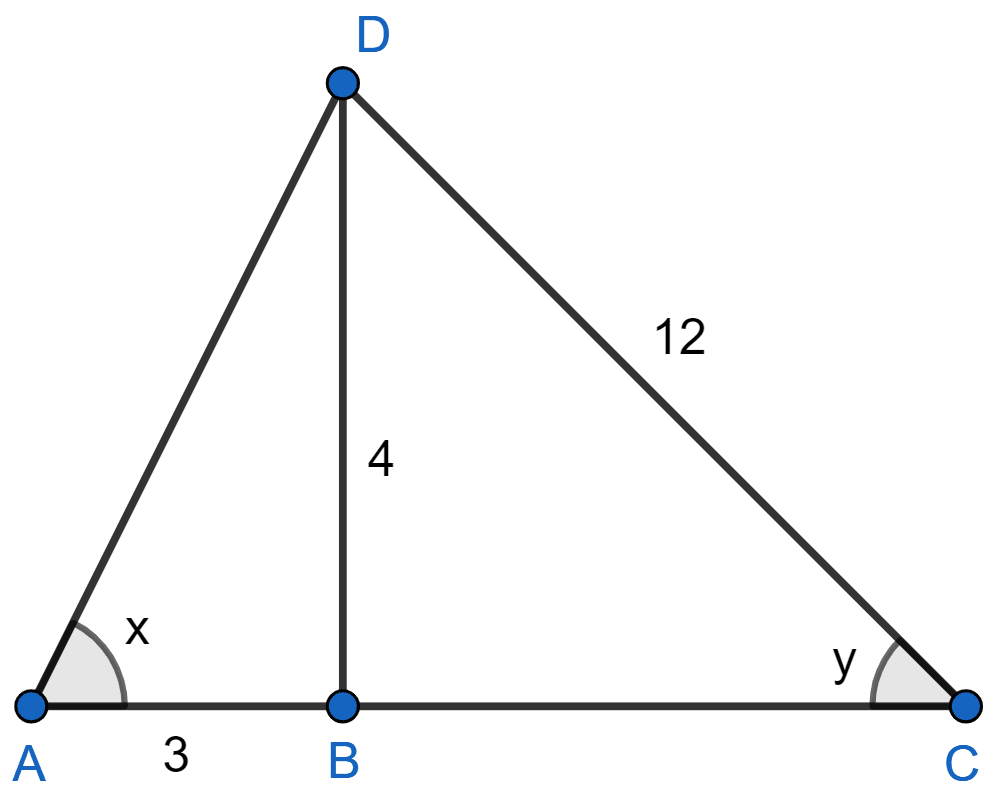
By pythagoras theorem we get,
⇒ AD2 = AB2 + BD2
⇒ AD2 = 32 + 42
⇒ AD2 = 9 + 16
⇒ AD2 = 25
⇒ AD =
⇒ AD = 5
In right angled triangle DBC,
By pythagoras theorem we get,
⇒ DC2 = DB2 + BC2
⇒ 122 = 42 + BC2
⇒ BC2 = 122 - 42
⇒ BC2 = 144 - 16
⇒ BC2 = 128
⇒ BC =
⇒ BC = .
(i) sin x =
= .
Hence, sin x = .
(ii) cot x =
= .
Hence, cot x = .
(iii) cosec x =
= .
Substituting values in cot2 x - cosec2 x we get :
Hence, cot2 x - cosec2 x = -1.
(iv) sec y =
= .
Hence, sec y = .
(v) tan y =
= .
Given,
Hence,
Answered By
31 Likes
Related Questions
From the figure (2) given below, find the values of :
(i) tan x
(ii) cos y
(iii) cosec2 y - cot2 y
(iv) .
From the figure (1) given below, find the value of sec θ.
From the figure (1) given below, find the values of :
(i) 2 sin y - cos y
(ii) 2 sin x - cos x
(iii) 1 - sin x + cos y
(iv) 2 cos x - 3 sin y + 4 tan x

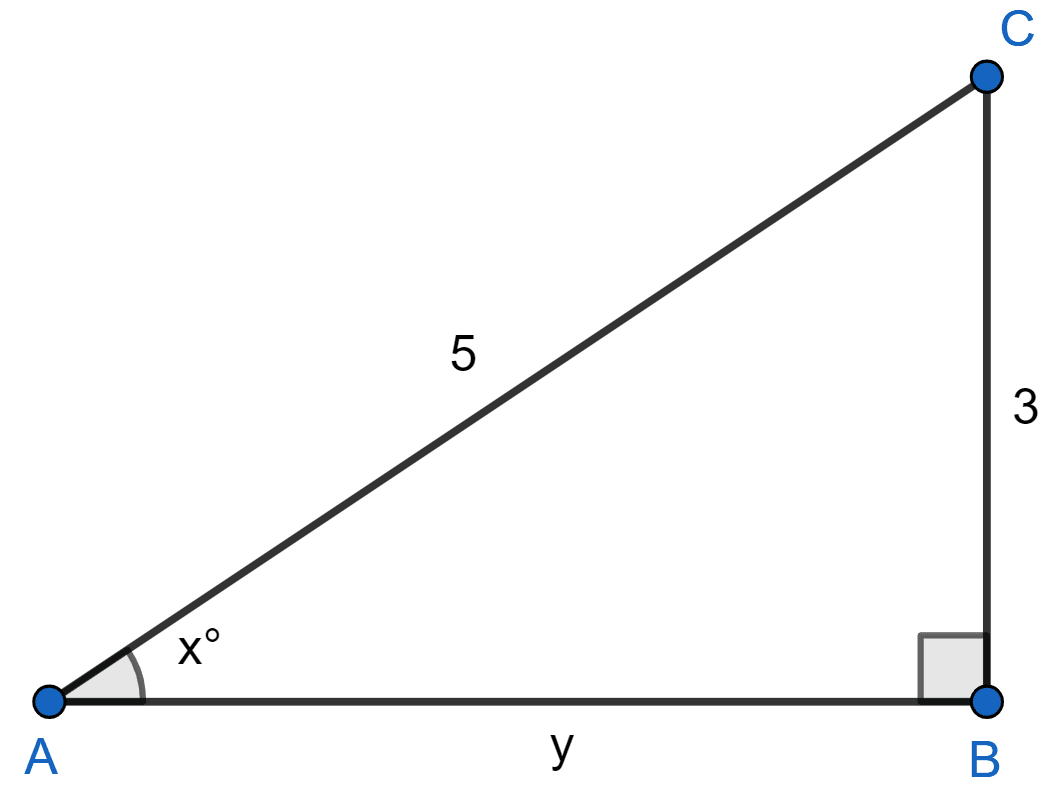
In the figure (2) given below, ∆ABC is right-angled at B. If AB = y units, BC = 3 units and CA = 5 units, find
(i) sin x°
(ii) y.