Mathematics
Answer
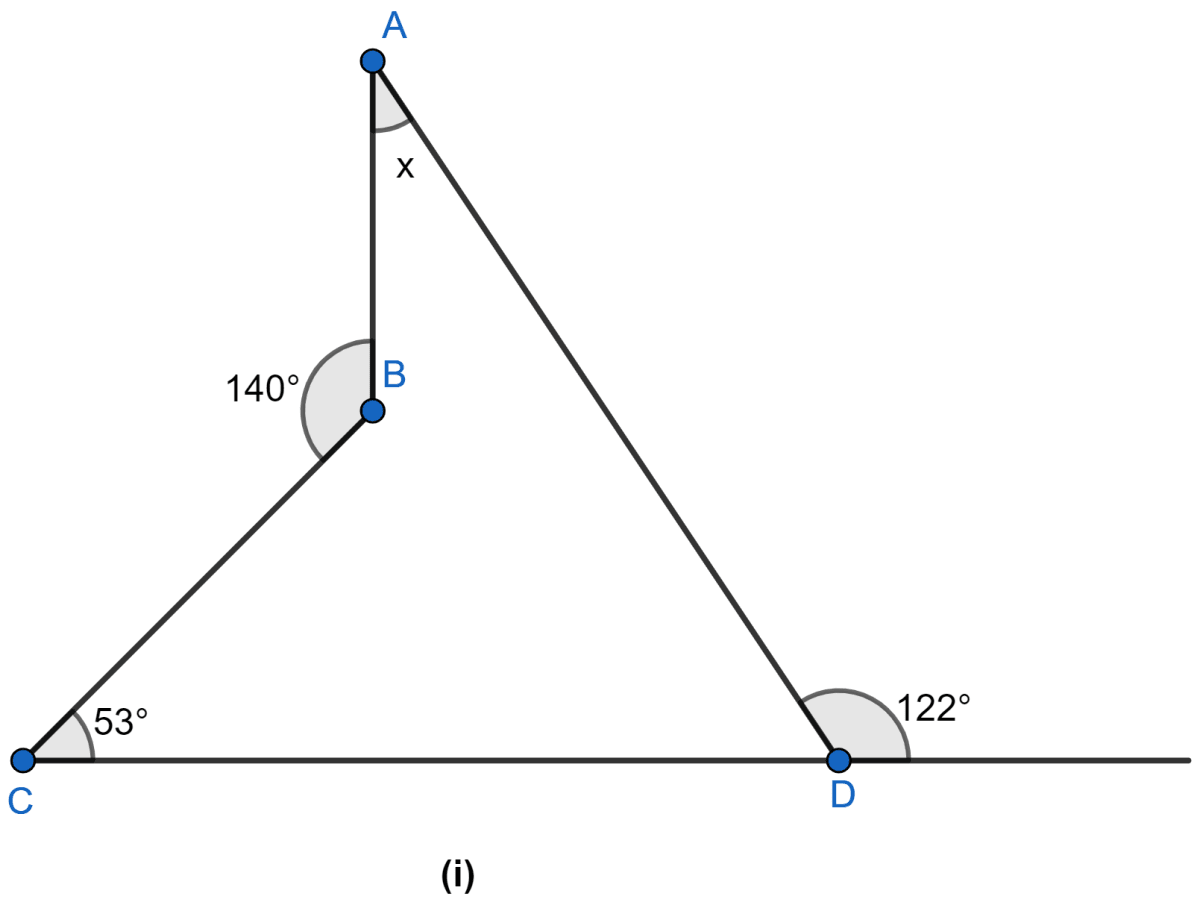
In △ADC,
AD = CD (Given)
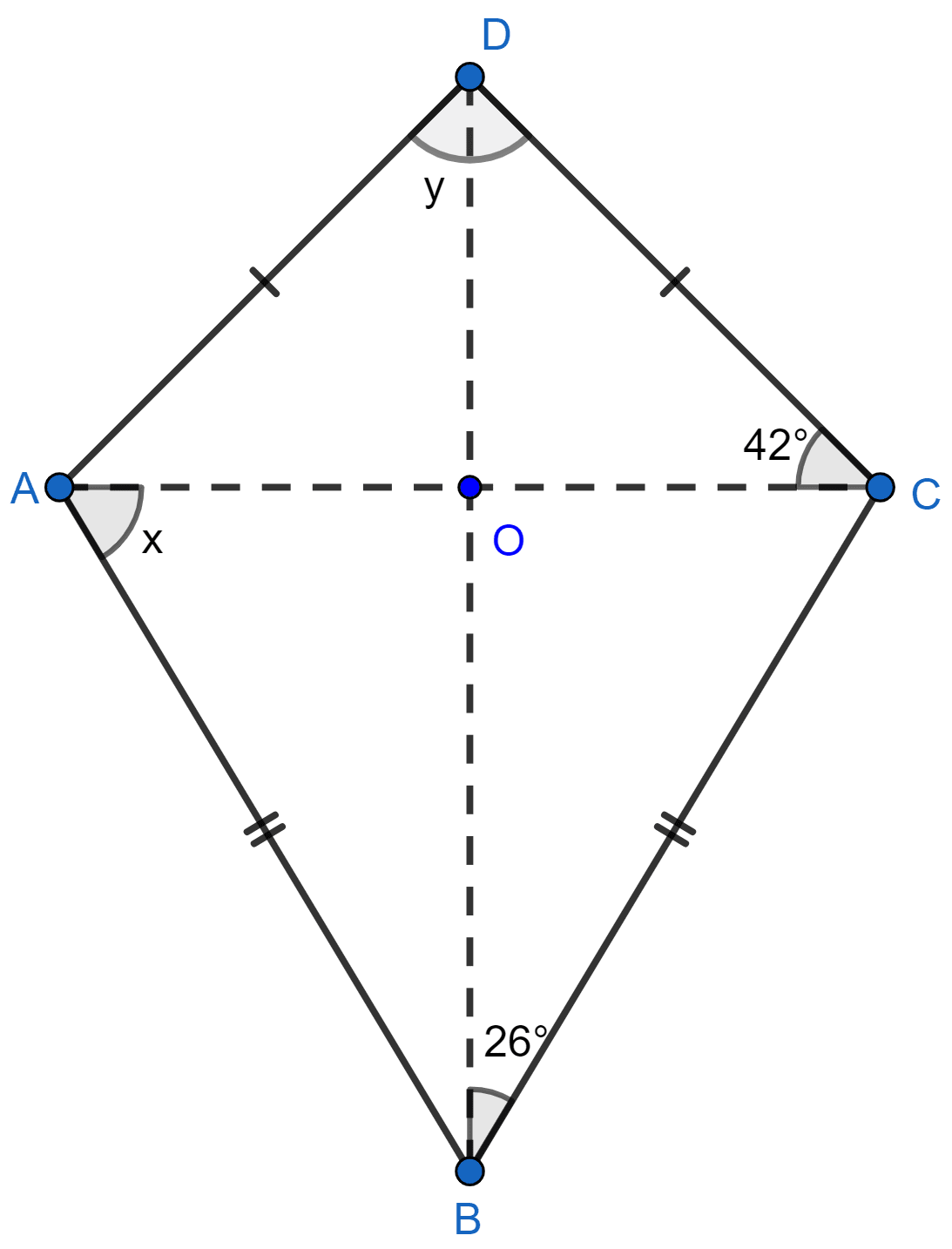
∠DAC = ∠DCA = 42° (Opposite angles of equal sides are equal).
⇒ ∠DAC + ∠DCA + ∠ADC = 180°
⇒ 42° + 42° + y = 180°
⇒ y + 84° = 180°
⇒ y = 96°
In △AOB and △COB,
AO = OC (Diagonals bisect each other)
AB = BC (Given)
∠AOB = ∠COB = 90° (Diagonals are perpendicular to each other)
Hence, △AOB ≅ △COB by RHS congruence rule.
By C.P.C.T. we get,
⇒ ∠ABO = ∠CBO = 26°
In △AOB,
⇒ ∠AOB + ∠ABO + ∠OAB = 180° (Sum of angles of triangle = 180°)
⇒ 90° + 26° + x = 180°
⇒ 116° + x = 180°
⇒ x = 180° - 116° = 64°
Hence, x = 64° and y = 96°.