Find the area of the quadrilateral ABCD in which ∠BCA = 90°, AB = 13 cm and ACD is an equilateral triangle of side 12 cm.

In right-angled △ABC,

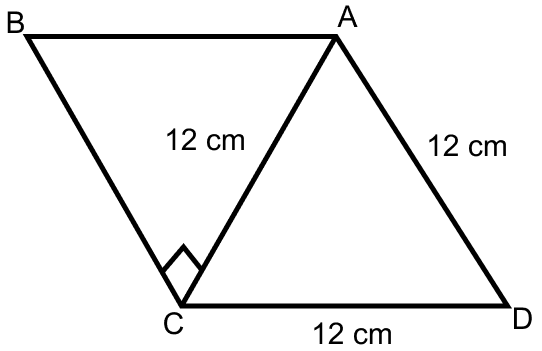
Using Pythagoras theorem,

⇒ AB² = AC² + BC²

Substituting the values we get,

⇒ 13² = 12² + BC²

⇒ BC² = 13² – 12²

⇒ BC² = 169 – 144 = 25

⇒ BC = $\sqrt{25}$ = 5 cm.

Calculating area of △BCA,

$\text{Area of △BCA } = \dfrac{1}{2} \times \text{ base × height} \\[1em] = \dfrac{1}{2} \times AC \times BC \\[1em] = \dfrac{1}{2} \times 12 \times 5 \\[1em] = 30 \text{ cm}^2.$

Calculating area of △ACD,

$\text{Area of △ACD } = \dfrac{\sqrt{3}}{4} \times \text{ (side)}^2 \\[1em] = \dfrac{\sqrt{3}}{4} \times (12)^2 \\[1em] = \dfrac{\sqrt{3}}{4} \times 144 \\[1em] = 36\sqrt{3} \\[1em] = 62.35 \text{ cm}^2 \\[1em]$

From figure,

Area of quadrilateral ABCD = Area of △BCA + Area of △ACD

= 30 cm² + 62.35 cm²

= 92.35 cm².

Hence, area of quadrilateral ABCD = 92.35 cm².