Mathematics
Draw two concentric circles with radii 4 cm and 6 cm. Taking a point on the outer circle, construct a pair of tangents to inner circle. By measuring the lengths of both the tangents, show that they are equal to each other.
Constructions
6 Likes
Answer
Steps of construction :
Draw two concentric circles of radius 4 cm and 6 cm with O as center.
Take a point P on the outer circle.
Join OP.
Draw perpendicular bisectors of OP, let it meet OP at point M.
With M as centre and OM as radius cut arcs at inner circle at point A and B.
Join PA and PB. Measure PA and PB.
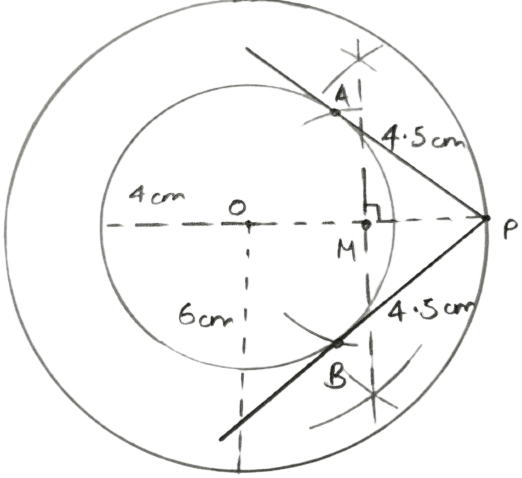
On measuring,
PA = PB = 4.5 cm.
Hence, PA and PB are tangents and length of each tangent = 4.5 cm.
Answered By
4 Likes
Related Questions
Using a ruler and a compass, construct a triangle ABC in which AB = 7 cm, ∠CAB = 60° and AC = 5 cm. Construct the locus of :
(i) points equidistant from AB and AC.
(ii) points equidistant from BA and BC.
Hence construct a circle touching the three sides of the triangle internally.
Construct a triangle ABC in which AB = 5 cm, BC = 6.8 cm and median AD = 4.4 cm. Draw incircle of this triangle.
In triangle ABC, ∠ABC = 90°, side AB = 6 cm, side BC = 7.2 cm and BD is perpendicular to side AC. Draw circumcircle of triangle BDC and then state the length of the radius of this circumcircle drawn.
Draw a line AB = 5 cm. Mark a point C on AB such that AC = 3 cm. Using a ruler and a compass only, construct :
(i) a circle of radius 2.5 cm, passing through A and C.
(ii) construct two tangents to the circle from the external point B. Measure and record the length of the tangents.