Mathematics
Draw the graph of 4x - 3y + 12 = 0 and use it to find the area of the triangle formed by the line and co-ordinate axes. Take 2 cm = 1 unit on both axes.
Coordinate Geometry
22 Likes
Answer
The above equation, 4x - 3y + 12 = 0 can be written as :
⇒ 3y = 4x + 12
⇒ y =
When x = -6, y = = -8 + 4 = -4,
x = -3, y = -4 + 4 = 0,
x = 0, y = 0 + 4 = 4.
Table of values :
| x | y |
|---|---|
| -6 | -4 |
| -3 | 0 |
| 0 | 4 |
Steps of construction :
Plot the points (-6, -4), (-3, 0) and (0, 4) on the graph.
Connect any two points by a straight line.
Observe that the third point lies on the straight line.
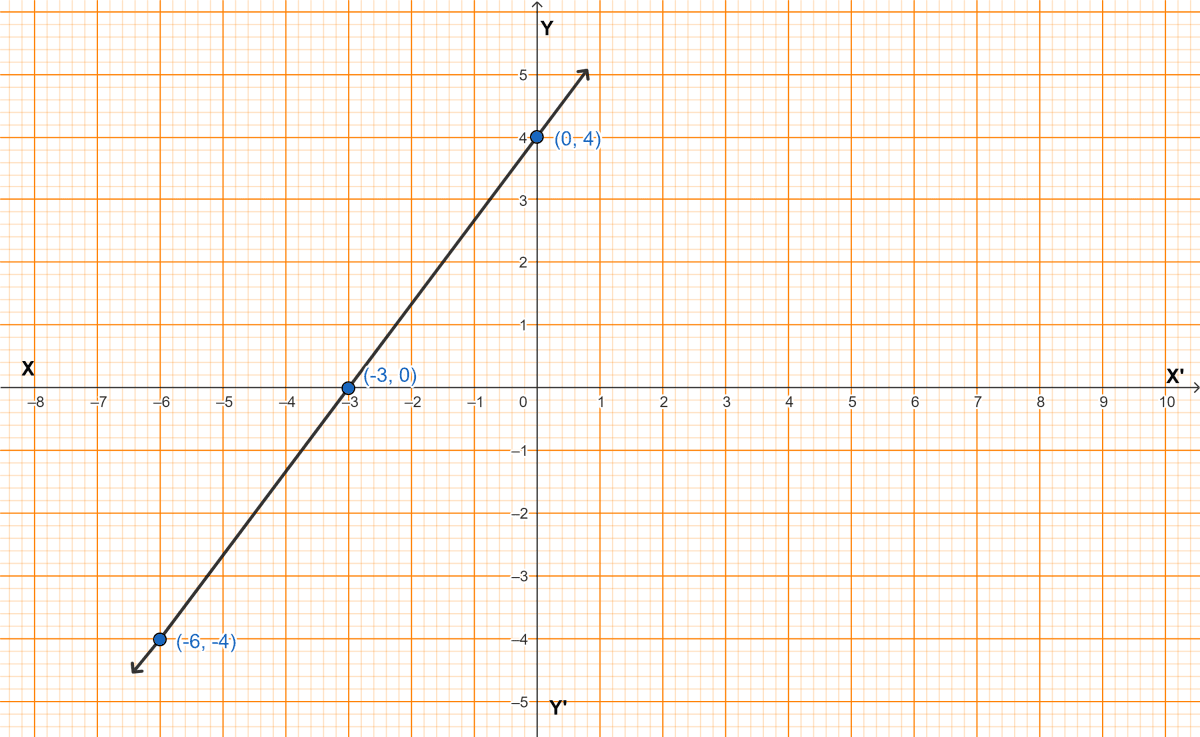
By formula,
Area of triangle =
From graph,
Base = 3 units, Height = 4 units.
Area = sq. units.
Hence, the graph of the given equation is shown in the adjoining figure and area of triangle = 6 sq. units.
Answered By
9 Likes
Related Questions
Draw the graph of 5x + 6y - 30 = 0 and use it to find the area of the triangle formed by the line and coordinate axes.
Draw the graph of 3y = 12 - 2x. Take 2 cm = 1 unit on both axes.
Draw the graph of the equation y = 3x - 4. Find graphically
(i) the value of y when x = -1
(ii) the value of x when y = 5.
The graph of a linear equation in x and y passes through (4, 0) and (0, 3). Find the value of k if the graph passes through (k, 1.5).