Mathematics
Diagonals AC and BD of a trapezium ABCD with AB || DC intersect each other at the point O. Using a similarity criterion for two triangles, show that .
Triangles
2 Likes
Answer
Trapezium ABCD is shown in the figure below:
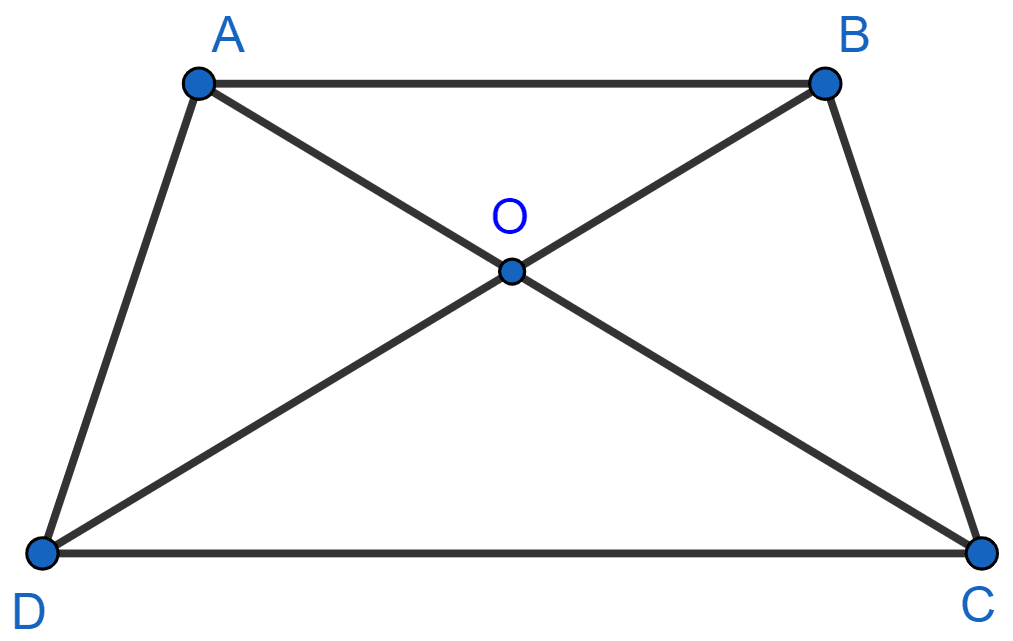
In Δ AOB and Δ COD,
∠AOB = ∠COD (Vertically opposite angles are equal)
∠BAO = ∠DCO (Alternate angles are equal)
⇒ Δ AOB ∼ Δ COD (By A.A. axiom)
We know that,
Ratio of corresponding sides of a similar triangle are proportional.
Hence, proved that .
Answered By
1 Like
Related Questions
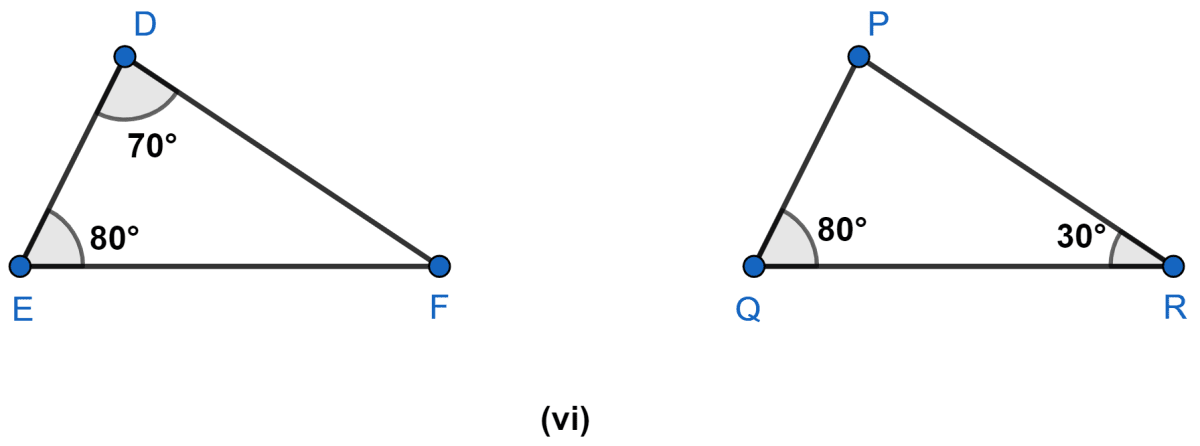
State which pairs of triangles are similar. Write the similarity criterion used by you for answering the question and also write the pairs of similar triangles in the symbolic form :
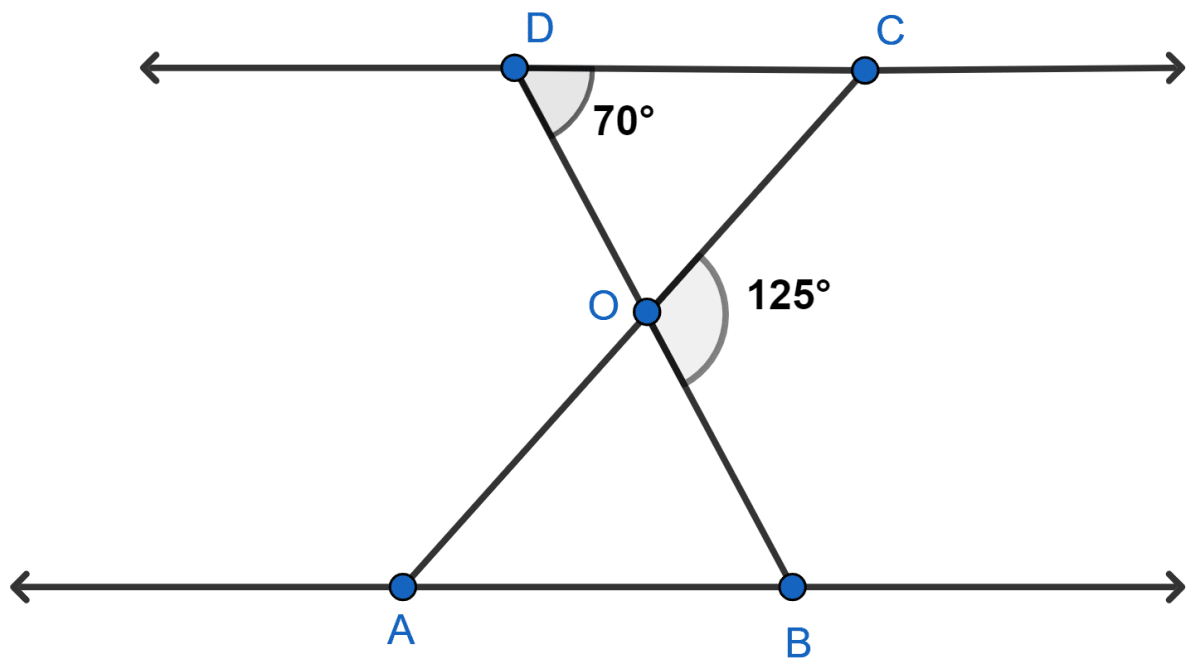
In given figure, △ ODC ~ △ OBA, ∠BOC = 125° and ∠CDO = 70°. Find ∠DOC, ∠DCO and ∠OAB.
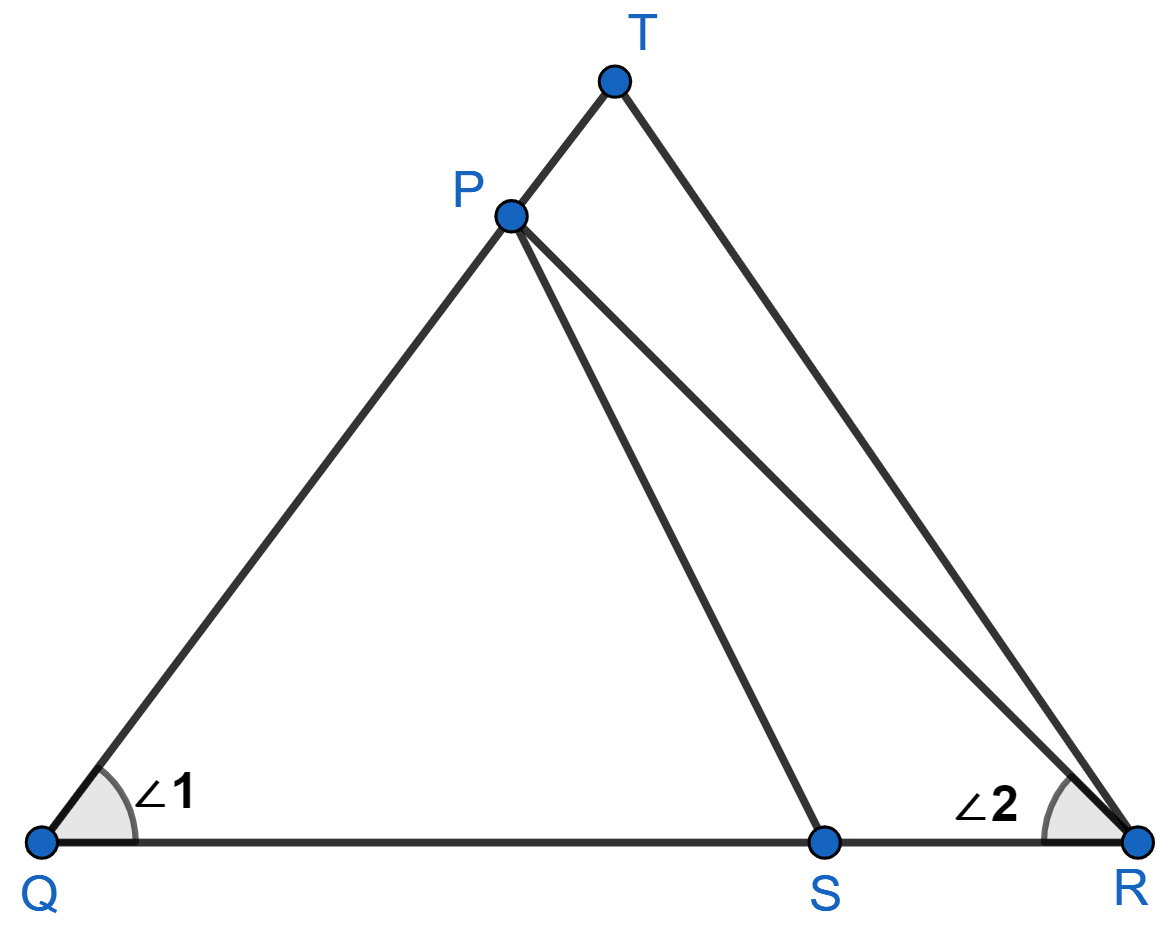
In the given figure, and ∠1 = ∠2. Show that △ PQS ~ △ TQR.
S and T are points on sides PR and QR of △ PQR such that ∠P = ∠RTS. Show that △ RPQ ~ △ RTS.