Construct a triangle ABC in which base BC = 5.5 cm, AB = 6 cm and ∠ABC = 120°.

(i) Construct a circle circumscribing the triangle ABC.

(ii) Draw a cyclic quadrilateral ABCD so that D is equidistant from B and C.

(i) Steps of construction :

1. Draw a line segment BC = 5.5 cm

2. From B draw a ray BX such that ∠XBC = 120°.

3. From B draw an arc of 6 cm cutting BX at A.

4. Join AC. ABC is the required triangle.

5. Construct perpendicular bisectors of AB and BC, such that they intersect at O.

6. With O as center and OA as radius draw a circle passing through A, B and C.

Hence, above is the required circumcircle of triangle ABC.

(ii) Steps of construction :

1. Draw perpendicular bisector of BC, such that it intersects the circle at D.

2. Join CD and AD. ABCD is the cyclic quadrilateral.

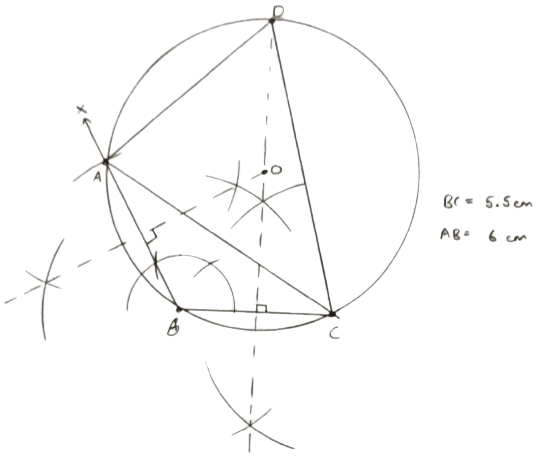
Hence, above is the required cyclic quadrilateral.