Mathematics
Construct a tangent to a circle of radius 4 cm from a point on the concentric circle of radius 6 cm and measure its length. Also verify the measurement by actual calculation.
Constructions
37 Likes
Answer
Steps of Construction :
Draw two concentric circles of radii 4 cm and 6 cm with point O as their centre.
Let P be a point on the outer circle. Join OP and draw its perpendicular bisector to meet OP at M.
Taking M as centre and OM (or MP) as radius, draw a circle. Let the circle intersect the smaller circle i.e. circle of radius 4 cm at points A and B.
Join PA and PB. Then PA and PB are the required tangents. On measuring, PA (or PB), we find that PA = 4.5 cm.
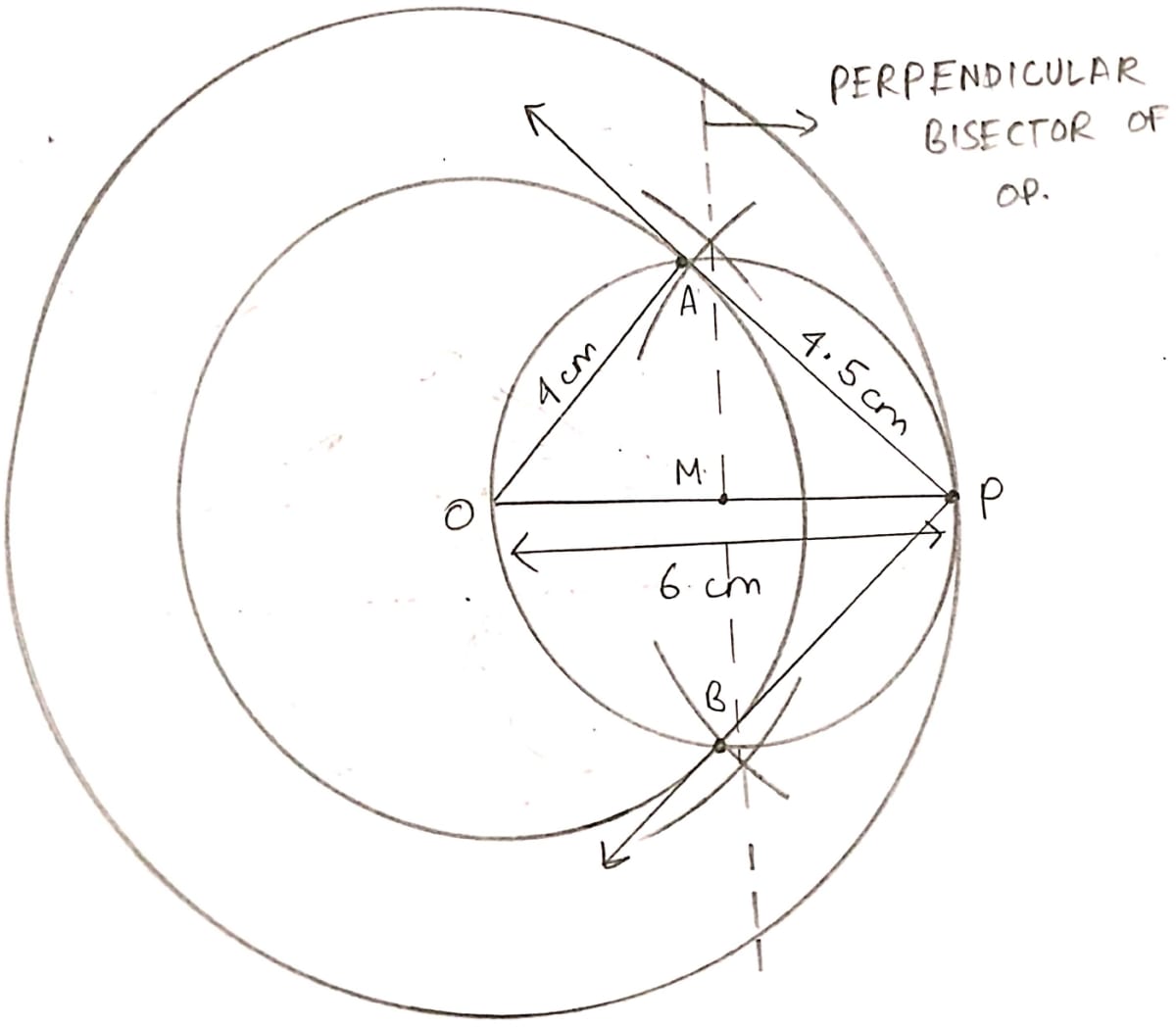
Calculation of length of PA
Join OA
In △OAP, ∠OAP = 90° (As angle in semicircle = 90°.)
By pythagoras theorem we get,
OA2 + PA2 = OP2
⇒ PA2 = OP2 - OA2
⇒ PA2 = 62 - 42 = 36 - 16 = 20 cm.
PA = = 4.5 cm.
Hence, the length of tangent = 4.5 cm.
Answered By
19 Likes
Related Questions
Use ruler and compass only for answering this question.
Draw a circle of radius 4 cm. Mark the centre as O. Mark a point P outside the circle at a distance of 7 cm from the centre. Construct two tangents to the circle from the external point P. Measure and write down the length of any one tangent.
Draw a line AB = 6 cm. Construct a circle with AB as diameter. Mark a point P at a distance of 5 cm from the mid-point of AB. Construct two tangents from P to the circle with AB as a diameter. Measure the length of each tangent.
Draw a circle of radius 3 cm. Take two points P and Q on one of its extended diameter each at a distance of 7 cm from its centre. Draw tangents to the circle from these two points P and Q.
Draw an equilateral triangle of side 4 cm. Draw its circumcircle.