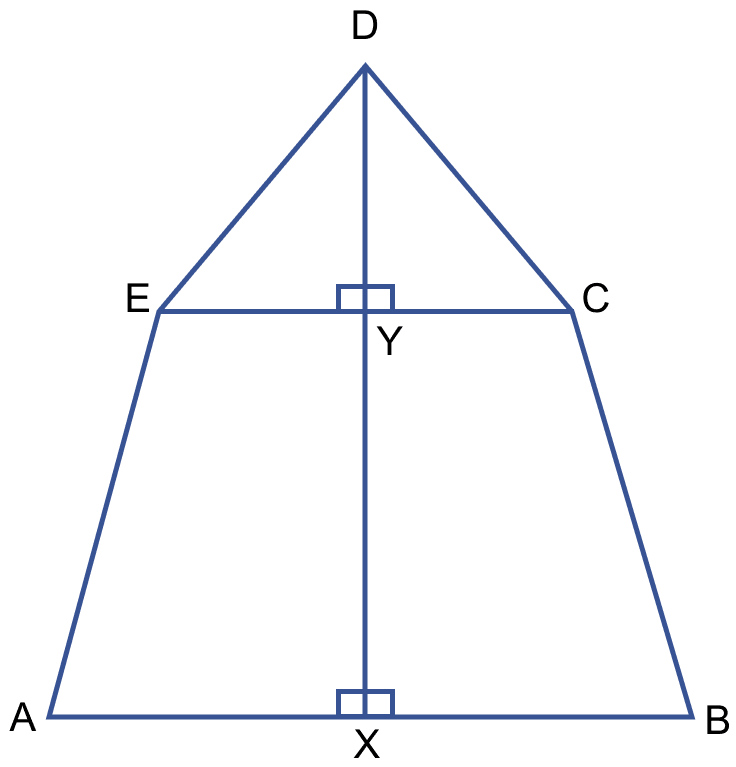
Calculate the area of the pentagon ABCDE shown in fig (iii) below, given that AX = BX = 6 cm, EY = CY = 4 cm, DE = DC = 5 cm, DX = 9 cm and DX is perpendicular to EC and AB.

From figure,

In right angled △DEY,

⇒ DE² = DY² + EY²

⇒ 5² = DY² + 4²

⇒ DY² = 5² - 4²

⇒ DY² = 25 - 16 = 9

⇒ DY = $\sqrt{9}$ = 3 cm.

Area of right angled △DEY = $\dfrac{1}{2}$ × base × height

= $\dfrac{1}{2}$ × EY × DY

= $\dfrac{1}{2}$ × 4 × 3

= 6 cm².

Area of right angle △DYC = $\dfrac{1}{2}$ × base × height

= $\dfrac{1}{2}$ × CY × DY

= $\dfrac{1}{2}$ × 4 × 3

= 6 cm².

From figure,

XY = DX - DY = 9 - 3 = 6 cm.

Area of trapezium ECBA = $\dfrac{1}{2} \times$ (sum of parallel sides) × distance between them

= $\dfrac{1}{2}$ × (EC + AB) × XY

= $\dfrac{1}{2}$ × [(EY + CY) + (AX + BX)] × XY

= $\dfrac{1}{2}$ × [(4 + 4) + (6 + 6)] × 6

= $\dfrac{1}{2}$ × 20 × 6

= 60 cm².

Area of pentagon = Area of right angled △DEY + Area of right angled △DYC + Area of trapezium ECBA

= 6 + 6 + 60

= 72 cm².

Hence, area of trapezium = 72 cm².