Mathematics
Calculate:
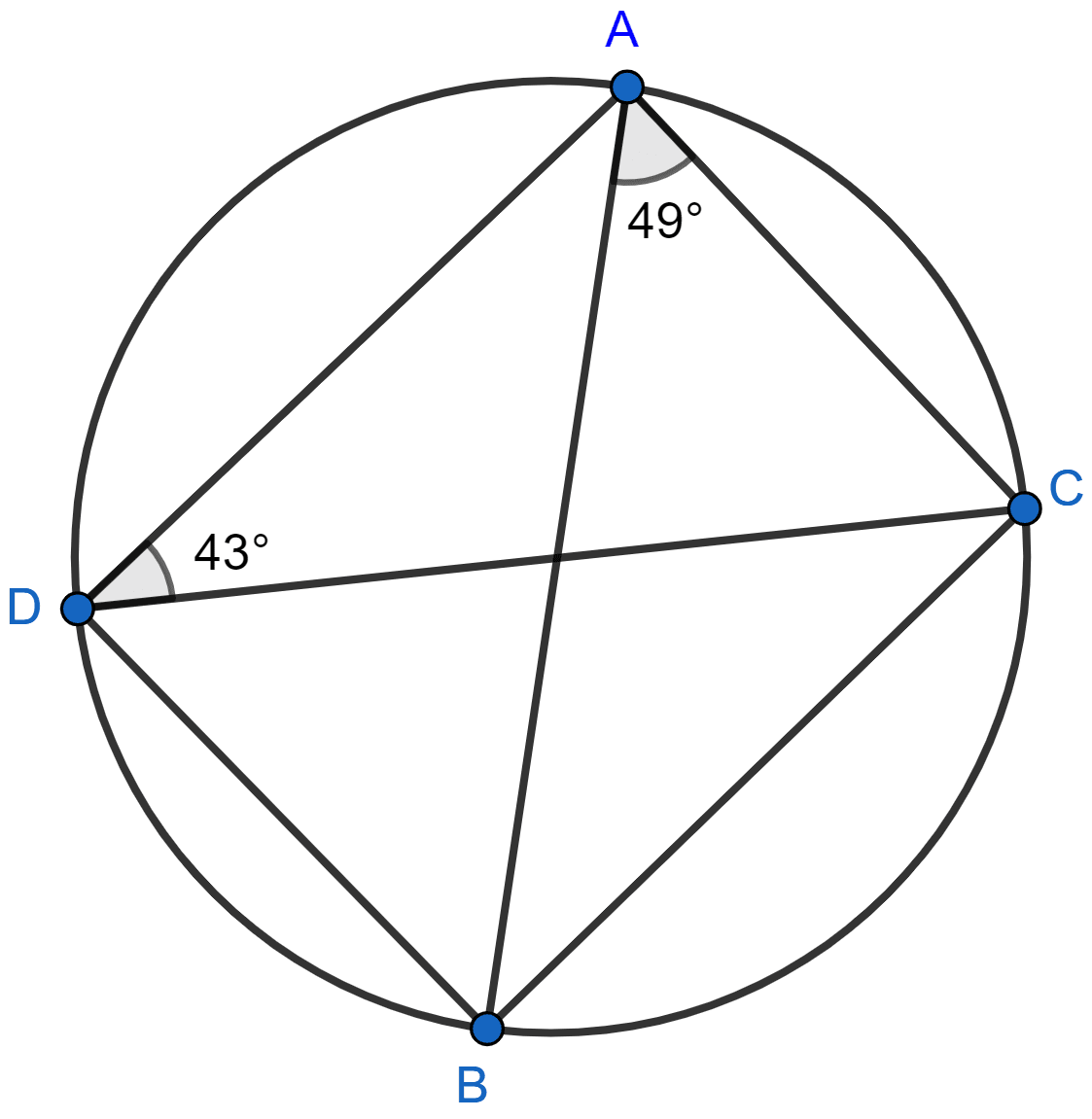
(i) ∠CDB,
(ii) ∠ABC,
(iii) ∠ACB.
Circles
31 Likes
Answer
(i) We know that,
Angles subtended by the same chord on the circle are equal.
∴ ∠CDB = ∠BAC = 49°.
Hence, ∠CDB = 49°.
(ii) We know that,
Angles subtended by the same chord on the circle are equal.
∠ABC = ∠ADC = 43°.
Hence, ∠ABC = 43°.
(iii) In △ABC,
⇒ ∠ABC + ∠ACB + ∠BAC = 180°
⇒ 43° + ∠ACB + 49° = 180°
⇒ ∠ACB + 92° = 180°
⇒ ∠ACB = 180° - 92° = 88°.
Hence, ∠ACB = 88°.
Answered By
21 Likes
Related Questions
In the following figure, O is the center of the circle. Find the value of c.

In the following figure, O is the center of the circle. Find the value of d.

Given: ∠CAB = 75° and ∠CBA = 50°. Find the value of ∠DAB + ∠ABD.

In the figure given alongside, AOB is a diameter of the circle and ∠AOC = 110°, find ∠BDC.
