Mathematics
At a point on level ground, the angle of elevation of a vertical tower is found to be such that its tangent is . On walking 192 m towards the tower, the tangent of the angle is found to be . Find the height of the tower.
Heights & Distances
27 Likes
Answer
Let the height of tower QR be h meters and the angle of elevation be θ1 and θ2 at points P and S respectively.
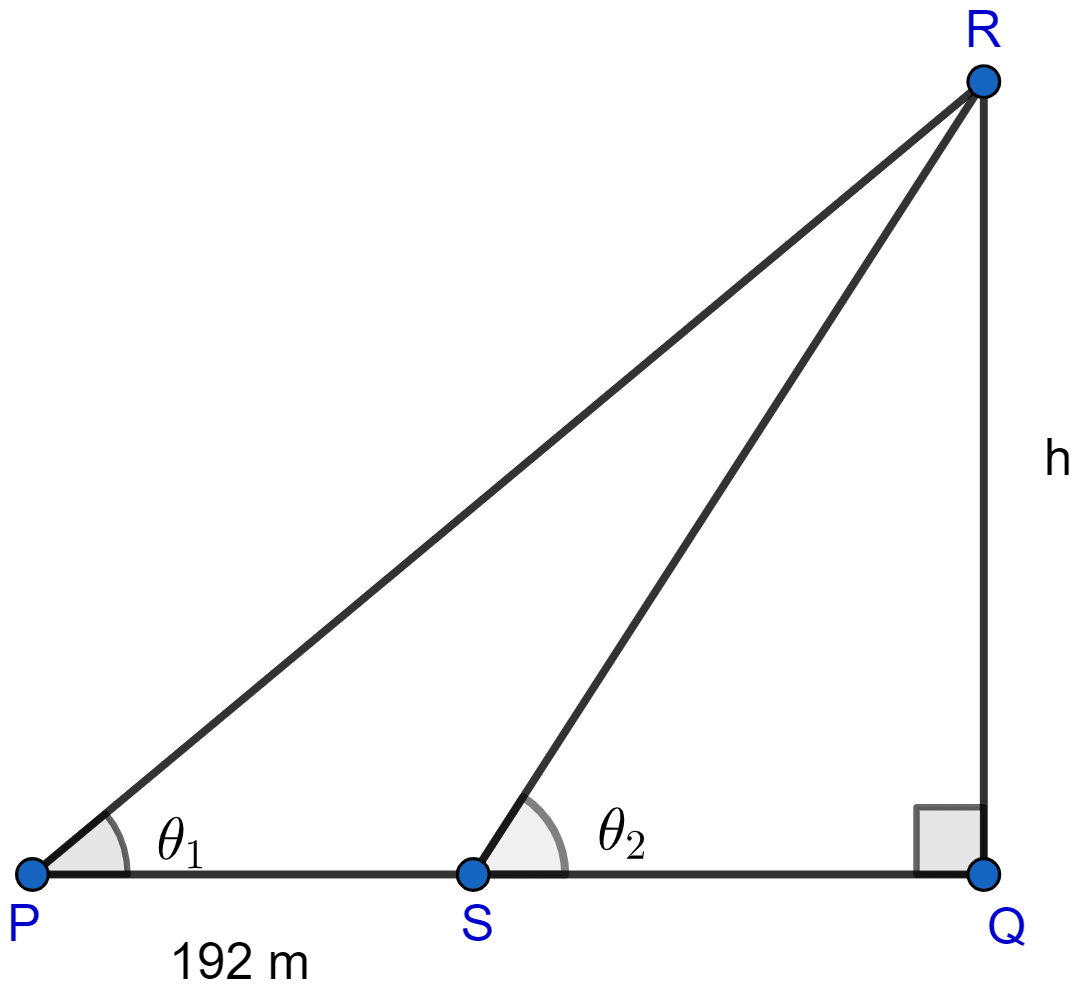
So,
From figure,
QR = h meters
PQ = PS + QS = (192 + QS) meters.
Considering right angled △PQR, we get
Considering right angled △SQR, we get
Putting value of QS from Eq 2 in Eq 1 we get,
Hence, the height of the tower is 180 meters.
Answered By
16 Likes
Related Questions
A man observes the angle of elevation of the top of a building to be 30°. He walks towards it in a horizontal line through its base. On covering 60 m, the angle of elevation changes to 60°. Find the height of the building correct to the nearest metre.
In the adjoining figure, not drawn to the scale, AB is a tower and two objects C and D are located on the ground, on the same side of AB. When observed from the top A of the tower, their angles of depression are 45° and 60°. Find the distance between the two objects, if the height of the tower is 300 m. Give your answer to the nearest meter.

From the top of a hill, the angles of depression of two consecutive kilometer stones, due east are found to be 30° and 45° respectively. Find the distance of two stones from the foot of the hill.
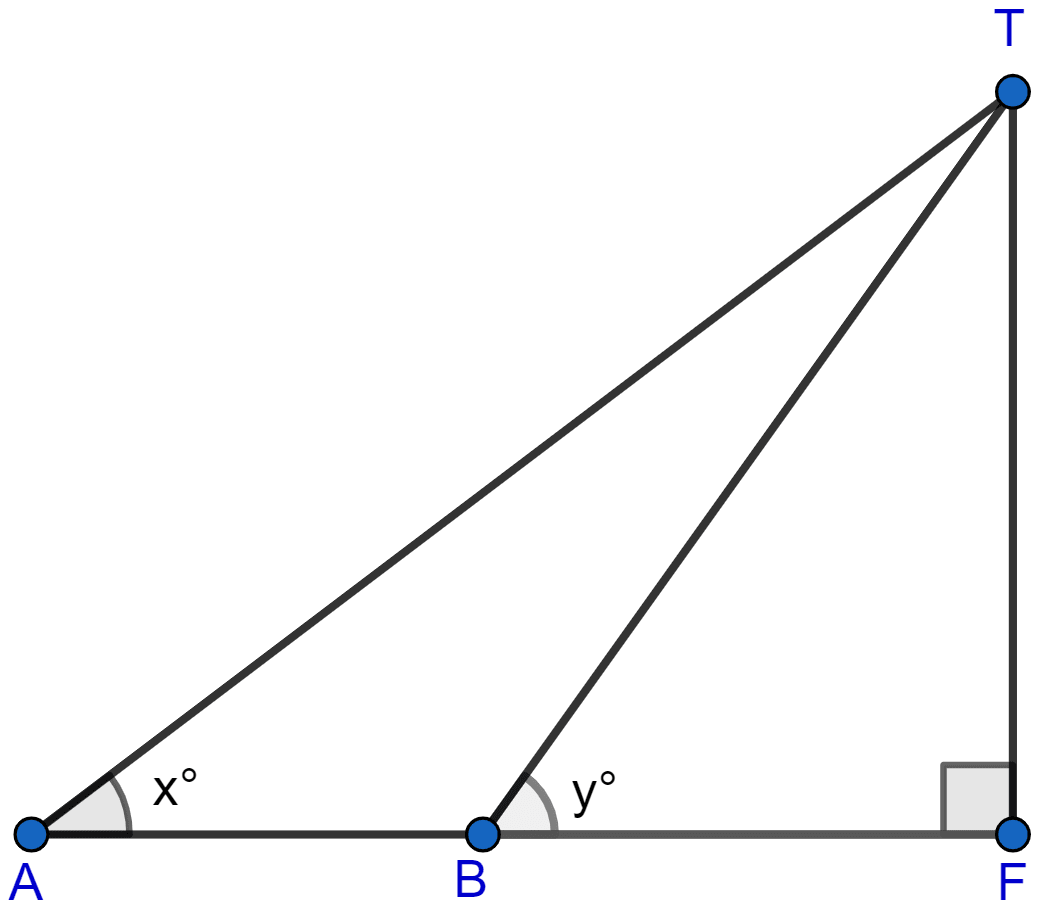
In the figure, not drawn to scale, TF is a tower. The elevation of T from A is x° where tan x = and AF = 200 m. The elevation of T from B, where AB = 80 m, is y°. Calculate :
(i) the height of the tower TF.
(ii) the angle y, correct to the nearest degree.