Mathematics

An exhibition tent is in the form of a cylinder surmounted by a cone. The height of the tent above ground is 85 m and height of the cylindrical part is 50 m. If the diameter of the base is 168 m, find the quantity of canvas required to make the tent. Allow 20% extra for fold and for stitching. Give your answer to the nearest m².

4 Likes

Given,

Diameter of base = 168 m

From figure,

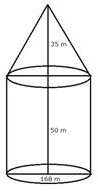
Diameter of cylindrical base = Diameter of cone

Radius of cylindrical base = Radius of conical base = r = $\dfrac{168}{2}$ = 84 m.

Height of cylindrical part (H) = 50 m

Height of conical part (h) = 35 m

By formula,

⇒ l² = r² + h²

⇒ l² = 84² + 35²

⇒ l² = 7056 + 1225

⇒ l² = 8281

⇒ l = $\sqrt{8281}$ = 91 m.

Surface area of exhibition tent = Surface area of cylindrical part + Surface area of conical part

= 2πrH + πrl

$= 2 \times \dfrac{22}{7} \times 84 \times 50 + \dfrac{22}{7} \times 84 \times 91 \\[1em] = 26400 + 24024 \\[1em] = 50424 \text{ m}^2.$

Area required for folds and stitching = $\dfrac{20}{100} \times 50424$ = 10084.8 m².

Total area of canvas required = 50424 + 10084.8 = 60508.8 m² ≈ 60509 m² (to the nearest m²).

Hence, area of canvas required = 60509 m².

Answered By

2 Likes