Mathematics
ABCD is a rhombus with P, Q and R as mid-points of AB, BC and CD respectively. Prove that PQ ⊥ QR.
Mid-point Theorem
37 Likes
Answer
Join AC and BD.
Diagonals of rhombus intersect at right angle.
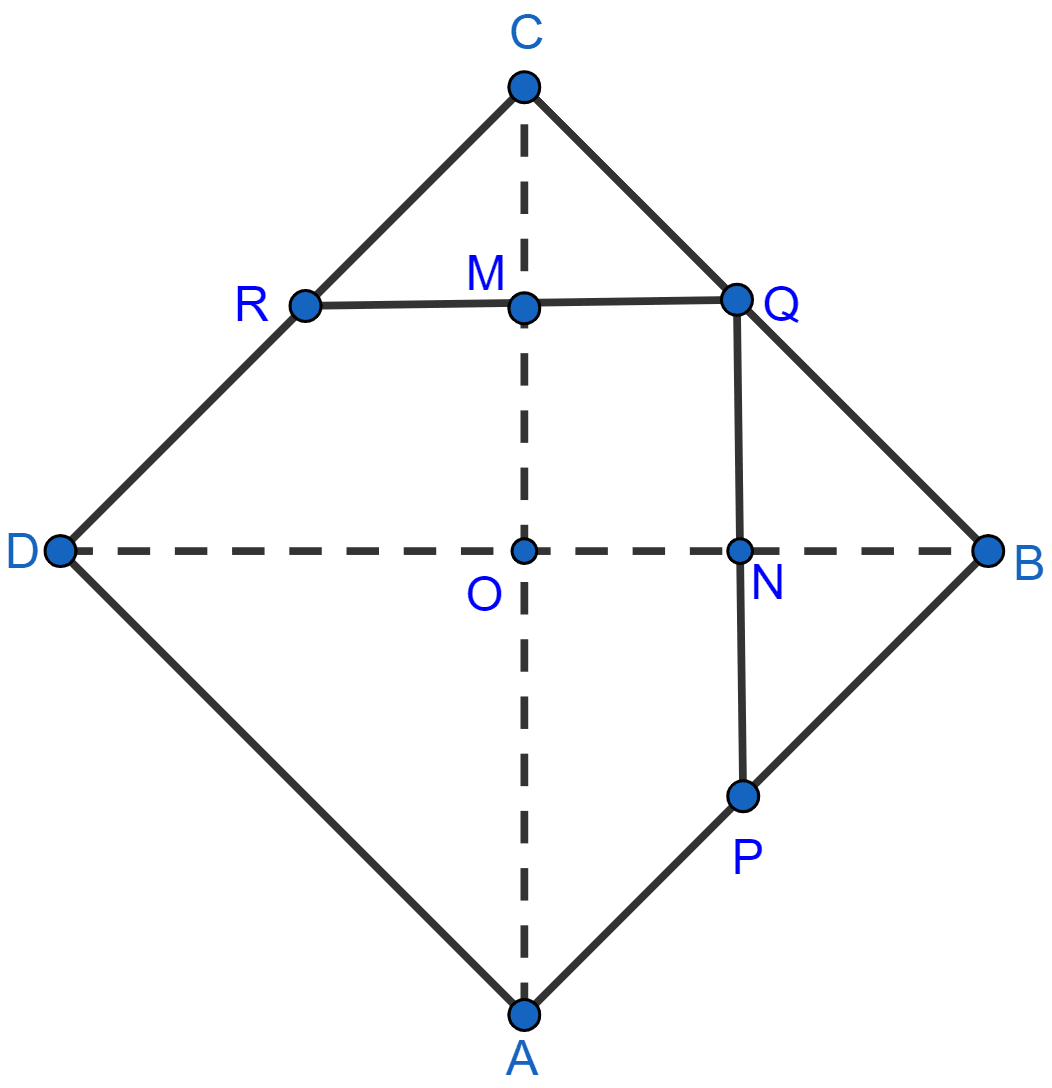
∠MON = 90°
In △BCD,
Q and R are mid-points of BC and CD.
RQ || DB and RQ = DB
RQ || DB ⇒ MQ || ON
From figure,
∠MON + ∠MQN = 180° (Sum of alternate angles of quadrilateral = 180°)
∠MQN = 180° - 90° = 90°
∴ PQ ⊥ QR.
Hence, proved that PQ ⊥ QR.
Answered By
23 Likes
Related Questions
The quadrilateral formed by joining the mid-points of the sides of a quadrilateral ABCD, taken in order is a rhombus if
ABCD is a parallelogram
ABCD is a rhombus
the diagonals of ABCD are equal
the diagonals of ABCD are perpendicular to each other.
The figure formed by joining the mid-points of the sides of a quadrilateral ABCD, taken in order, is a square only if
ABCD is a rhombus
diagonals of ABCD are equal
diagonals of ABCD are perpendicular to each other
diagonals of ABCD are equal and perpendicular to each other.
The diagonals of a quadrilateral ABCD are perpendicular. Show that the quadrilateral formed by joining the mid-points of its adjacent sides is a rectangle.
If D, E and F are mid-points of the sides BC, CA and AB respectively of a △ABC, prove that AD and FE bisect each other.