Mathematics
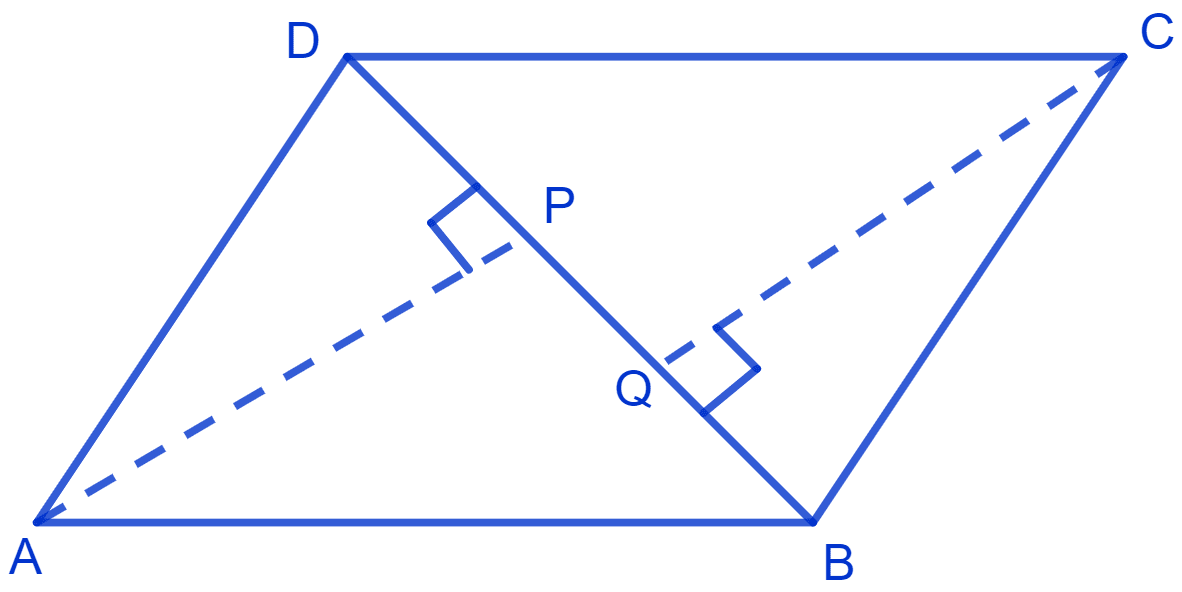
ABCD is a parallelogram and AP and CQ are perpendiculars from vertices A and C on diagonal BD. Show that
(i) Δ APB ≅ Δ CQD
(ii) AP = CQ
Rectilinear Figures
1 Like
Answer
Given :
ABCD is a parallelogram with AP ⊥ BD and CQ ⊥ BD.
From figure,
AB || DC and BD is transversal.
(i) In Δ APB and Δ CQD,
⇒ ∠APB = ∠CQD (Both equal to 90°)
⇒ AB = CD (Opposite sides of parallelogram are equal)
⇒ ∠ABP = ∠CDQ (Alternate interior angles are equal)
∴ Δ APB ≅ Δ CQD (By A.A.S. congruence rule)
Hence, proved that Δ APB ≅ Δ CQD.
(ii) Since,
Δ APB ≅ Δ CQD
We know that,
Corresponding parts of congruent triangles are equal.
⇒ AP = CQ (By C.P.C.T.)
Hence, proved that AP = CQ.
Answered By
2 Likes
Related Questions
Diagonal AC of a parallelogram ABCD bisects ∠A. Show that
(i) it bisects ∠C also,
(ii) ABCD is a rhombus.
(Ex.8.1Q3.png)
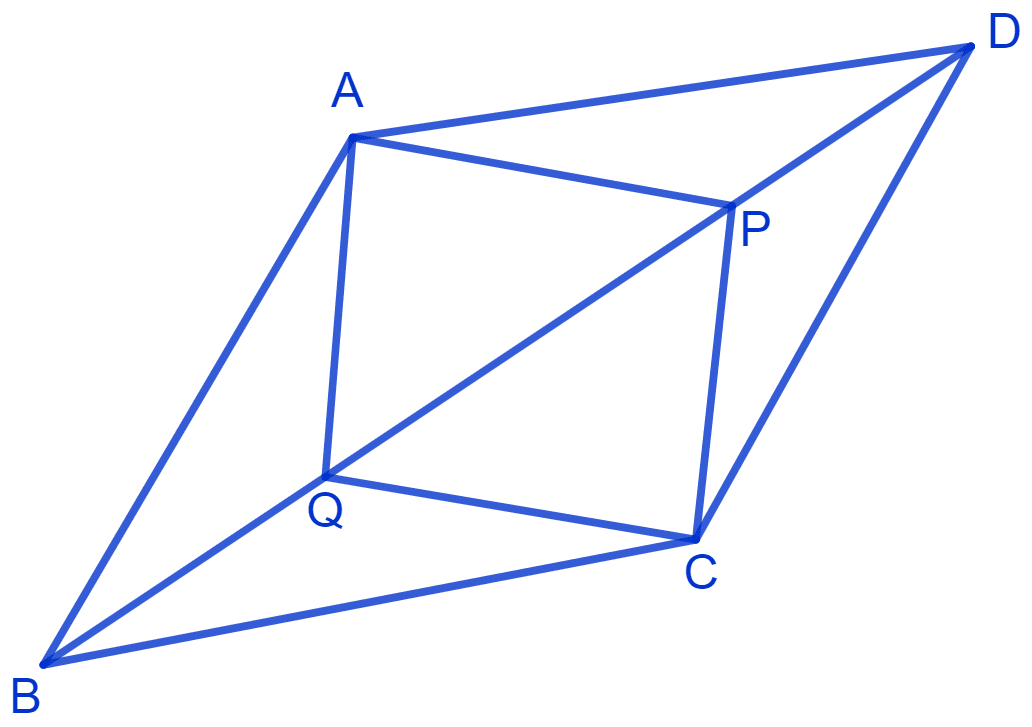
In parallelogram ABCD, two points P and Q are taken on diagonal BD such that DP = BQ see Fig. Show that:
(i) Δ APD ≅ Δ CQB
(ii) AP = CQ
(iii) Δ AQB ≅ Δ CPD
(iv) AQ = CP
(v) APCQ is a parallelogram
ABCD is a rectangle in which diagonal AC bisects ∠A as well as ∠C. Show that :
(i) ABCD is a square
(ii) diagonal BD bisects ∠B as well as ∠D.
ABCD is a trapezium in which AB || CD and AD = BC. Show that
(i) ∠A = ∠B
(ii) ∠C = ∠D
(iii) Δ ABC ≅ Δ BAD
(iv) diagonal AC = diagonal BD
