Mathematics
ABCD is a parallelogram. A circle through vertices A and B meets side BC at point P and side AD at point Q. Show that quadrilateral PCDQ is cyclic.
Answer
The figure of the parallelogram ABCD with a circle through its vertices A and B meeting side BC at point P and side AD at point Q is shown below:
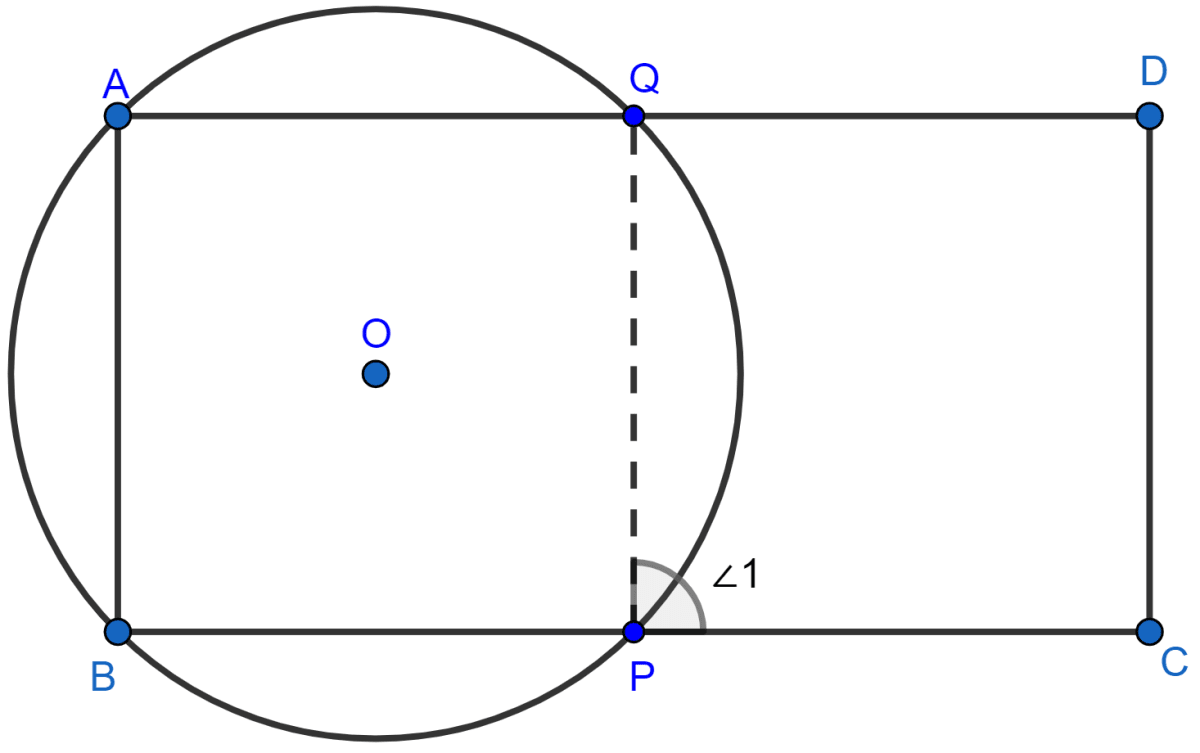
We know that,
An exterior angle of a cyclic quadrilateral is equal to its opposite interior angle.
∴ ∠1 = ∠A ……. (i)
Also,
∠A = ∠C ………..(ii) [Opposite angles of a parallelogram are equal.]
From (i) and (ii) we get,
∠1 = ∠C ………(iii)
Also,
⇒ ∠C + ∠D = 180° [Sum of co-interior angles of a parallelogram = 180°]
⇒ ∠1 + ∠D = 180°
Hence, proved that PCDQ is cyclic.
Related Questions
ABCD is a cyclic quadrilateral in a circle with centre O. If ∠ADC = 130°, find ∠BAC.

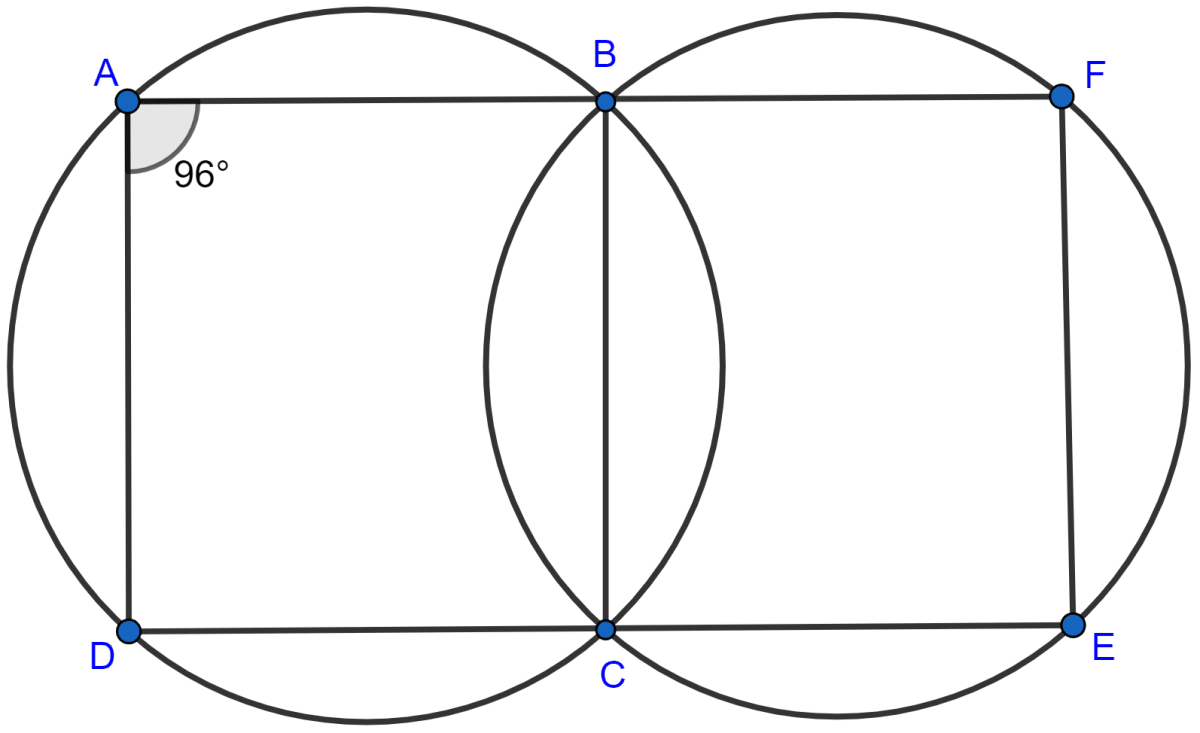
In the following figure,
(i) if ∠BAD = 96°, find ∠BCD and ∠BFE.
(ii) Prove that AD is parallel to FE.
Prove that:
(i) the parallelogram, inscribed in a circle, is a rectangle.
(ii) the rhombus, inscribed in a circle, is a square.
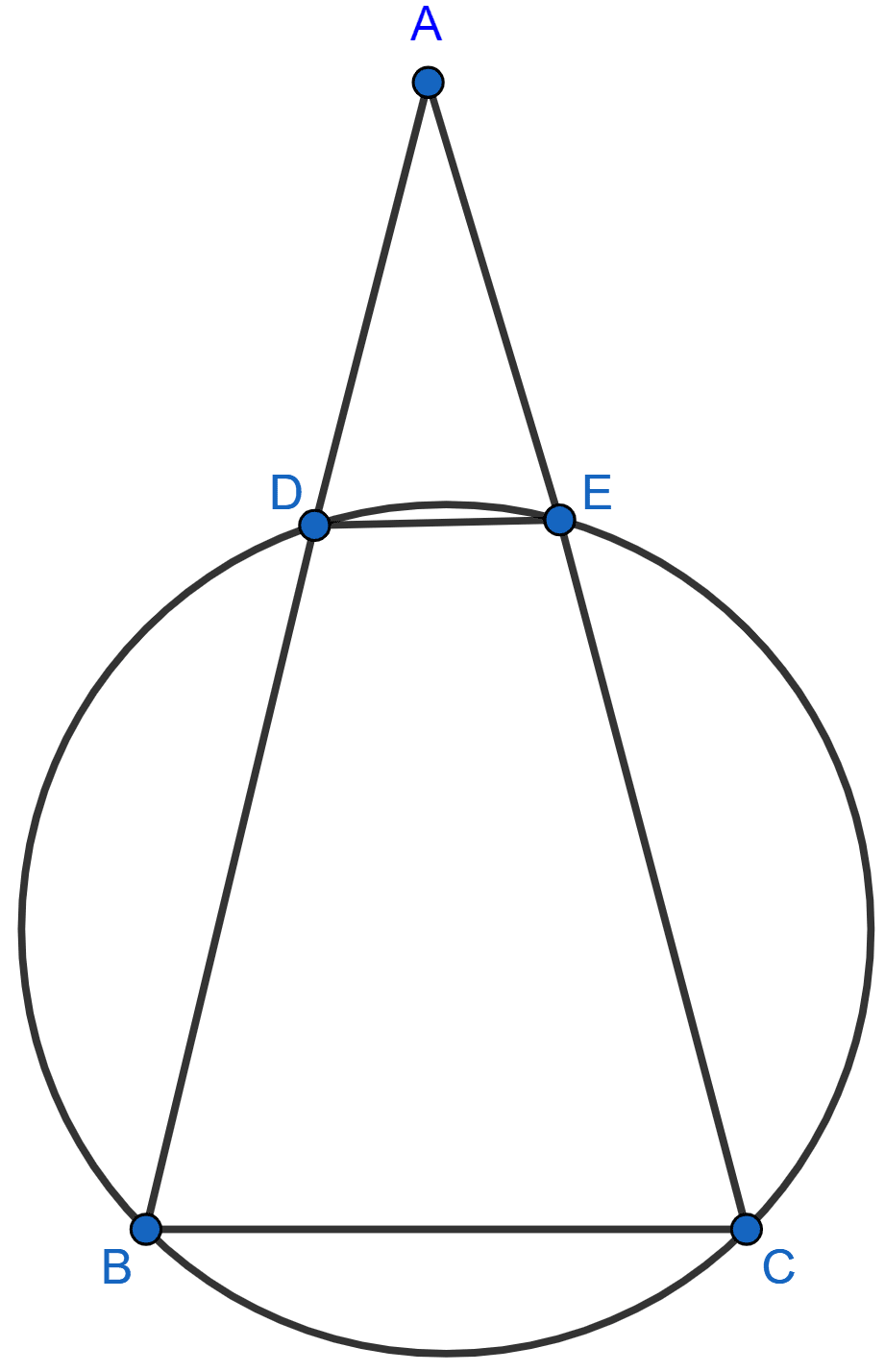
In the given figure, AB = AC. Prove that DECB is an isosceles trapezium.