Mathematics
ABC is a triangle in which AB = AC = 4 cm and ∠A = 90°. Calculate the area of △ABC. Also find the length of perpendicular from A to BC.
Mensuration
32 Likes
Answer
It is given that
AB = AC = 4 cm
From figure,
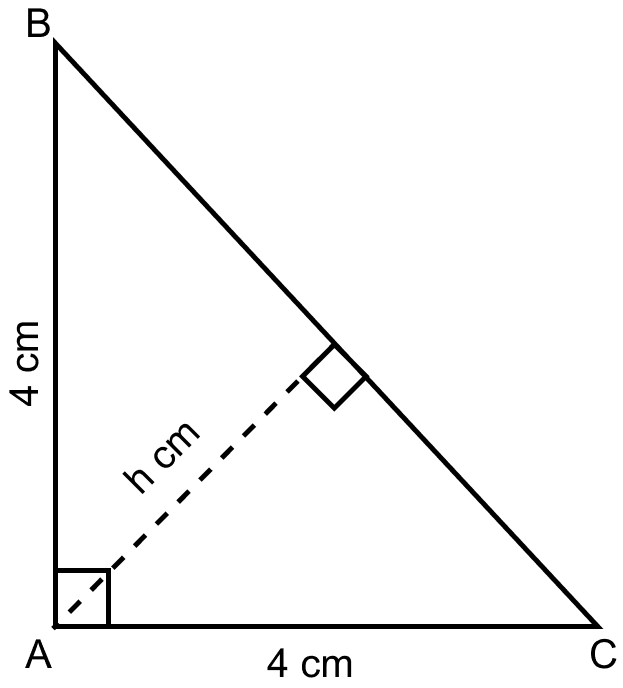
Using the Pythagoras theorem,
BC2 = AB2 + AC2
Substituting the values we get,
⇒ BC2 = 42 + 42
⇒ BC2 = 16 + 16 = 32
⇒ BC = cm.
Let perpendicular from A to BC be h cm.
Area of △ABC = × base × height
= × AC × AB
=
= 8 cm.
From figure,
Area of △ABC = × BC × h.
Hence, area of △ABC = 8 cm2 and length of perpendicular from A to BC = 2.83 cm.
Answered By
15 Likes
Related Questions
If the lengths of the sides of a triangle are in the ratio 3: 4 : 5 and its perimeter is 48 cm, find its area.
The sides of a triangular plot are in the ratio 3 : 5 : 7 and its perimeter is 300 m. Find its area. Take .
Find the area of an isosceles triangle whose equal sides are 12 cm each and the perimeter is 30 cm.
Find the area of an isosceles triangle whose base is 6 cm and perimeter is 16 cm.