Mathematics
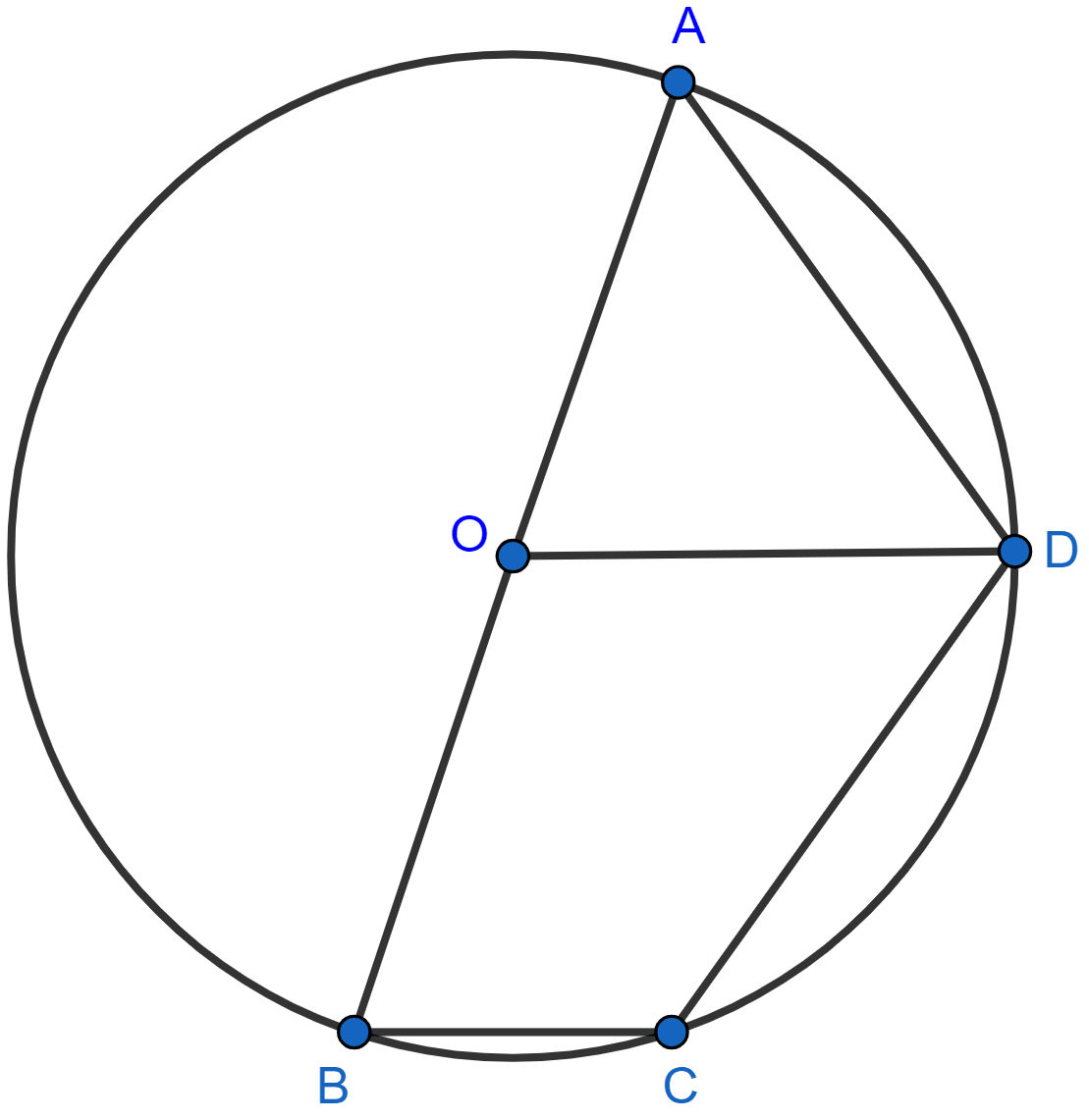
AB is the diameter of the circle with center O. OD is parallel to BC and ∠AOD = 60°. Calculate the numerical values of :
(i) ∠ABD,
(ii) ∠DBC,
(iii) ∠ADC.
Circles
15 Likes
Answer
Join BD.
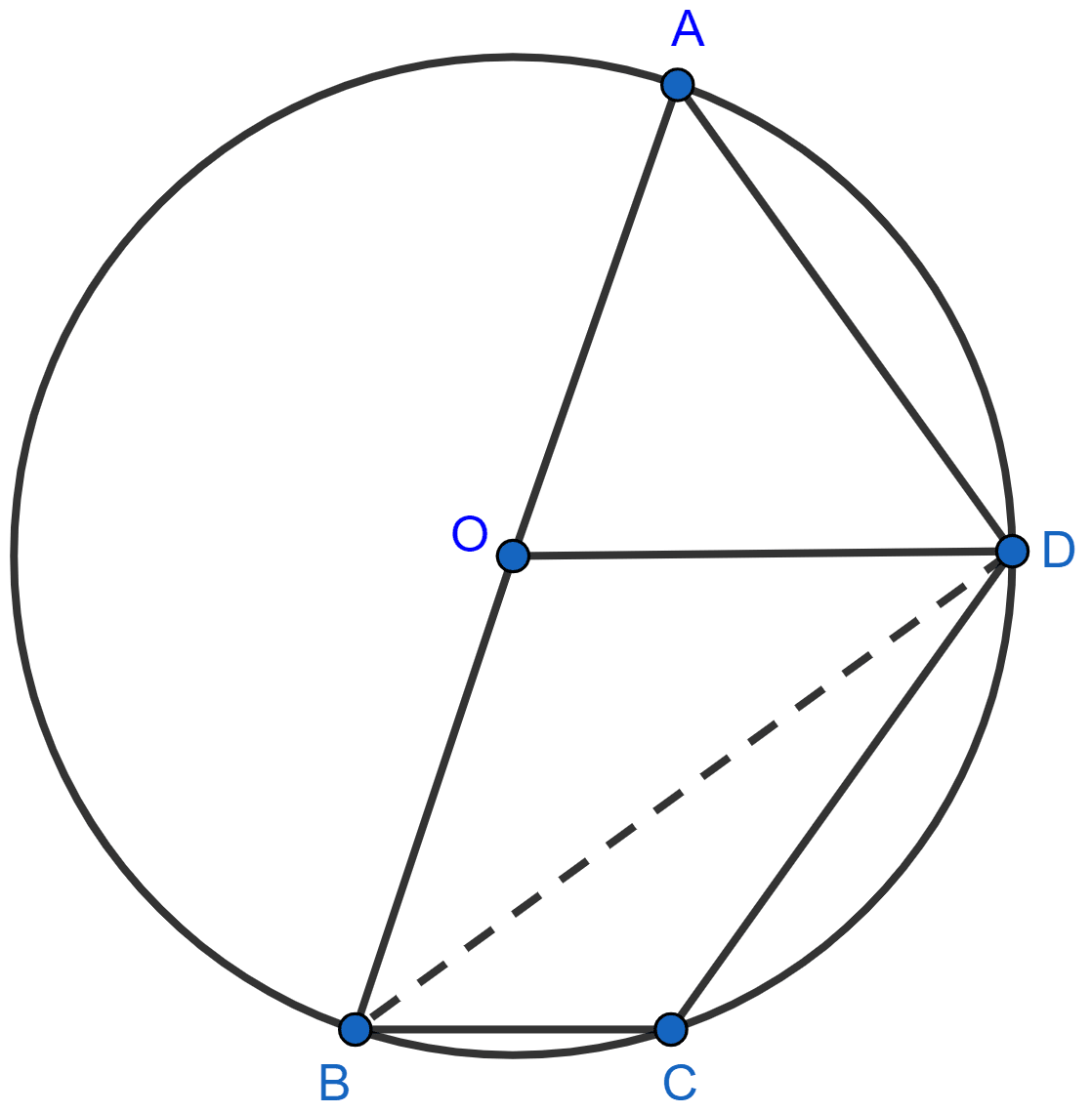
(i) From figure,
⇒ ∠BDA = 90° [Angle in semi-circle is a right angle.]
In △OAD,
OA = OD [Radius of same circle]
∠OAD = ∠ODA = x (let) [As angles opposite to equal side are equal]
In △OAD,
⇒ ∠OAD + ∠ODA + ∠AOD = 180° [Angle sum property of triangle]
⇒ x + x + 60° = 180°
⇒ 2x = 180° - 60°
⇒ 2x = 120°
⇒ x = = 60°.
From figure,
∠ODB = ∠BDA - ∠ADO = 90° - 60° = 30°.
Given, OD || BC
∠DBC = ∠ODB = 30° [Alternate angles are equal]
Hence, ∠DBC = 30°.
(ii) We know that,
Angle in a semi-circle is a right angle.
∴ ∠BDA = 90°.
Since,
∠OAD = ∠ODA = ∠AOD = 60°.
From figure,
⇒ ∠BDA = ∠ODA + ∠ODB
⇒ 90° = 60° + ∠ODB
⇒ ∠ODB = 90° - 60° = 30°.
Given,
OD || BC
∴ ∠DBC = ∠ODB = 30° [Alternate angles are equal].
Hence, ∠DBC = 30°.
(iii) From figure,
∠ABC = ∠ABD + ∠DBC = 30° + 30° = 60°.
Since, sum of opposite nagles in a cyclic quadrilateral = 180°.
∴ ∠ABC + ∠ADC = 180°
⇒ 60° + ∠ADC = 180°
⇒ ∠ADC = 120°.
Hence, ∠ADC = 120°.
Answered By
9 Likes
Related Questions
Use the given figure to find :
(i) ∠BAD
(ii) ∠DQB.

In the given figure, PQ is a diameter. Chord SR is parallel to PQ. Given that ∠PQR = 58°, Calculate :
(i) ∠RPQ
(ii) ∠STP.

In the given figure, the center O of the small circle lies on the circumference of the bigger circle. If ∠APB = 75° and ∠BCD = 40°, find :
(i) ∠AOB,
(ii) ∠ACB,
(iii) ∠ABD,
(iv) ∠ADB.
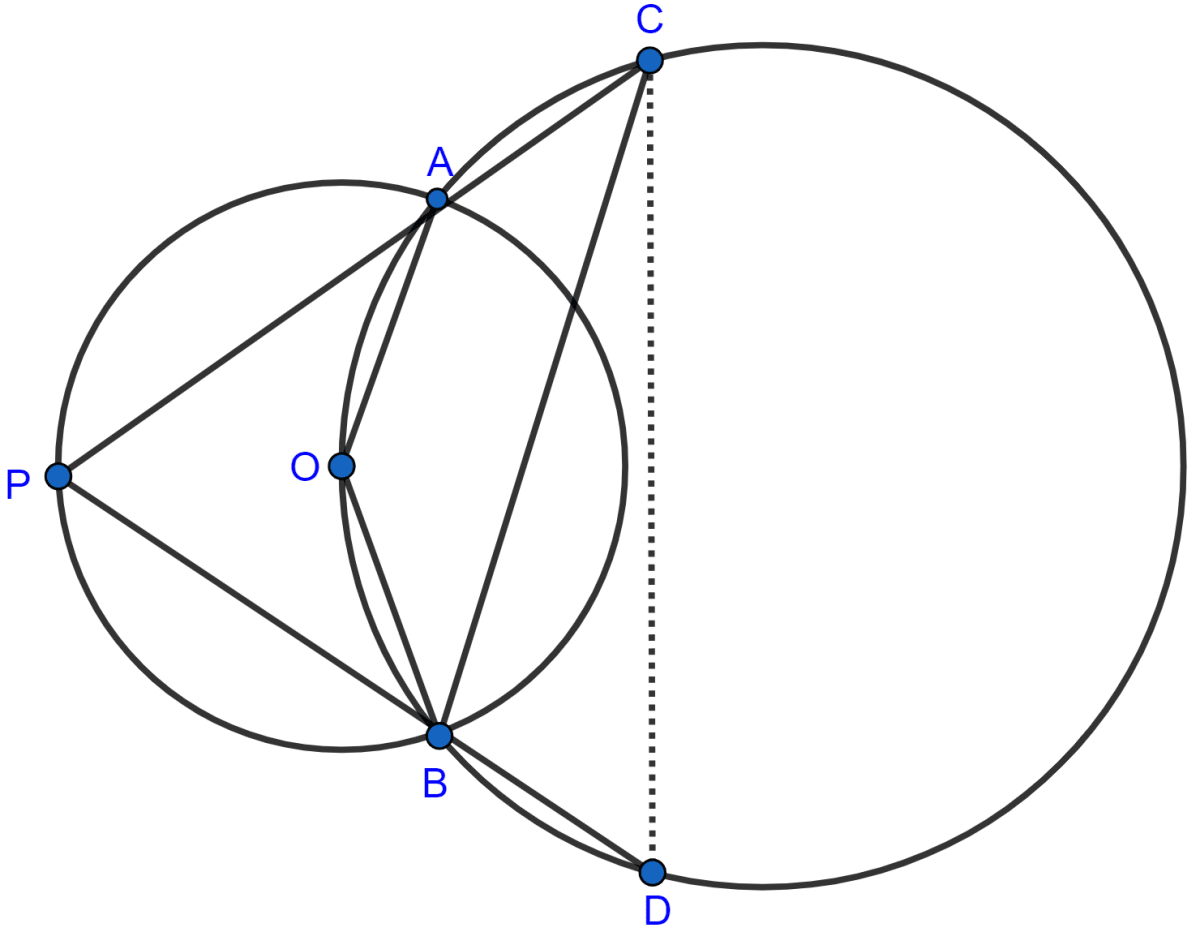
In the given figure, ∠BAD = 65°, ∠ABD = 70° and ∠BDC = 45°. Find :
(i) ∠BCD
(ii) ∠ACB
Hence, show that AC is a diameter.
