Mathematics
A wooden article was made by scooping out a hemisphere from each end of a solid cylinder, as shown in the figure. If the height of the cylinder is 10 cm, and its base is of radius 3.5 cm, find the total surface area of the article.
Mensuration
16 Likes
Answer
Given,
Height of cylinder = 10 cm
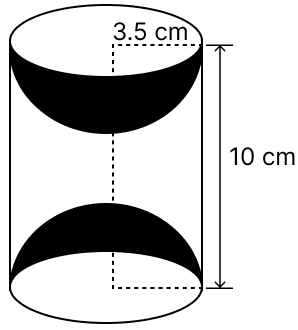
From figure,
Radius of base of cylinder = Radius of hemisphere = r = 3.5 cm
From figure,
Total surface area of article = Curved surface area of cylinder + 2 × Curved surface area of each hemisphere
= 2πrh + 2 × 2πr2
= 2πr(h + 2r)
Substituting values we get :
Hence, the total surface area of the article is 374 cm2.
Answered By
11 Likes
Related Questions
A tent is in the shape of a cylinder surmounted by a conical top. If the height and diameter of the cylindrical part are 2.1 m and 4 m respectively, and the slant height of the top is 2.8 m, find the area of the canvas used for making the tent. Also, find the cost of the canvas of the tent at the rate of ₹ 500 per m2. (Note that the base of the tent will not be covered with canvas.)
From a solid cylinder whose height is 2.4 cm and diameter 1.4 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid to the nearest cm2.
A solid is in the shape of a cone standing on a hemisphere with both their radii being equal to 1 cm and the height of the cone is equal to its radius. Find the volume of the solid in terms of π.
Rachel, an engineering student, was asked to make a model shaped like a cylinder with two cones attached at its two ends by using a thin aluminium sheet. The diameter of the model is 3 cm and its length is 12 cm. If each cone has a height of 2 cm, find the volume of air contained in the model that Rachel made. (Assume the outer and inner dimensions of the model to be nearly the same.)