Mathematics
A vertical pole and a vertical tower are on the same level ground. From the top of the pole, the angle of elevation of the top of the tower is 60° and the angle of depression of the foot of tower is 30°. Find the height of the tower if the height of the pole is 20 m.
Heights & Distances
53 Likes
Answer
Let AB be the pole and CD be the tower. Let length of tower (CD) be h metres.
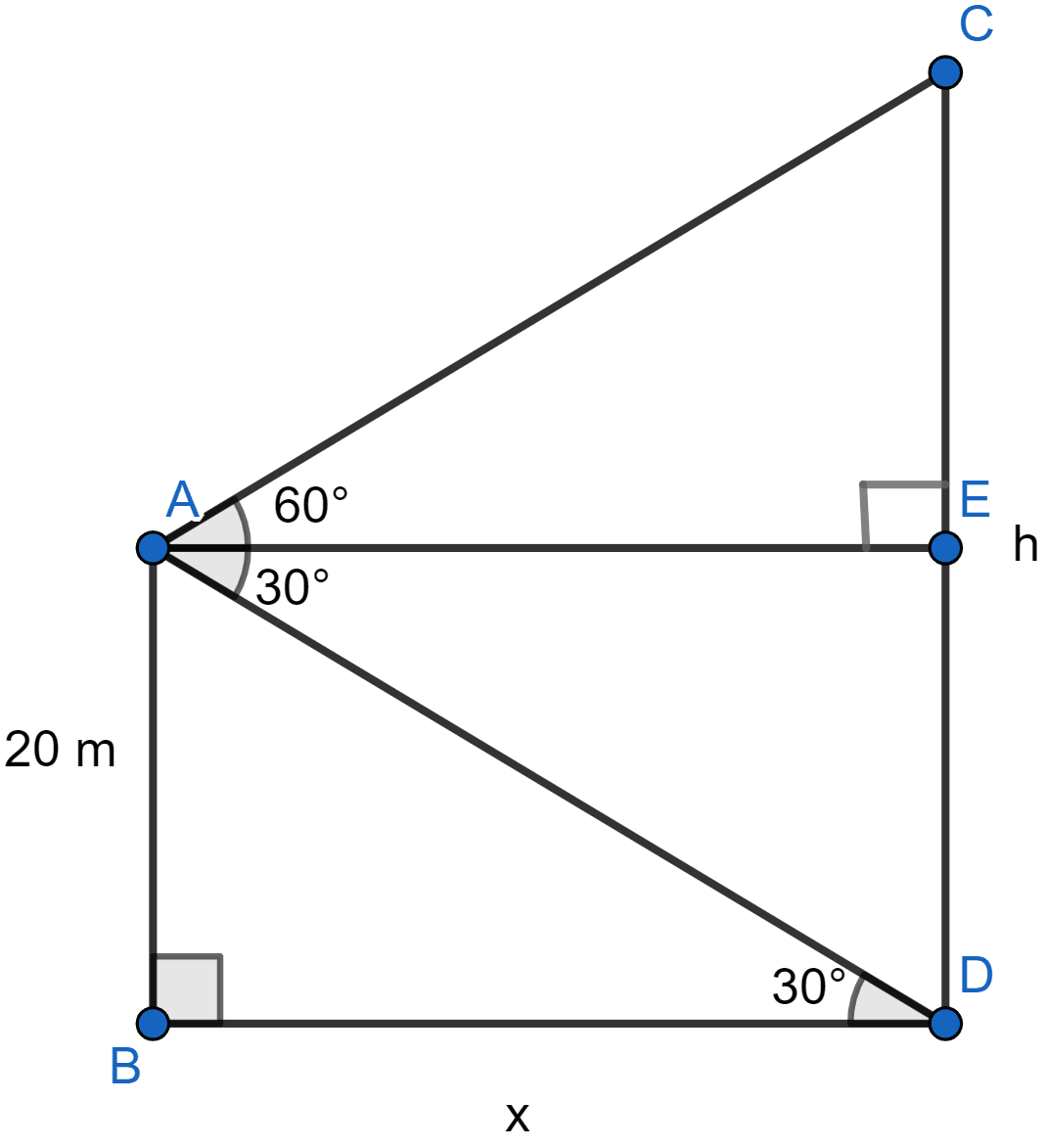
Let distance between pole and tower (BD) be x meters.
From figure,
ABDE is a rectangle so,
DE = AB = 20 meters
AE = BD = x meters
CE = CD - DE = (h - 20) meters
∠EAD = ∠ADB = 30° (Alternate angles are equal)
Considering right angled △ABD we get,
Considering right angled △ACE we get,
Hence, the height of the tower is 80 meters.
Answered By
15 Likes
Related Questions
The angles of depression of the top and the bottom of a 8 m tall building from the top of a multi-storeyed building are 30° and 45° respectively. Find the height of the multi storeyed building and the distance between the two buildings, correct to two decimal places.
A pole of height 5 m is fixed on the top of a tower. The angle of elevation of the top of pole as observed from a point A on the ground is 60° and the angle of depression of the point A from the top of the tower is 45°. Find the height of the tower. (Take )
From the top of a building 20 m high, the angle of elevation of the top of a monument is 45° and the angle of depression of its foot is 15°. Find the height of the monument.
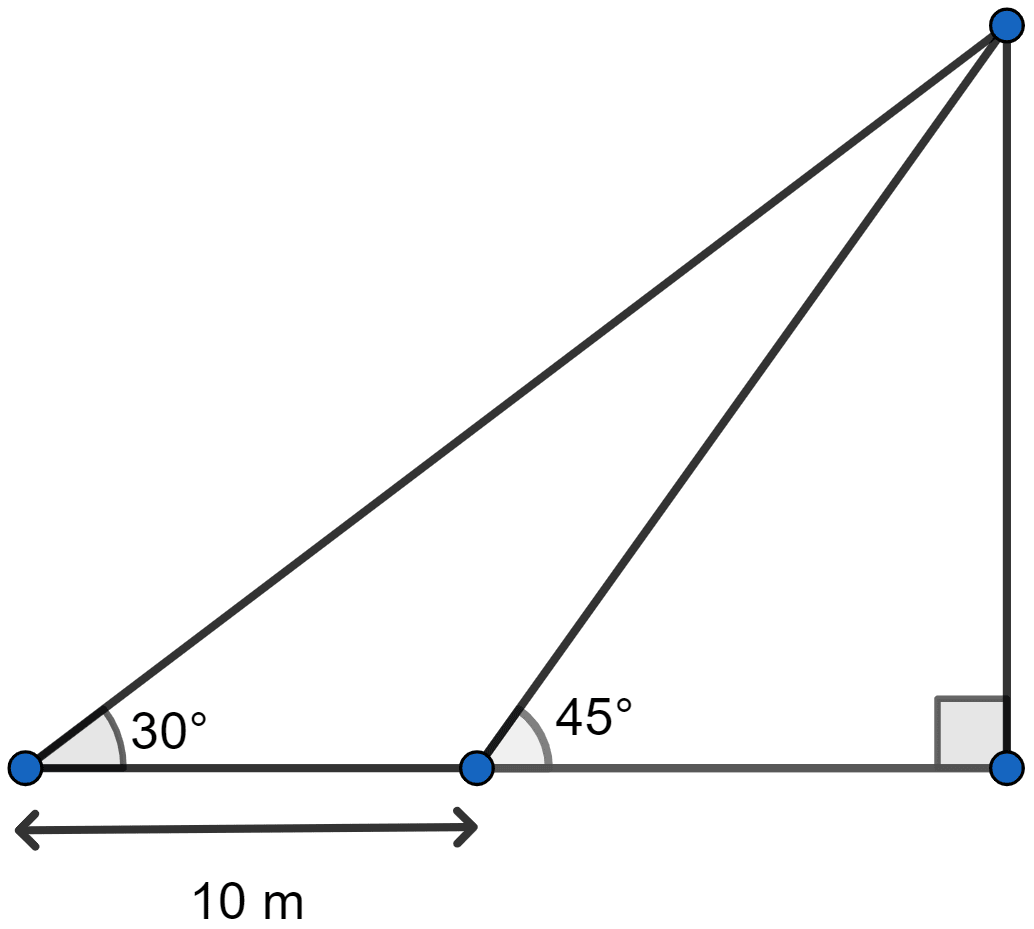
In the adjoining figure, the shadow of a vertical tower on the level ground increases by 10 m, when the altitude of the sun changes from 45° to 30°. Find the height of the tower and give your answer, correct to of a metre.