A triangle ABC is drawn to circumscribe a circle of radius 4 cm such that the segments BD and DC into which BC is divided by the point of contact D are of lengths 8 cm and 6 cm respectively in figure. Find the sides AB and AC.

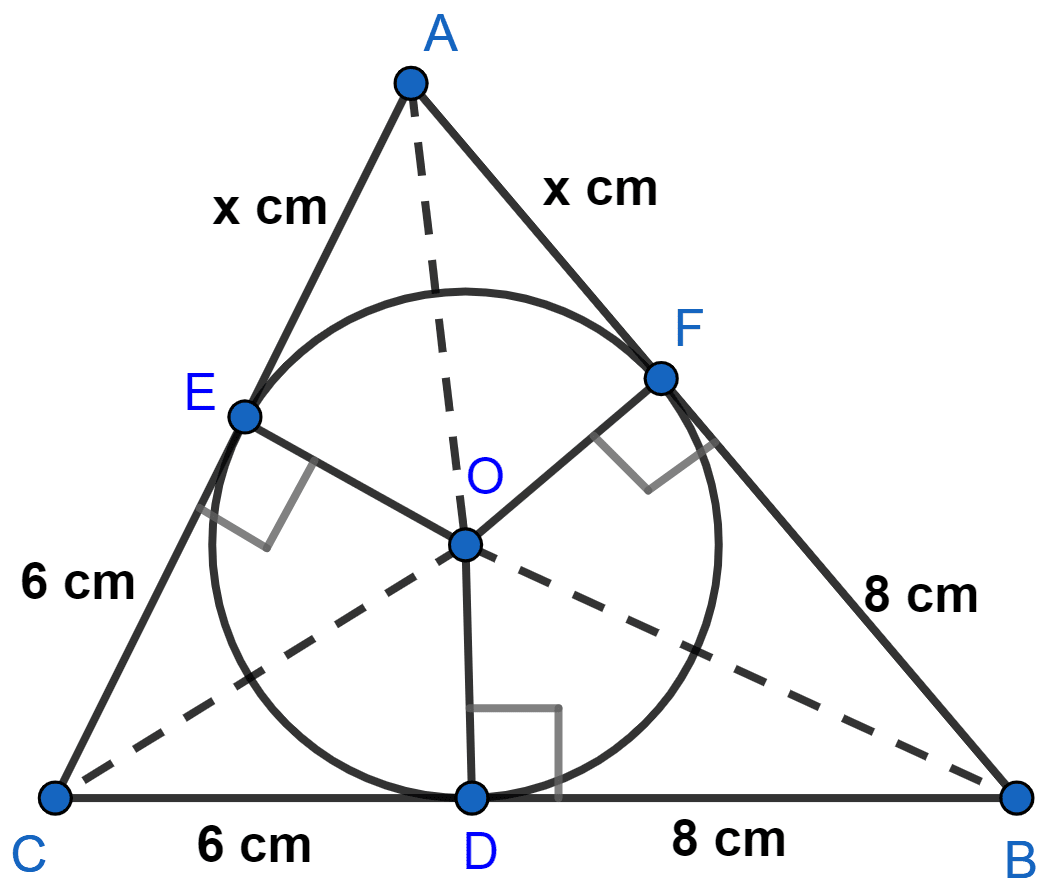
Draw OE perpendicular to AC and OF perpendicular to AB.

We know that,

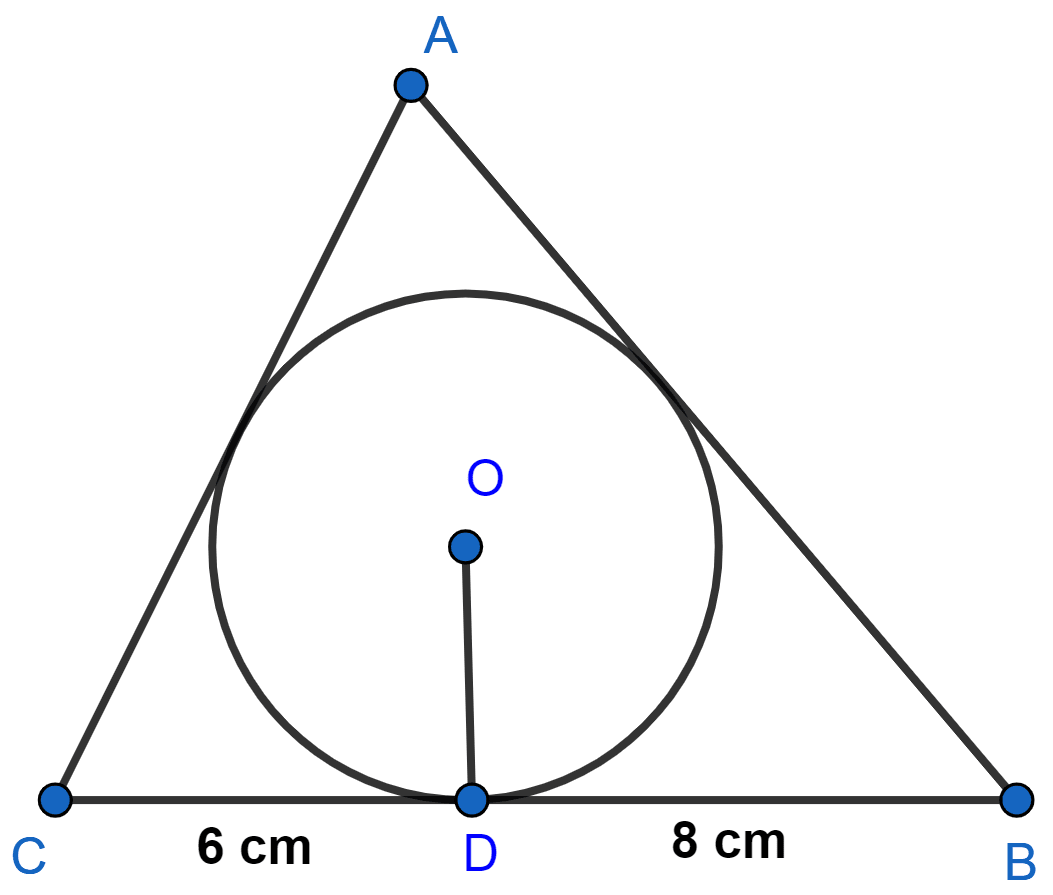
The lengths of tangents drawn from an external point to a circle are equal.

CE = CD = 6 cm, BF = BD = 8 cm.

AE = AF = x cm (let)

By heron's formula,

Area = $\sqrt{s(s - a)(s - b)(s - c)}$, where a, b and c are sides of triangle and s = $\dfrac{1}{2}$ (AB + BC + AC).

Substituting values we get :

$s = \dfrac{1}{2}[(AF + BF) + (BD + CD) + (AE + CE)] \\[1em] = \dfrac{1}{2}[(x + 8) + (8 + 6) + (x + 6)] \\[1em] = \dfrac{1}{2}[2x + 28] \\[1em] = \dfrac{1}{2} \times 2[x + 14] \\[1em] = x + 14.$

Substituting value of s in Heron's formula,

$\text{Area } = \sqrt{(x + 14)(x + 14 - AB)(x + 14 - BC)(x + 14 - AC)} \\[1em] = \sqrt{(x + 14)[x + 14 - (x + 8)][x + 14 - 14][x + 14 - (x + 6)]} \\[1em] = \sqrt{(x + 14)\times 6 \times x \times 8} \\[1em] = \sqrt{48(x^2 + 14x)} \text{ cm}^2.$

From figure,

Area of △ABC = Area of △AOB + Area of △BOC + Area of △AOC …………(1)

We know that,

Area of triangle = $\dfrac{1}{2} \times \text{ base } \times \text{height}$

Substituting values we get :

$\text{Area of △AOB } = \dfrac{1}{2} \times AB \times OF \\[1em] = \dfrac{1}{2} \times (x + 8) \times 4 \\[1em] = 2(x + 8) \\[1em] = (2x + 16) \text{ cm}^2. \\[1em] \text{Area of △BOC } = \dfrac{1}{2} \times BC \times OD \\[1em] = \dfrac{1}{2} \times 14 \times 4 \\[1em] = 28 \text{ cm}^2. \\[1em] \text{Area of △AOC } = \dfrac{1}{2} \times AC \times OE \\[1em] = \dfrac{1}{2} \times (x + 6) \times 4 \\[1em] = 2(x + 6) \\[1em] = (2x + 12) \text{ cm}^2. \\[1em]$

Substituting values in equation (1), we get :

$\Rightarrow \sqrt{48(x^2 + 14x)} = (2x + 16) + 28 + (2x + 12) \\[1em] \Rightarrow \sqrt{48(x^2 + 14x)} = 4x + 56 \\[1em] \Rightarrow \sqrt{48(x^2 + 14x)} = 4(x + 14)$

Squaring both sides we get :

$\Rightarrow 48(x^2 + 14x) = 4^2 \times (x + 14)^2 \\[1em] \Rightarrow 48(x^2 + 14x) = 16(x^2 + 196 + 28x) \\[1em] \Rightarrow \dfrac{48}{16}(x^2 + 14x) = x^2 + 196 + 28x \\[1em] \Rightarrow 3x^2 + 42x = x^2 + 196 + 28x \\[1em] \Rightarrow 3x^2 - x^2 + 42x - 28x - 196 = 0 \\[1em] \Rightarrow 2x^2 + 14x - 196 = 0 \\[1em] \Rightarrow 2(x^2 + 7x - 98) = 0 \\[1em] \Rightarrow x^2 + 7x - 98 = 0 \\[1em] \Rightarrow x^2 + 14x - 7x - 98 = 0 \\[1em] \Rightarrow x(x + 14) - 7(x + 14) = 0 \\[1em] \Rightarrow (x - 7)(x + 14) = 0 \\[1em] \Rightarrow x - 7 = 0 \text{ or } x + 14 = 0 \\[1em] \Rightarrow x = 7 \text{ or } x = -14.$

Since, side cannot be negative.

∴ x = 7 cm.

From figure,

AB = AF + BF = x + 8 = 7 + 8 = 15 cm

AC = AE + CE = x + 6 = 7 + 6 = 13 cm.

Hence, AB = 15 cm and AC = 13 cm.