A straight line passes through the point (3, 2) and the portion of this line, intercepted between the positive axes, is bisected at this point. Find the equation of the line.

Let P = (3, 2).

Let the line intersect the x-axis at point A (x, 0) and y-axis at point B (0, y).

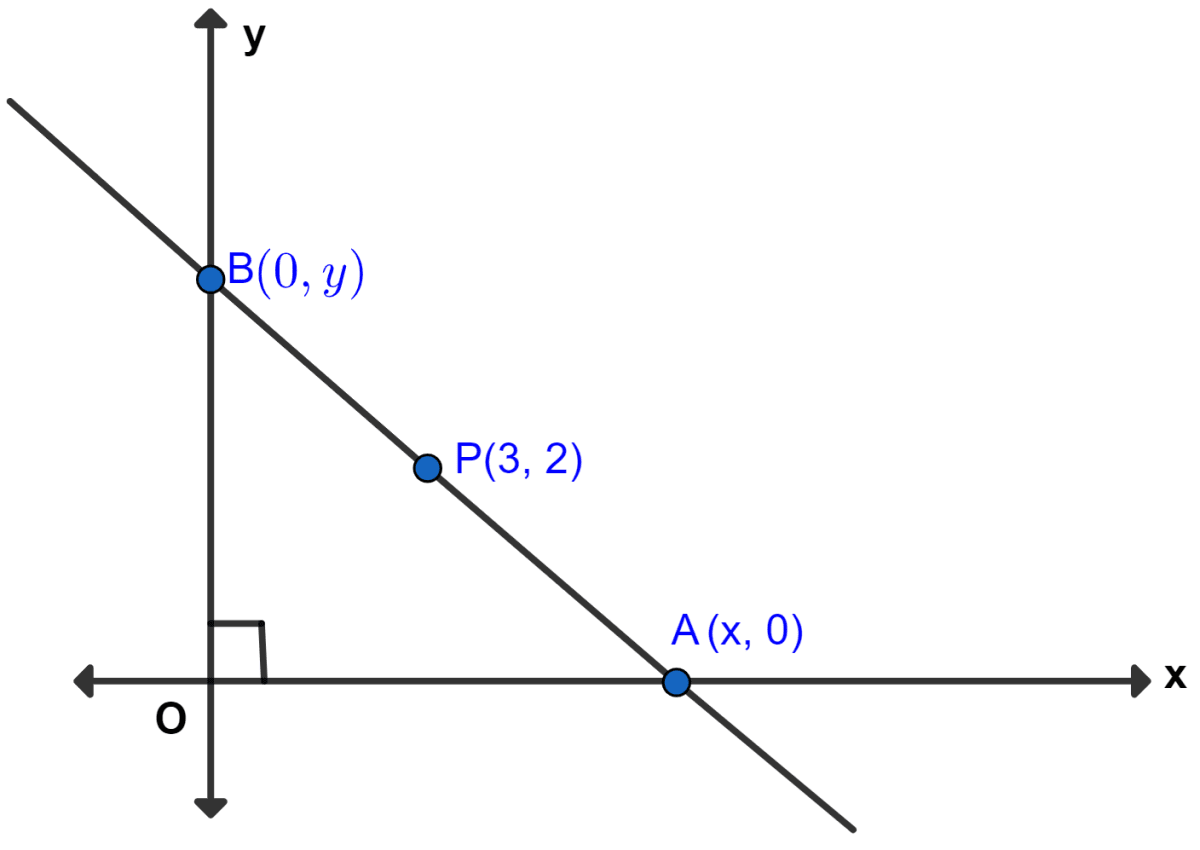
Since, P is the mid-point of AB, we have:

$\Rightarrow P = \Big(\dfrac{x + 0}{2}, \dfrac{0 + y}{2}\Big) \\[1em] \Rightarrow (3, 2) = \Big(\dfrac{x}{2}, \dfrac{y}{2}\Big) \\[1em] \Rightarrow \dfrac{x}{2} = 3 \text{ and } \dfrac{y}{2} = 2\\[1em] \Rightarrow x = 6 \text{ and } y = 4.$

Thus, A = (6, 0) and B = (0, 4)

Slope of line AB = $\dfrac{4 - 0}{0 - 6} = \dfrac{4}{-6} = -\dfrac{2}{3}$.

So, the required equation of the line AB is given by

⇒ y – y₁ = m(x – x₁)

⇒ y – 0 = $-\dfrac{2}{3}$(x – 6)

⇒ 3y = -2x + 12

⇒ 2x + 3y = 12

Hence, equation of the required line is 2x + 3y = 12.