Mathematics
A solid metallic sphere of radius 6 cm is melted and made into a solid cylinder of height 32 cm. Find the :
(i) radius of the cylinder
(ii) curved surface area of the cylinder. Take π = 3.1
Mensuration
45 Likes
Answer
(i) Radius of metallic sphere (R) = 6 cm
Height of cylinder (h) = 32 cm
Volume of cylinder = Volume of metallic sphere (As sphere is melted and formed into a cylinder).
Let radius of cylinder = r cm.
Hence, the radius of the cylinder = 3 cm.
(ii) Curved surface area of cylinder = 2πrh
Putting values we get,
Curved surface area of cylinder = 2 × 3.1 × 3 × 32 = 595.2 cm2.
Hence, curved surface area of cylinder = 595.2 cm2.
Answered By
14 Likes
Related Questions
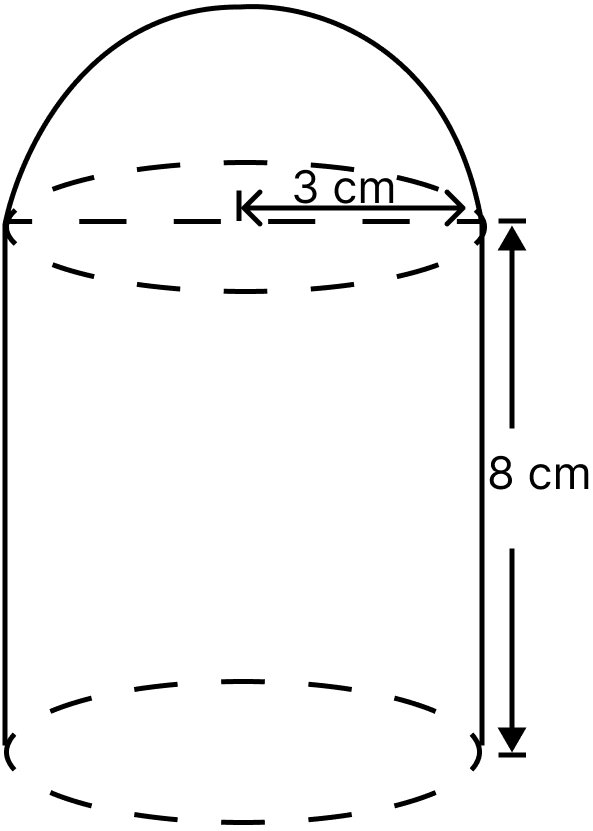
The adjoining figure shows a model of a solid consisting of a cylinder surmounted by a hemisphere at one end. If the model is drawn to a scale of 1 : 200, find
(i) the total surface area of the solid in π m2.
(ii) the volume of the solid in π litres.
The diameter of a metallic sphere is 6 cm. The sphere is melted and drawn into a wire of uniform cross-section. If the length of the wire is 36 m, find its radius.
A solid metallic hemisphere of radius 8 cm is melted and recasted into right circular cone of base radius 6 cm. Determine the height of the cone.
A rectangular water tank of base 11 m × 6 m contains water upto a height of 5 m if the water in the tank is transferred to a cylindrical tank of radius 3.5 m, find the height of water level in the tank.