A man standing on the deck of the ship which is 20 m above the sea level, observes the angle of elevation of a bird as 30° and the angle of depression of its reflection in the sea as 60°. Find the height of the bird.

Let P be the man standing on the deck of the ship which is 20 m above sea level and B is the bird.

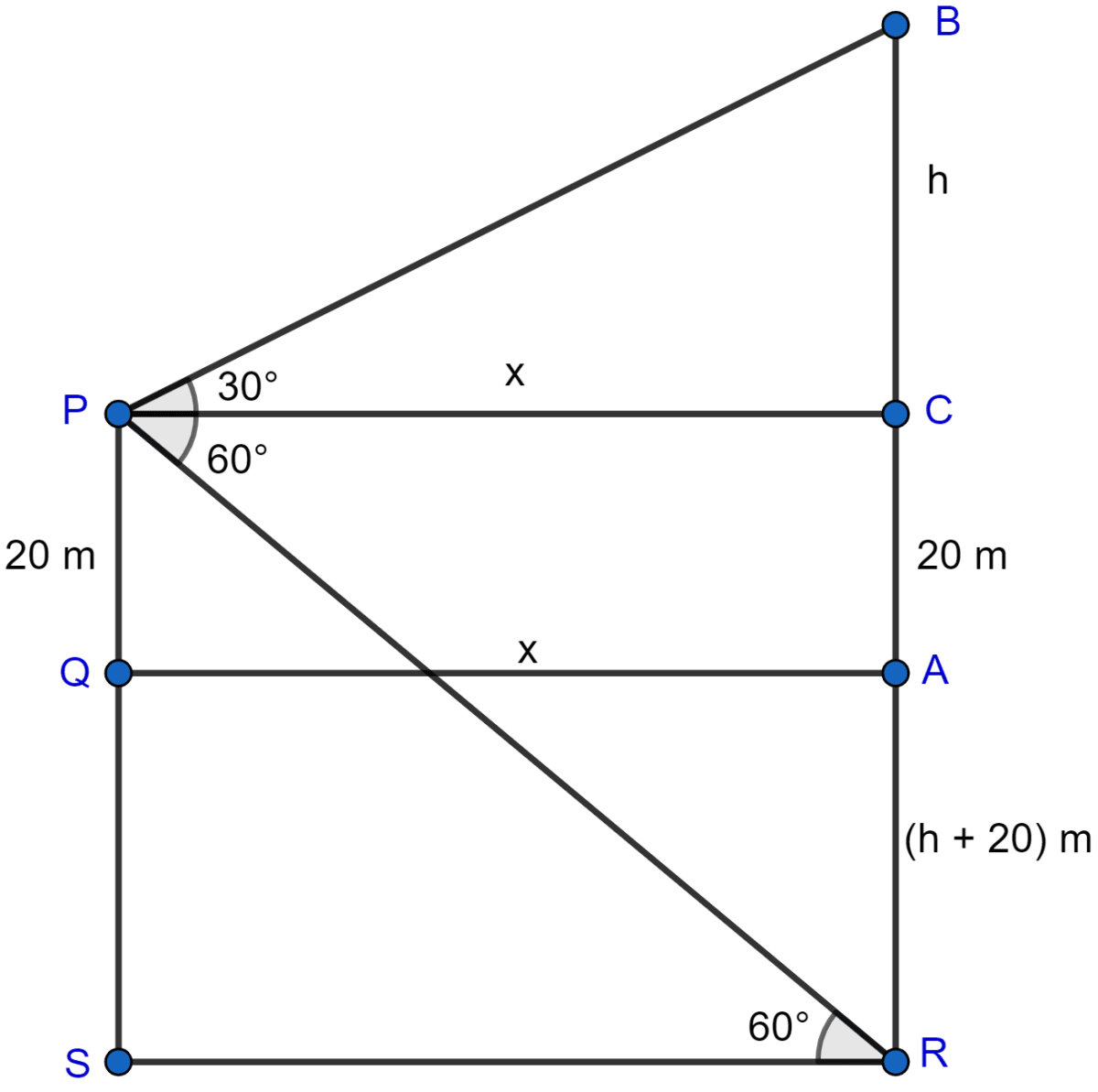
Let bird be flying above h meters from deck of the ship.

∴ BC = h

Let the shadow be created at point R, the shadow will be created vertically opposite at same distance as bird is from ship.

∴ AR = AB = AC + BC = h + 20.

From figure,

In right angled triangle △PCB,

$\Rightarrow \text{tan 30°} = \dfrac{BC}{PC} \\[1em] \Rightarrow \dfrac{1}{\sqrt{3}} = \dfrac{h}{x} \\[1em] \Rightarrow x = h\sqrt{3} ……(i)$

In right angled triangle △PCR,

$\Rightarrow \text{tan 60°} = \dfrac{CR}{CP} \\[1em] \Rightarrow \sqrt{3} = \dfrac{h + 40}{x} \\[1em] \Rightarrow \dfrac{h + 40}{h\sqrt{3}} = \sqrt{3} \quad \text{ [From (i)]}\\[1em] \Rightarrow h + 40 = \sqrt{3} \times \sqrt{3}h \\[1em] \Rightarrow h + 40 = 3h \\[1em] \Rightarrow 3h - h = 40 \\[1em] \Rightarrow 2h = 40 \\[1em] \Rightarrow h = 20.$

From sea level, height of bird (AB) = h + 20 = 20 + 20 = 40 m.

Hence, from sea level the height of bird is 40 m.