Mathematics
A conical vessel of radius 6 cm and height 8 cm is completely filled with water. A sphere is lowered into the water and its size is such that when it touches the sides it is just immersed . What fraction of water overflows ?
Mensuration
25 Likes
Answer
Given,
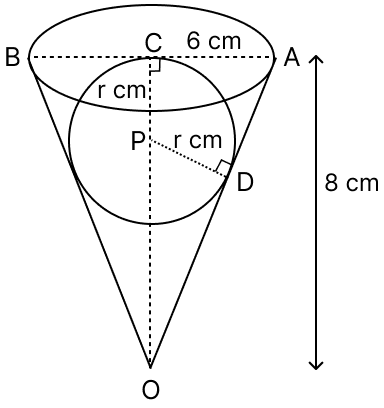
Radius of conical vessel (R) = AC = 6 cm
Height of conical vessel (H) = OC = 8 cm
Radius of sphere (r)
∴ PC = PD = r cm.
We know that,
Since, lengths of two tangents from an external point to a circle are equal.
∴ AC = AD = 6 cm
As radius from center to the point of tangent are perpendicular to each other.
△OCA and △OPD are right angle triangles.
In △OCA,
By pythagoras theorem,
In △OPD,
By pythagoras theorem,
From figure,
OD = OA - AD = 10 - 6 = 4 cm.
OP = OC - PC = 8 - r
Substituting values of OP, OD and PD in equation (1), we get :
Volume of water overflown = Volume of sphere
=
= cm3.
Original volume of water = Volume of cone
= = 96π cm3.
Fraction of water overflown = .
Hence, fraction of water overflown = .
Answered By
6 Likes
Related Questions
Use ruler and compasses for this question.
(i) Construct an isosceles triangle ABC in which AB = AC = 7.5 cm and BC = 6 cm.
(ii) Draw AD, the perpendicular from vertex A to side BC.
(iii) Draw a circle with center A and radius 2.8 cm, cutting AD at E.
(iv) Construct another circle to circumscribe the triangle BCE.
In triangle ABC, ∠BAC = 90°, AB = 6 cm and BC = 10 cm. A circle is drawn inside the triangle which touches all the sides of the triangle (i.e. an incircle of △ABC is drawn). Find the area of the triangle excluding the circle.
Prove that :
= 2(sec A + cosec A)
Prove that :
= 2 tan A