A (2, 5), B (1, 0), C(-4, 3) and D (-3, 8) are the vertices of quadrilateral ABCD. Find the co-ordinates of the mid-points of AC and BD. Give a special name to the quadrilateral.

By formula,

Mid-point = $\Big(\dfrac{x$1 + x2}{2}, \dfrac{y1 + y2}{2}\Big)

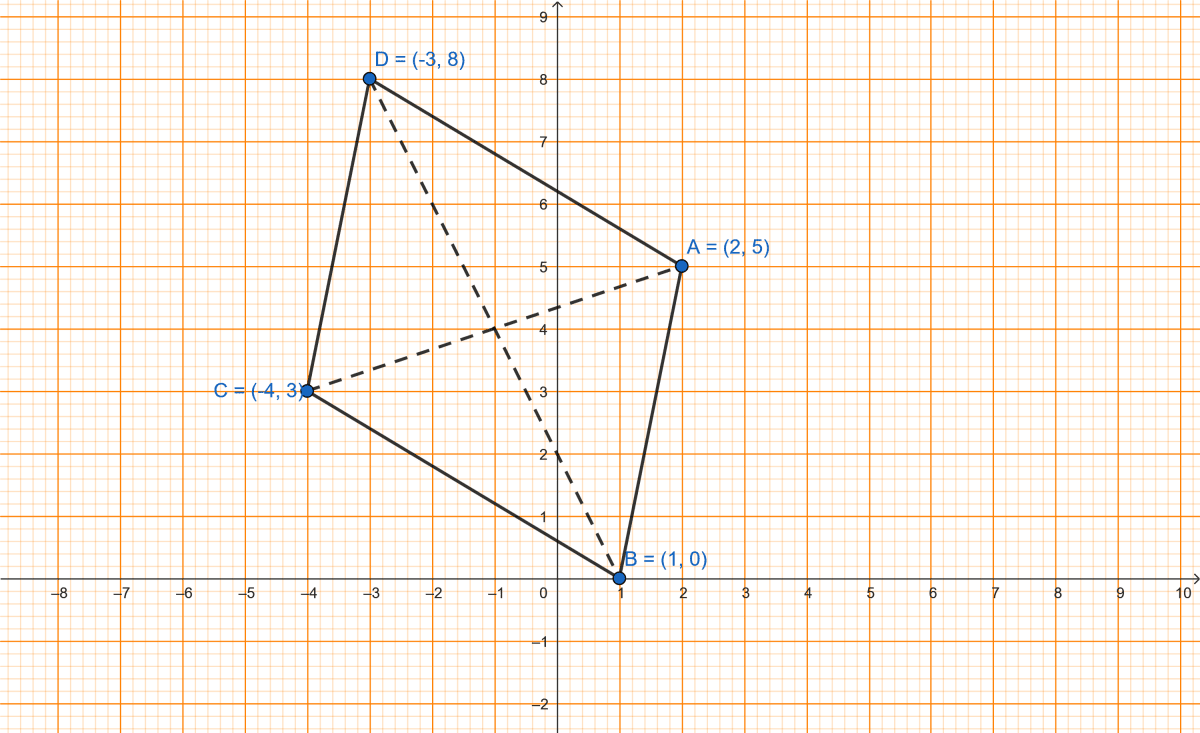
Let mid-point of AC be E.

Substituting value we get,

$E = \Big[\dfrac{2 + (-4)}{2}, \dfrac{5 + 3}{2}\Big] \\[1em] = \Big(-\dfrac{2}{2}, \dfrac{8}{2}\Big) \\[1em] = (-1, 4).$

Let mid-point of BD be F.

Substituting value we get,

$F = \Big[\dfrac{1 + (-3)}{2}, \dfrac{0 + 8}{2}\Big] \\[1em] = \Big(-\dfrac{2}{2}, \dfrac{8}{2}\Big) \\[1em] = (-1, 4).$

Thus, the co-ordinates of the mid-points of AC and BD are same i.e., AC and BD bisect each other.

∴ ABCD is a parallelogram.

Hence, the co-ordinates of the mid-points of AC = (-1, 4) and BD = (-1, 4) and ABCD is a parallelogram.